
AllQuestion and Answers: Page 1595
Question Number 48725 Answers: 1 Comments: 3
Question Number 48724 Answers: 1 Comments: 1
Question Number 48720 Answers: 0 Comments: 2
Question Number 48719 Answers: 1 Comments: 1
Question Number 48718 Answers: 1 Comments: 2
Question Number 48717 Answers: 0 Comments: 1
Question Number 48715 Answers: 0 Comments: 4
Question Number 48711 Answers: 1 Comments: 1

Question Number 48703 Answers: 1 Comments: 0

Question Number 48687 Answers: 0 Comments: 0
Question Number 48705 Answers: 3 Comments: 2
Question Number 48678 Answers: 0 Comments: 0

Question Number 48677 Answers: 1 Comments: 0

Question Number 48676 Answers: 1 Comments: 0
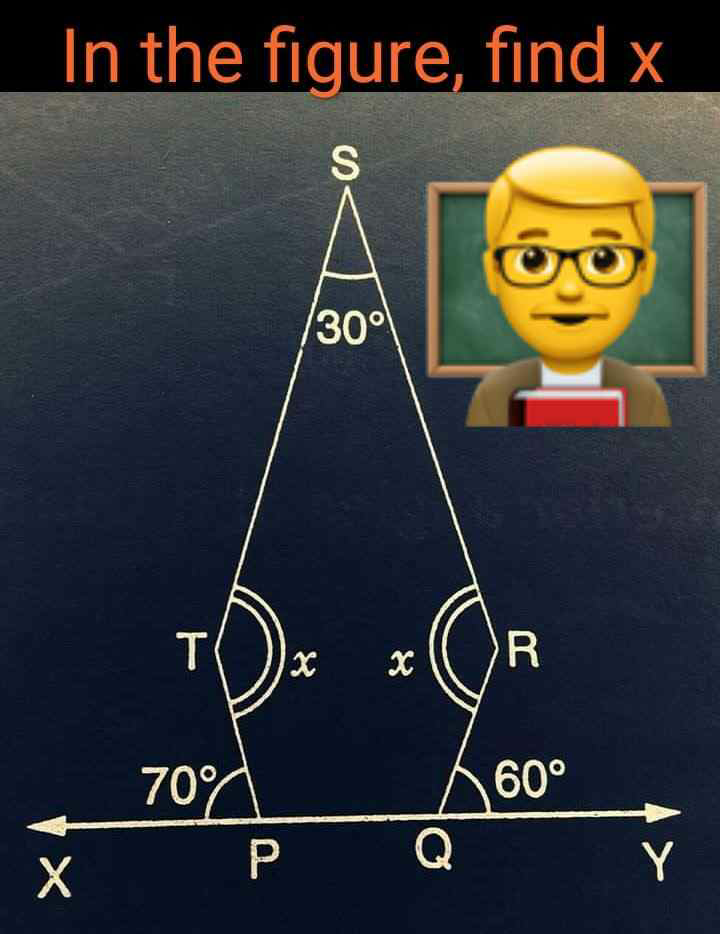
Question Number 48675 Answers: 2 Comments: 0
Question Number 48668 Answers: 1 Comments: 3
Question Number 48667 Answers: 1 Comments: 1
Question Number 48664 Answers: 0 Comments: 3
Question Number 48659 Answers: 1 Comments: 0
Question Number 48656 Answers: 0 Comments: 3

Question Number 48653 Answers: 1 Comments: 1

Question Number 48643 Answers: 1 Comments: 2

Question Number 48638 Answers: 1 Comments: 4

Question Number 48616 Answers: 0 Comments: 1
Question Number 48611 Answers: 1 Comments: 0

Question Number 48610 Answers: 0 Comments: 0

Pg 1590 Pg 1591 Pg 1592 Pg 1593 Pg 1594 Pg 1595 Pg 1596 Pg 1597 Pg 1598 Pg 1599
