
AllQuestion and Answers: Page 1583
Question Number 50330 Answers: 1 Comments: 1

Question Number 50299 Answers: 1 Comments: 7
Question Number 50293 Answers: 2 Comments: 4
Question Number 50279 Answers: 2 Comments: 0
Question Number 50278 Answers: 1 Comments: 6
Question Number 50275 Answers: 1 Comments: 0

Question Number 50274 Answers: 2 Comments: 0

Question Number 50258 Answers: 1 Comments: 0
Question Number 50257 Answers: 1 Comments: 0

Question Number 50248 Answers: 1 Comments: 0

Question Number 50228 Answers: 0 Comments: 3

Question Number 50226 Answers: 0 Comments: 1
Question Number 50220 Answers: 0 Comments: 4
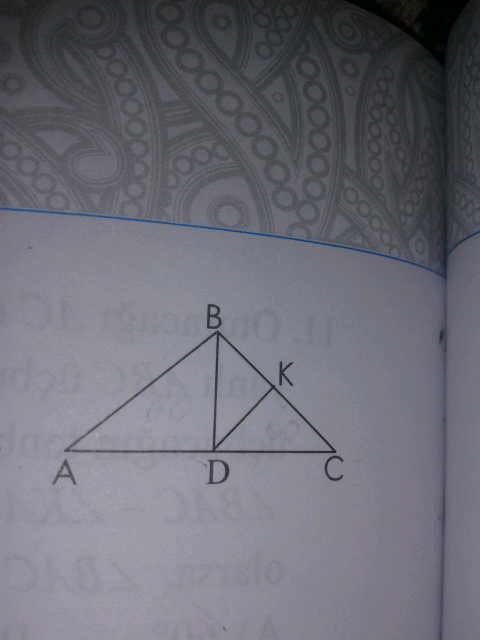
Question Number 50219 Answers: 7 Comments: 0

Question Number 50423 Answers: 1 Comments: 1
Question Number 50209 Answers: 1 Comments: 0
Question Number 50201 Answers: 0 Comments: 2
$$\mathrm{a}=\mathrm{665},\mathrm{21}\:\:\:\:\:\:\:\mathrm{b}=\mathrm{47},\mathrm{14} \\ $$
Question Number 50198 Answers: 0 Comments: 1
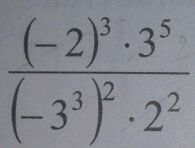
Question Number 50197 Answers: 0 Comments: 0
$$\mathrm{plz}\:\mathrm{plz}\:\mathrm{help}\:\mathrm{me}\:\mathrm{sir} \\ $$
Question Number 50196 Answers: 0 Comments: 0
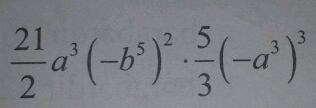
Question Number 50195 Answers: 0 Comments: 0

Question Number 50194 Answers: 0 Comments: 2

Question Number 50349 Answers: 0 Comments: 0

Question Number 50200 Answers: 0 Comments: 2
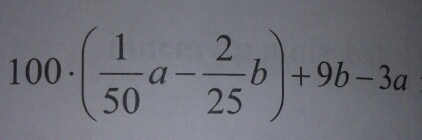
Question Number 50239 Answers: 1 Comments: 1

Question Number 50186 Answers: 1 Comments: 0
Pg 1578 Pg 1579 Pg 1580 Pg 1581 Pg 1582 Pg 1583 Pg 1584 Pg 1585 Pg 1586 Pg 1587
