
AllQuestion and Answers: Page 1570
Question Number 51849 Answers: 3 Comments: 3
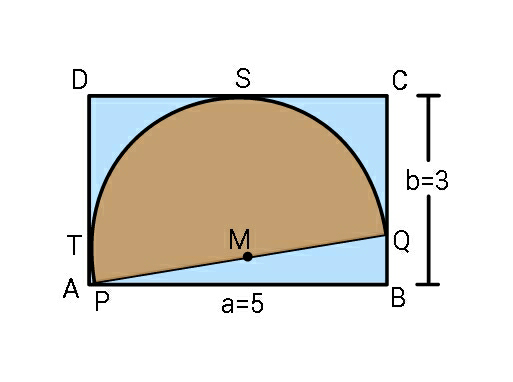
Question Number 51847 Answers: 0 Comments: 1

Question Number 51843 Answers: 1 Comments: 3
Question Number 51841 Answers: 0 Comments: 2

Question Number 51840 Answers: 2 Comments: 0
Question Number 51837 Answers: 1 Comments: 1

Question Number 51834 Answers: 0 Comments: 1
Question Number 51825 Answers: 1 Comments: 3
Question Number 51824 Answers: 1 Comments: 0
Question Number 51818 Answers: 0 Comments: 0
Question Number 51817 Answers: 0 Comments: 0
Question Number 51812 Answers: 0 Comments: 4

Question Number 51805 Answers: 2 Comments: 3
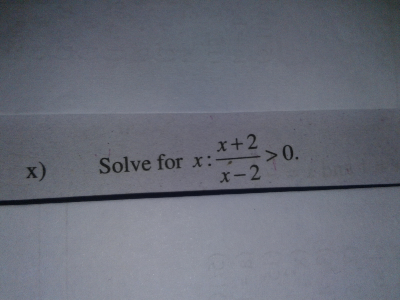
Question Number 51798 Answers: 0 Comments: 3

Question Number 51779 Answers: 0 Comments: 3
Question Number 51774 Answers: 1 Comments: 4

Question Number 51768 Answers: 1 Comments: 2

Question Number 51772 Answers: 2 Comments: 0
Question Number 51733 Answers: 2 Comments: 3

Question Number 51729 Answers: 1 Comments: 0
Question Number 51728 Answers: 0 Comments: 5
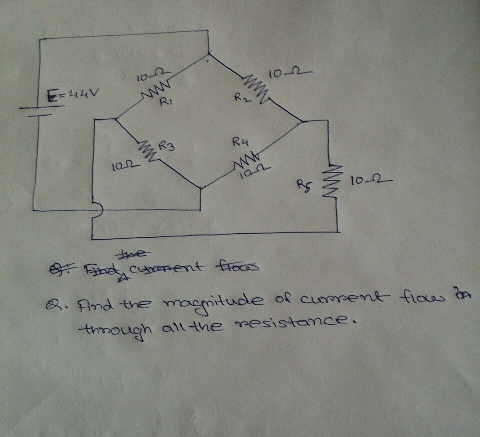
Question Number 51721 Answers: 1 Comments: 0
Question Number 51720 Answers: 0 Comments: 0

Question Number 51712 Answers: 0 Comments: 9
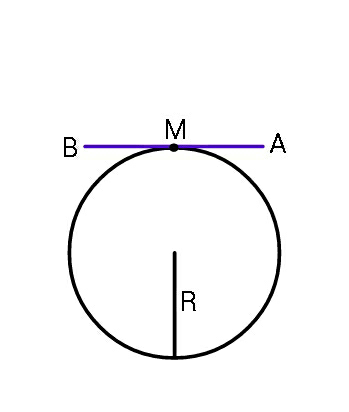
Question Number 51700 Answers: 1 Comments: 1
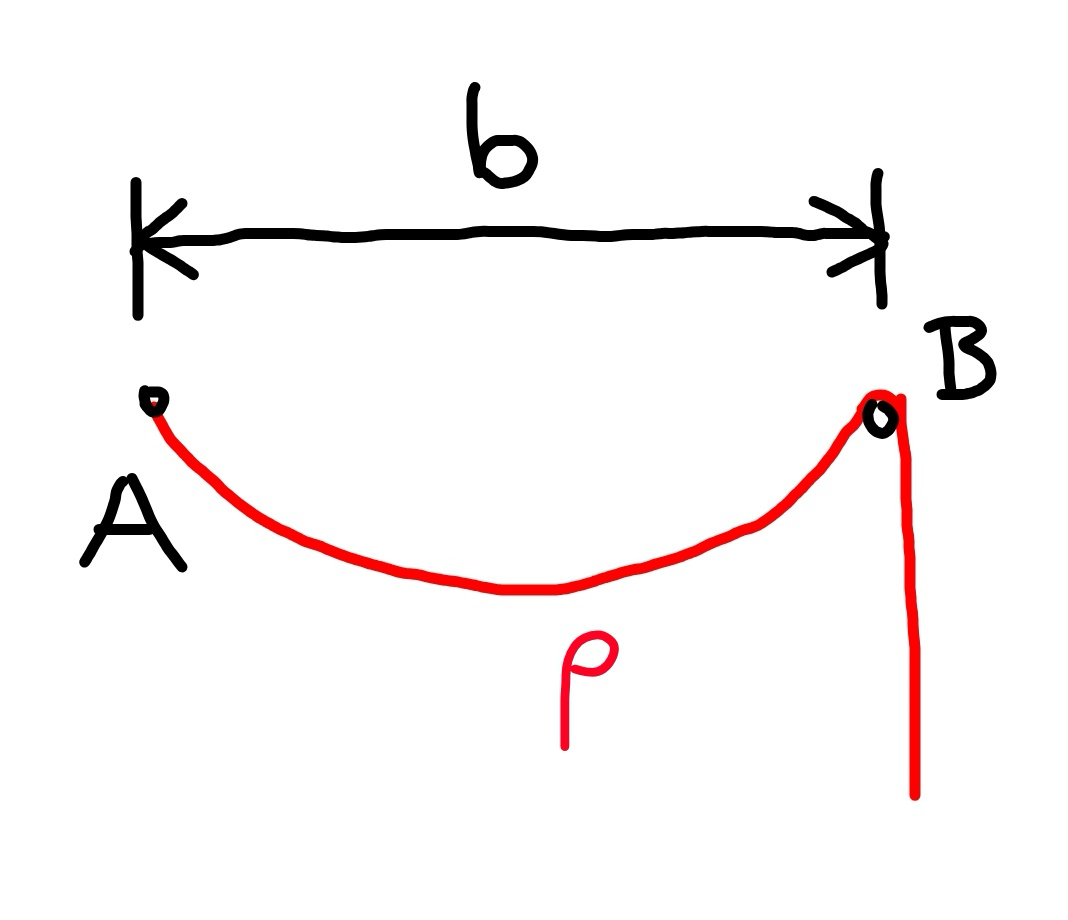
Question Number 51694 Answers: 1 Comments: 0

Pg 1565 Pg 1566 Pg 1567 Pg 1568 Pg 1569 Pg 1570 Pg 1571 Pg 1572 Pg 1573 Pg 1574
