
AllQuestion and Answers: Page 1569
Question Number 51992 Answers: 0 Comments: 0
Question Number 51991 Answers: 0 Comments: 1
Question Number 51990 Answers: 1 Comments: 0
Question Number 51989 Answers: 1 Comments: 0
Question Number 51988 Answers: 0 Comments: 0
Question Number 51987 Answers: 1 Comments: 1
Question Number 51986 Answers: 0 Comments: 0
Question Number 51985 Answers: 0 Comments: 1
Question Number 51984 Answers: 0 Comments: 0
Question Number 51983 Answers: 0 Comments: 0
Question Number 51982 Answers: 0 Comments: 0
Question Number 51981 Answers: 0 Comments: 0
Question Number 51980 Answers: 0 Comments: 0
Question Number 51973 Answers: 0 Comments: 1
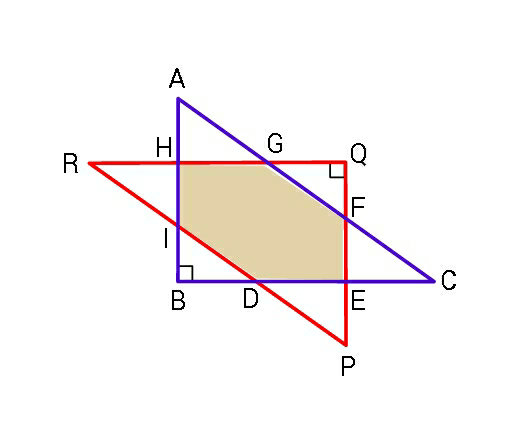
Question Number 51959 Answers: 0 Comments: 4

Question Number 51950 Answers: 2 Comments: 0
Question Number 51942 Answers: 1 Comments: 5

Question Number 51938 Answers: 0 Comments: 2
Question Number 51933 Answers: 2 Comments: 1
Question Number 51922 Answers: 1 Comments: 0
Question Number 51921 Answers: 1 Comments: 0
Question Number 51911 Answers: 0 Comments: 2

Question Number 51910 Answers: 0 Comments: 4
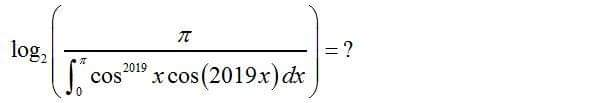
Question Number 51907 Answers: 0 Comments: 2

Question Number 51905 Answers: 2 Comments: 0
Question Number 51901 Answers: 0 Comments: 0
Pg 1564 Pg 1565 Pg 1566 Pg 1567 Pg 1568 Pg 1569 Pg 1570 Pg 1571 Pg 1572 Pg 1573
