Question and Answers Forum

AllQuestion and Answers: Page 1566

Question Number 52161 Answers: 2 Comments: 7

Question Number 52129 Answers: 1 Comments: 5

Question Number 52124 Answers: 1 Comments: 0

The curve y = ax + (b/(2x− 1)) has the stationary point at (2, 7) . Find the value of a and b .

$$\mathrm{The}\:\mathrm{curve}\:\mathrm{y}\:=\:\mathrm{ax}\:+\:\frac{\mathrm{b}}{\mathrm{2x}−\:\mathrm{1}}\:\mathrm{has}\:\mathrm{the}\:\mathrm{stationary}\:\mathrm{point}\:\mathrm{at}\:\:\left(\mathrm{2},\:\mathrm{7}\right)\:.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{a}\:\mathrm{and}\:\mathrm{b}\:. \\ $$

Question Number 52123 Answers: 1 Comments: 1

Find lim_(x→∞) ((sin x)/x).

$$\mathrm{Find}\:\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}. \\ $$

Question Number 52108 Answers: 1 Comments: 0

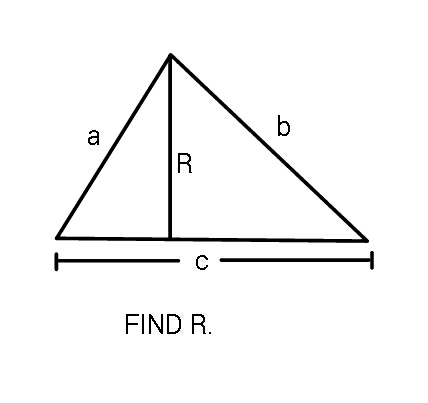
Question Number 52103 Answers: 2 Comments: 0

((×−0.1)/(×+0.1))=((1.2)/(1.7)) Sir plz help me

$$\frac{×−\mathrm{0}.\mathrm{1}}{×+\mathrm{0}.\mathrm{1}}=\frac{\mathrm{1}.\mathrm{2}}{\mathrm{1}.\mathrm{7}}\:\:\:\:\:\:\:\:\:\:\mathrm{Sir}\:\mathrm{plz}\:\mathrm{help}\:\mathrm{me} \\ $$

Question Number 52099 Answers: 1 Comments: 1

Question Number 52091 Answers: 2 Comments: 1

3^x +4^x =5^x find x BY ISHOLA

$$\mathrm{3}^{{x}} +\mathrm{4}^{{x}} =\mathrm{5}^{{x}} \\ $$$${find}\:{x} \\ $$$${BY}\:{ISHOLA} \\ $$

Question Number 52089 Answers: 0 Comments: 0

Question Number 52088 Answers: 0 Comments: 0

determine the value of 5e^(0.5 ) correct to 5 significant fig using d power series of e^x

$${determine}\:{the}\:{value}\:{of}\:\mathrm{5}{e}^{\mathrm{0}.\mathrm{5}\:} \:{correct}\:{to}\:\mathrm{5}\:{significant}\:{fig}\:{using}\:{d}\:{power}\:{series}\:{of}\:{e}^{{x}} \\ $$

Question Number 52087 Answers: 1 Comments: 1

If 1,a_1 ,a_2 ,...,a_(n−1) are n^(th) roots of unity the find the value of (1/(1+1))+(1/(1+a_1 ))+(1/(1+a_2 ))+..+(1/(1+a_(n−1) )) n is odd number

$$\mathrm{If}\:\mathrm{1},{a}_{\mathrm{1}} ,{a}_{\mathrm{2}} ,...,{a}_{{n}−\mathrm{1}} \:\mathrm{are}\:{n}^{{th}} \:\mathrm{roots}\:\mathrm{of} \\ $$$$\mathrm{unity}\:\mathrm{the}\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of} \\ $$$$\frac{\mathrm{1}}{\mathrm{1}+\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{1}+{a}_{\mathrm{1}} }+\frac{\mathrm{1}}{\mathrm{1}+{a}_{\mathrm{2}} }+..+\frac{\mathrm{1}}{\mathrm{1}+{a}_{{n}−\mathrm{1}} } \\ $$$${n}\:\mathrm{is}\:\mathrm{odd}\:\mathrm{number} \\ $$

Question Number 52086 Answers: 1 Comments: 3

If 1,a_(1,) a_2 ,...,a_(n−1) are n^(th) roots of unity, then prove that. (1+a_1 )(1+a_2 )..(1+a_(n−1) )= n if n is even 0 if n is odd

$$\mathrm{If}\:\mathrm{1},{a}_{\mathrm{1},} {a}_{\mathrm{2}} ,...,{a}_{{n}−\mathrm{1}} \:\mathrm{are}\:{n}^{{th}} \:\mathrm{roots}\:\mathrm{of} \\ $$$$\mathrm{unity},\:\mathrm{then}\:\mathrm{prove}\:\mathrm{that}. \\ $$$$\left(\mathrm{1}+{a}_{\mathrm{1}} \right)\left(\mathrm{1}+{a}_{\mathrm{2}} \right)..\left(\mathrm{1}+{a}_{{n}−\mathrm{1}} \right)= \\ $$$${n}\:\:\mathrm{if}\:{n}\:\mathrm{is}\:\mathrm{even} \\ $$$$\mathrm{0}\:\mathrm{if}\:{n}\:\mathrm{is}\:\mathrm{odd} \\ $$

Question Number 52082 Answers: 1 Comments: 1

Question Number 52079 Answers: 1 Comments: 1

Sum to the n terms of the series whose n^(th ) term is 2^(n−1 ) + 8n^3 −6n^2

$${Sum}\:{to}\:{the}\:{n}\:{terms}\:{of}\:{the}\:{series}\:{whose}\:{n}^{{th}\:} \:{term}\:{is}\:\mathrm{2}^{{n}−\mathrm{1}\:} \:+\:\mathrm{8}{n}^{\mathrm{3}} \:−\mathrm{6}{n}^{\mathrm{2}} \\ $$

Question Number 52075 Answers: 0 Comments: 0

Question Number 52072 Answers: 0 Comments: 0

Question Number 52061 Answers: 2 Comments: 3

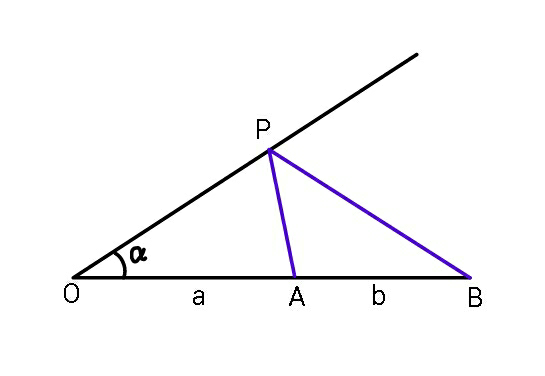
Question Number 52059 Answers: 0 Comments: 1

Question Number 52058 Answers: 1 Comments: 0

Question Number 52052 Answers: 0 Comments: 1

Question Number 52038 Answers: 1 Comments: 0

(6x+8)+3=(8x−5)−6 sir plz help me

$$\left(\mathrm{6x}+\mathrm{8}\right)+\mathrm{3}=\left(\mathrm{8x}−\mathrm{5}\right)−\mathrm{6}\:\:\: \\ $$$$\mathrm{sir}\:\mathrm{plz}\:\mathrm{help}\:\mathrm{me} \\ $$

Question Number 52034 Answers: 1 Comments: 1

Question Number 52043 Answers: 1 Comments: 1

Question Number 52031 Answers: 2 Comments: 1

Question Number 52030 Answers: 1 Comments: 0

given that log2=0.3010 log3=0.477 log5=0.699 find the values of log(√((0.2)))

$${given}\:{that}\:{log}\mathrm{2}=\mathrm{0}.\mathrm{3010}\:{log}\mathrm{3}=\mathrm{0}.\mathrm{477}\:{log}\mathrm{5}=\mathrm{0}.\mathrm{699} \\ $$$${find}\:{the}\:{values}\:{of}\:{log}\sqrt{\left(\mathrm{0}.\mathrm{2}\right)} \\ $$$$ \\ $$

Question Number 52449 Answers: 0 Comments: 0

let j=e^((i2π)/3) and P(x)=(1+jx)^n −(1−jx)^n with n integr natural 1) find roots of P(x) 2)factorize P(x) inside C[x] 3) calculate ∫_0 ^1 P(x)dx. 4) decompose inside C(x) the fraction F(x)=(1/(P(x)))

$${let}\:{j}={e}^{\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} \:\:\:{and}\:{P}\left({x}\right)=\left(\mathrm{1}+{jx}\right)^{{n}} −\left(\mathrm{1}−{jx}\right)^{{n}} \:\:{with}\:{n}\:{integr}\:{natural} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{roots}\:{of}\:{P}\left({x}\right) \\ $$$$\left.\mathrm{2}\right){factorize}\:{P}\left({x}\right)\:{inside}\:{C}\left[{x}\right] \\ $$$$\left.\mathrm{3}\right)\:\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} {P}\left({x}\right){dx}. \\ $$$$\left.\mathrm{4}\right)\:{decompose}\:{inside}\:{C}\left({x}\right)\:{the}\:{fraction}\:{F}\left({x}\right)=\frac{\mathrm{1}}{{P}\left({x}\right)} \\ $$

Pg 1561 Pg 1562 Pg 1563 Pg 1564 Pg 1565 Pg 1566 Pg 1567 Pg 1568 Pg 1569 Pg 1570

Contact: info@tinkutara.com