Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1562

Question Number 52947 Answers: 1 Comments: 0

∫_( 0) ^π ((x tan x)/(sec x+cos x)) dx =

$$\underset{\:\mathrm{0}} {\overset{\pi} {\int}}\:\:\frac{{x}\:\mathrm{tan}\:{x}}{\mathrm{sec}\:{x}+\mathrm{cos}\:{x}}\:{dx}\:= \\ $$

Question Number 52944 Answers: 1 Comments: 0

∫_( 0) ^( 1) ((x^3 − 1)/((1 + x^2 ) ln x)) dx

$$\int_{\:\mathrm{0}} ^{\:\mathrm{1}} \:\:\frac{\boldsymbol{\mathrm{x}}^{\mathrm{3}} \:−\:\mathrm{1}}{\left(\mathrm{1}\:+\:\boldsymbol{\mathrm{x}}^{\mathrm{2}} \right)\:\boldsymbol{\mathrm{ln}}\:\boldsymbol{\mathrm{x}}}\:\:\boldsymbol{\mathrm{dx}} \\ $$

Question Number 52938 Answers: 0 Comments: 3

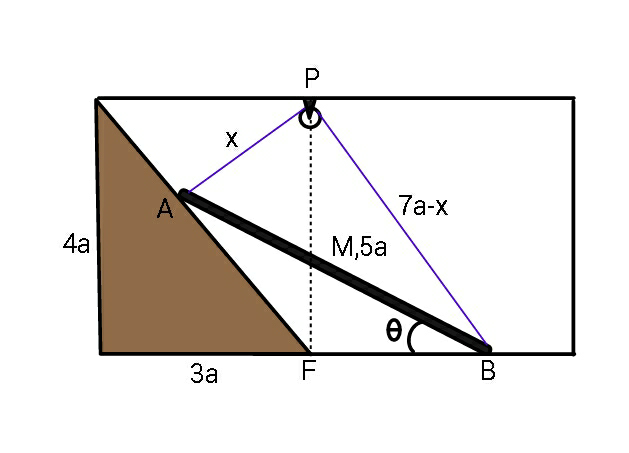
Question Number 52936 Answers: 0 Comments: 1

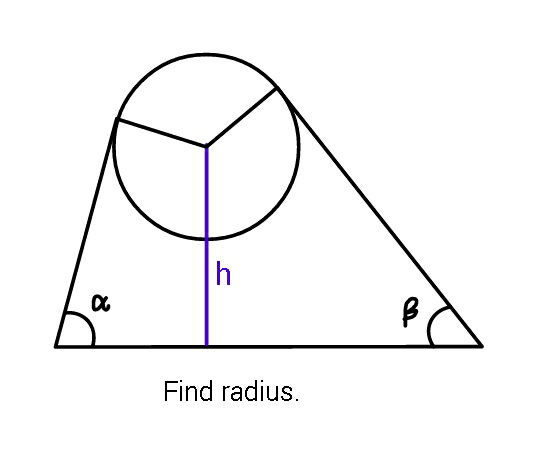
Question Number 52946 Answers: 1 Comments: 1

∫_( 0) ^(π/2) log sin 2x dx =

$$\underset{\:\mathrm{0}} {\overset{\pi/\mathrm{2}} {\int}}\:\:\mathrm{log}\:\mathrm{sin}\:\mathrm{2}{x}\:{dx}\:= \\ $$

Question Number 52945 Answers: 3 Comments: 2

Question Number 52911 Answers: 2 Comments: 2

Question Number 52898 Answers: 1 Comments: 0

∫arcsin x arccos x dx=?

$$\int\mathrm{arcsin}\:{x}\:\mathrm{arccos}\:{x}\:{dx}=? \\ $$

Question Number 52900 Answers: 3 Comments: 0

∫_0 ^(π/2) sin x (√(sin 2x)) dx=? ∫_(−(π/4)) ^(π/4) cos x (√(cos 2x)) dx=?

$$\underset{\mathrm{0}} {\overset{\frac{\pi}{\mathrm{2}}} {\int}}\mathrm{sin}\:{x}\:\sqrt{\mathrm{sin}\:\mathrm{2}{x}}\:{dx}=? \\ $$$$\underset{−\frac{\pi}{\mathrm{4}}} {\overset{\frac{\pi}{\mathrm{4}}} {\int}}\mathrm{cos}\:{x}\:\sqrt{\mathrm{cos}\:\mathrm{2}{x}}\:{dx}=? \\ $$

Question Number 52867 Answers: 1 Comments: 0

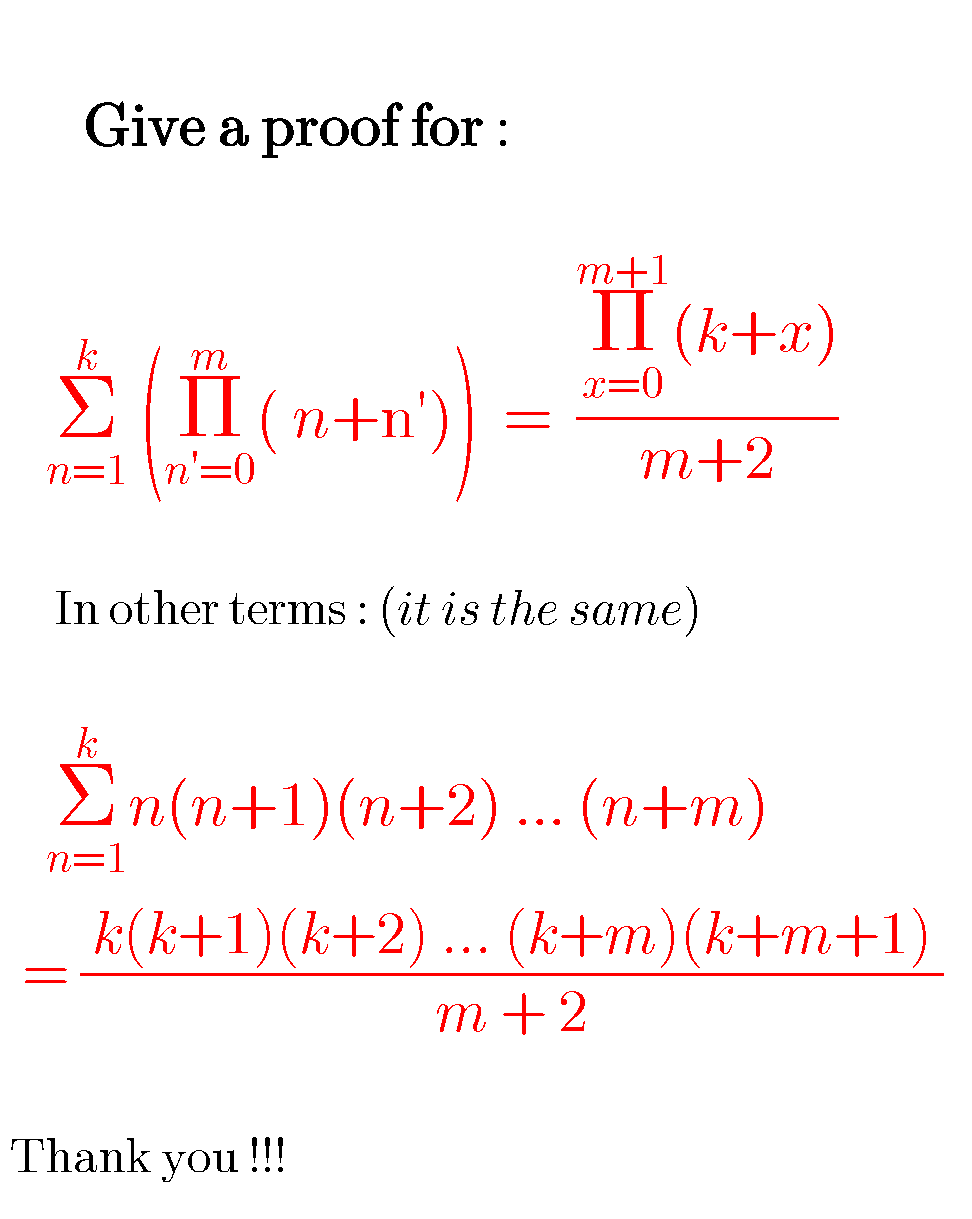
Question Number 52859 Answers: 0 Comments: 3

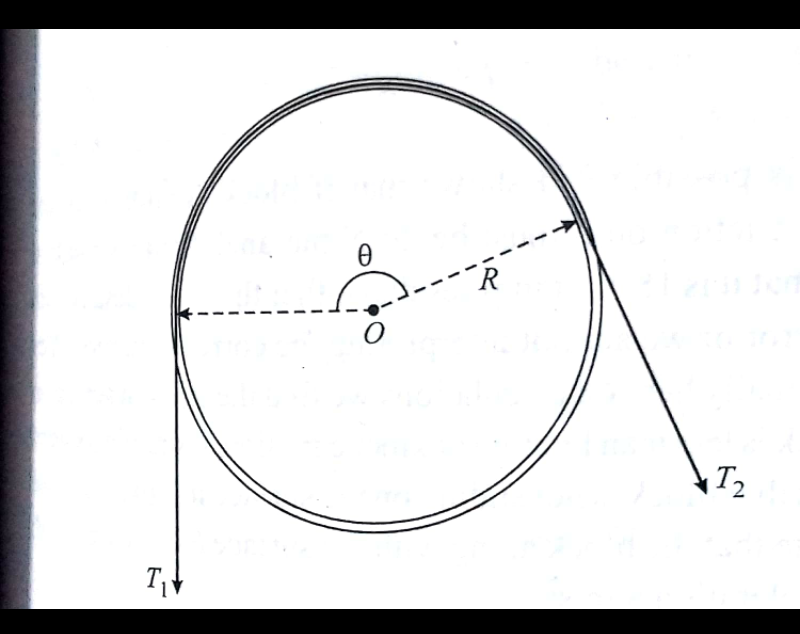
Question Number 52847 Answers: 0 Comments: 2

Question Number 52846 Answers: 1 Comments: 0

Question Number 52845 Answers: 1 Comments: 0

Question Number 52841 Answers: 1 Comments: 4

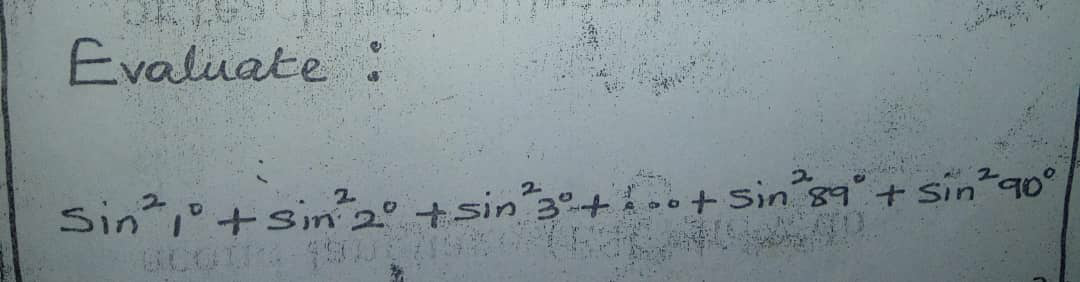
Question Number 52881 Answers: 1 Comments: 9

Question Number 52825 Answers: 1 Comments: 4

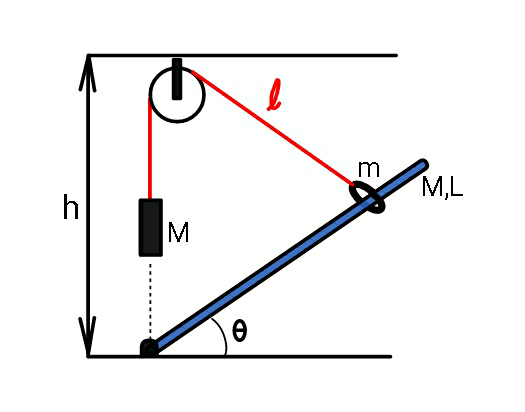
Question Number 52820 Answers: 1 Comments: 0

y = log{(√x) +(1/((√x) ))}^2 show that x(x+1)^2 y_2 + (x+1)^2 y_1 = 2

$${y}\:=\:{log}\left\{\sqrt{{x}}\:+\frac{\mathrm{1}}{\sqrt{{x}}\:\:\:}\right\}^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$${show}\:{that} \\ $$$${x}\left({x}+\mathrm{1}\right)^{\mathrm{2}} {y}_{\mathrm{2}} \:+\:\left({x}+\mathrm{1}\right)^{\mathrm{2}} {y}_{\mathrm{1}} \:=\:\mathrm{2} \\ $$

Question Number 52818 Answers: 1 Comments: 0

Question Number 52814 Answers: 0 Comments: 6

Question Number 52808 Answers: 0 Comments: 0

Question Number 52806 Answers: 1 Comments: 2

Question Number 52802 Answers: 0 Comments: 0

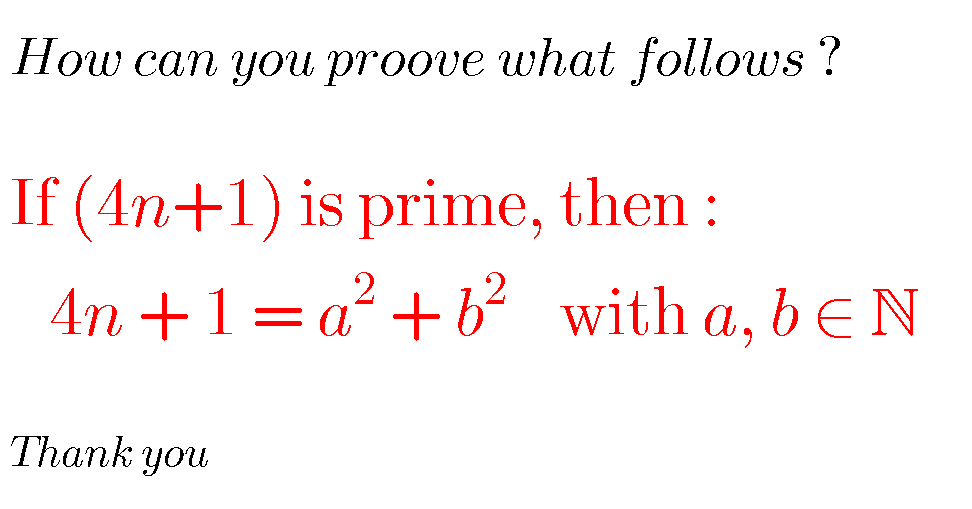
Question Number 52795 Answers: 2 Comments: 0

Question Number 52790 Answers: 1 Comments: 0

Question Number 52787 Answers: 2 Comments: 0

Pg 1557 Pg 1558 Pg 1559 Pg 1560 Pg 1561 Pg 1562 Pg 1563 Pg 1564 Pg 1565 Pg 1566

Terms of Service

Contact: info@tinkutara.com