Question and Answers Forum

AllQuestion and Answers: Page 1560

Question Number 52558 Answers: 1 Comments: 6

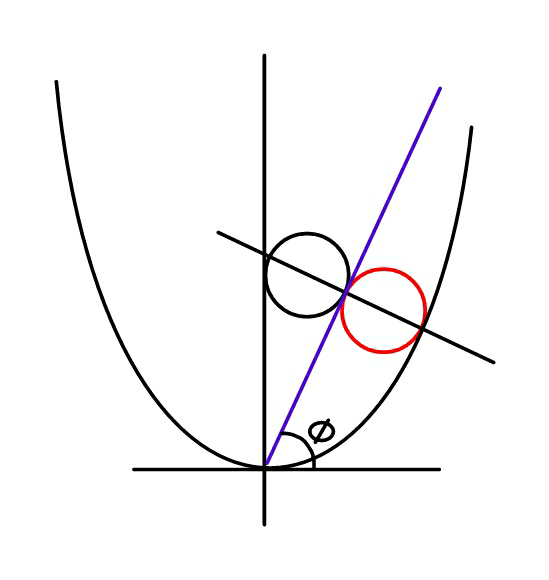
Question Number 52550 Answers: 1 Comments: 1

∫_0 ^( ∞) (x/(e^x − 1)) dx

$$\:\:\int_{\mathrm{0}} ^{\:\infty} \:\:\:\frac{\mathrm{x}}{\mathrm{e}^{\mathrm{x}} \:−\:\mathrm{1}}\:\:\mathrm{dx}\: \\ $$

Question Number 52542 Answers: 1 Comments: 3

Let f(x) = (( 5x + 40 )/(x + 2)) (1) Calculate the derivative of f(4) without using the answer of question (2), (2) Calculate the derivative of f(x), (3) Calculate lim_(x → +∞) f(x) . All your answers should be correctly proved and detailed. Can you please help me for that exercise.

$$\mathrm{Let}\:{f}\left({x}\right)\:=\:\frac{\:\mathrm{5}{x}\:+\:\mathrm{40}\:}{{x}\:+\:\mathrm{2}} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{Calculate}\:\mathrm{the}\:\mathrm{derivative}\:\mathrm{of}\:{f}\left(\mathrm{4}\right)\:\mathrm{without} \\ $$$$\mathrm{using}\:\mathrm{the}\:\mathrm{answer}\:\mathrm{of}\:\mathrm{question}\:\left(\mathrm{2}\right), \\ $$$$\left(\mathrm{2}\right)\:\mathrm{Calculate}\:\mathrm{the}\:\mathrm{derivative}\:\mathrm{of}\:{f}\left({x}\right), \\ $$$$\left(\mathrm{3}\right)\:\mathrm{Calculate}\:\:\underset{{x}\:\rightarrow\:+\infty} {\mathrm{lim}}\:{f}\left({x}\right)\:. \\ $$$$\mathrm{All}\:\mathrm{your}\:\mathrm{answers}\:\mathrm{should}\:\mathrm{be}\:\mathrm{correctly}\:\mathrm{proved}\:\mathrm{and}\:\mathrm{detailed}. \\ $$$$ \\ $$$${Can}\:{you}\:{please}\:{help}\:{me}\:{for}\:{that}\:{exercise}. \\ $$

Question Number 52539 Answers: 2 Comments: 0

Question Number 52533 Answers: 0 Comments: 5

Question Number 52523 Answers: 0 Comments: 12

Question Number 52578 Answers: 0 Comments: 0

Question Number 52520 Answers: 0 Comments: 0

Please help John travels a distance of 24km from twon A on a bearing of 060° to town B. He then travels a distance of 18km to town C, which is 30km east of town A. (i) what is the bearing of town C from town B? (ii) calculate the bearing of town A from town C?

$$\mathrm{Please}\:\mathrm{he}{lp} \\ $$$$\:\mathrm{John}\:\mathrm{travels}\:\mathrm{a}\:\mathrm{distance}\:\mathrm{of}\:\mathrm{24km} \\ $$$$\mathrm{from}\:\mathrm{twon}\:\mathrm{A}\:\mathrm{on}\:\mathrm{a}\:\mathrm{bearing}\:\mathrm{of}\:\mathrm{060}°\:\mathrm{to} \\ $$$$\mathrm{town}\:\mathrm{B}.\:\mathrm{He}\:\mathrm{then}\:\mathrm{travels}\:\mathrm{a}\:\mathrm{distance} \\ $$$$\mathrm{of}\:\mathrm{18km}\:\mathrm{to}\:\mathrm{town}\:\mathrm{C},\:\mathrm{which}\:\mathrm{is}\:\mathrm{30km} \\ $$$$\mathrm{east}\:\mathrm{of}\:\mathrm{town}\:\mathrm{A}. \\ $$$$\left(\mathrm{i}\right)\:\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{bearing}\:\mathrm{of}\:\mathrm{town}\:\mathrm{C}\:\mathrm{from} \\ $$$$\:\:\:\:\:\:\:\mathrm{town}\:\mathrm{B}? \\ $$$$\left(\mathrm{ii}\right)\:\mathrm{calculate}\:\mathrm{the}\:\mathrm{bearing}\:\mathrm{of}\:\mathrm{town}\:\mathrm{A}\: \\ $$$$\:\:\:\:\:\:\:\:\mathrm{from}\:\mathrm{town}\:\mathrm{C}? \\ $$

Question Number 52516 Answers: 1 Comments: 0

find the value tanθ+2tan2θ+2^2 tan2^2 θ+2^3 tan2^3 θ+..+2^(n−1) tan2^(n−1) θ

$${find}\:{the}\:{value} \\ $$$${tan}\theta+\mathrm{2}{tan}\mathrm{2}\theta+\mathrm{2}^{\mathrm{2}} {tan}\mathrm{2}^{\mathrm{2}} \theta+\mathrm{2}^{\mathrm{3}} {tan}\mathrm{2}^{\mathrm{3}} \theta+..+\mathrm{2}^{{n}−\mathrm{1}} {tan}\mathrm{2}^{{n}−\mathrm{1}} \theta \\ $$

Question Number 52515 Answers: 2 Comments: 3

1)∫_0 ^∞ ((tan^(−1) (ax)−tan^(−1) (bx))/x)dx 2)∫_0 ^∞ ((e^(−x) sinx)/x)dx

$$\left.\mathrm{1}\right)\int_{\mathrm{0}} ^{\infty} \frac{{tan}^{−\mathrm{1}} \left({ax}\right)−{tan}^{−\mathrm{1}} \left({bx}\right)}{{x}}{dx} \\ $$$$\left.\mathrm{2}\right)\int_{\mathrm{0}} ^{\infty} \frac{{e}^{−{x}} {sinx}}{{x}}{dx} \\ $$

Question Number 52506 Answers: 1 Comments: 1

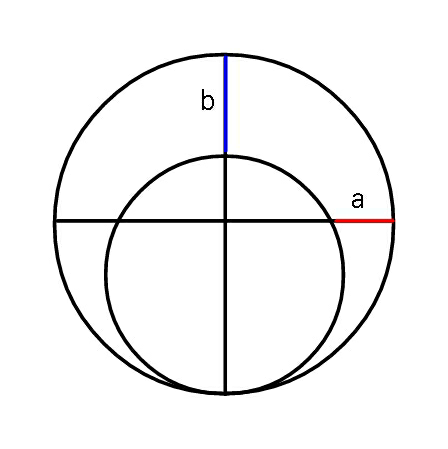
Question Number 52499 Answers: 0 Comments: 0

Let f is a 2^(nd) degree polynomial. (1/(2πi)) ∫_(∣z∣=2) ((z f ′(z))/(f(z))) dz = 0, and (1/(2πi)) ∫_(∣z∣=2) ((z^2 f ′(z))/(f(z))) dz = −2 If f(0) = 2017, find explicit form of f(z)

$$\mathrm{Let}\:{f}\:\mathrm{is}\:\mathrm{a}\:\mathrm{2}^{\mathrm{nd}} \:\mathrm{degree}\:\mathrm{polynomial}. \\ $$$$\frac{\mathrm{1}}{\mathrm{2}\pi{i}}\:\int_{\mid{z}\mid=\mathrm{2}} \frac{{z}\:{f}\:'\left({z}\right)}{{f}\left({z}\right)}\:{dz}\:=\:\mathrm{0},\:\:\mathrm{and}\:\frac{\mathrm{1}}{\mathrm{2}\pi{i}}\:\int_{\mid{z}\mid=\mathrm{2}} \frac{{z}^{\mathrm{2}} \:{f}\:'\left({z}\right)}{{f}\left({z}\right)}\:{dz}\:=\:−\mathrm{2} \\ $$$$\mathrm{If}\:{f}\left(\mathrm{0}\right)\:=\:\mathrm{2017},\:\mathrm{find}\:\mathrm{explicit}\:\mathrm{form}\:\mathrm{of}\:{f}\left({z}\right) \\ $$

Question Number 52498 Answers: 1 Comments: 1

Let z is complex number and satisfy z^(2017) = 1, z ≠ 1 Find (1 + z)(1 + z^2 )(1 + z^3 )…(1 + z^(2016) )

$$\mathrm{Let}\:{z}\:\mathrm{is}\:\mathrm{complex}\:\mathrm{number}\:\mathrm{and}\:\mathrm{satisfy}\:{z}^{\mathrm{2017}} \:=\:\mathrm{1},\:\:{z}\:\neq\:\mathrm{1} \\ $$$$\mathrm{Find}\:\left(\mathrm{1}\:+\:{z}\right)\left(\mathrm{1}\:+\:{z}^{\mathrm{2}} \right)\left(\mathrm{1}\:+\:{z}^{\mathrm{3}} \right)\ldots\left(\mathrm{1}\:+\:{z}^{\mathrm{2016}} \right) \\ $$

Question Number 52496 Answers: 2 Comments: 0

How does Evaporation occur and why does it cause Cooling

$${How}\:{does}\:{Evaporation} \\ $$$${occur}\:{and}\:{why}\:{does}\:{it}\: \\ $$$${cause}\:{Cooling} \\ $$

Question Number 52490 Answers: 2 Comments: 1

Question Number 52487 Answers: 0 Comments: 13

Question Number 52484 Answers: 2 Comments: 0

∫ ((cos x − x sin x)/(x cos x)) dx

$$\int\:\:\frac{\mathrm{cos}\:\mathrm{x}\:−\:\mathrm{x}\:\mathrm{sin}\:\mathrm{x}}{\mathrm{x}\:\mathrm{cos}\:\mathrm{x}}\:\:\mathrm{dx} \\ $$

Question Number 52482 Answers: 0 Comments: 2

find the value or ∫_0 ^∞ ((arctan(x^2 ))/(1+x^4 ))dx .

$${find}\:{the}\:{value}\:{or}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{arctan}\left({x}^{\mathrm{2}} \right)}{\mathrm{1}+{x}^{\mathrm{4}} }{dx}\:. \\ $$

Question Number 52470 Answers: 1 Comments: 1

Question Number 52459 Answers: 0 Comments: 2

let f(α)=∫_0 ^1 ((ln(1+iαx))/(1+x^2 ))dx 1)determine a explicit form of f(α) 2) calculate ∫_0 ^1 ((ln(1+ix))/(1+x^2 ))dx and ∫_0 ^1 ((ln(1+2ix))/(1+x^2 ))dx.

$${let}\:{f}\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{i}\alpha{x}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx} \\ $$$$\left.\mathrm{1}\right){determine}\:{a}\:{explicit}\:{form}\:{of}\:{f}\left(\alpha\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+{ix}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}\:{and}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}+\mathrm{2}{ix}\right)}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}. \\ $$

Question Number 52457 Answers: 1 Comments: 0

Two radioactive elements A and B has half lives of 100 and 50years. Samples of A and B initial contains equal numbers of atoms.What is the ratio of the remaining atoms of A to that of B after 200years?

$${Two}\:{radioactive}\:{elements}\:{A}\:{and}\:{B} \\ $$$${has}\:{half}\:{lives}\:{of}\:\mathrm{100}\:{and}\:\mathrm{50}{years}. \\ $$$${Samples}\:{of}\:{A}\:{and}\:{B}\:{initial}\:{contains} \\ $$$${equal}\:{numbers}\:{of}\:{atoms}.{What}\:{is} \\ $$$${the}\:{ratio}\:{of}\:{the}\:{remaining}\:{atoms} \\ $$$${of}\:{A}\:{to}\:{that}\:{of}\:{B}\:{after}\:\mathrm{200}{years}? \\ $$

Question Number 52450 Answers: 0 Comments: 0

let P(x)=(1+ix −x^2 )^n −1 find roots of P(x) and factorize P(x)inside C(x).

$${let}\:{P}\left({x}\right)=\left(\mathrm{1}+{ix}\:−{x}^{\mathrm{2}} \right)^{{n}} −\mathrm{1} \\ $$$${find}\:{roots}\:{of}\:\:{P}\left({x}\right)\:{and}\:{factorize}\:{P}\left({x}\right){inside}\:{C}\left({x}\right). \\ $$

Question Number 52468 Answers: 2 Comments: 4

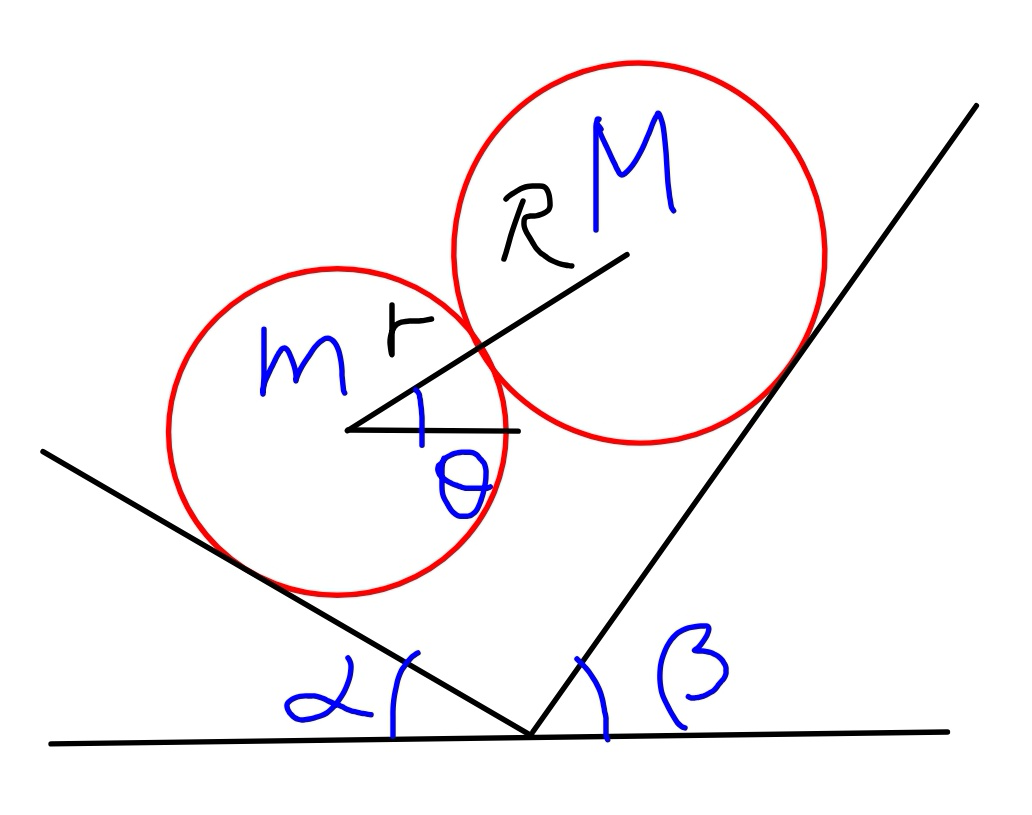
Question Number 52466 Answers: 1 Comments: 1

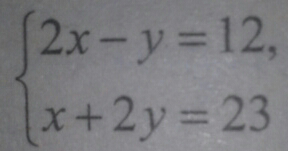
Question Number 52433 Answers: 0 Comments: 4

Question Number 52428 Answers: 0 Comments: 7

Pg 1555 Pg 1556 Pg 1557 Pg 1558 Pg 1559 Pg 1560 Pg 1561 Pg 1562 Pg 1563 Pg 1564

Contact: info@tinkutara.com