
AllQuestion and Answers: Page 1555
Question Number 53755 Answers: 1 Comments: 9
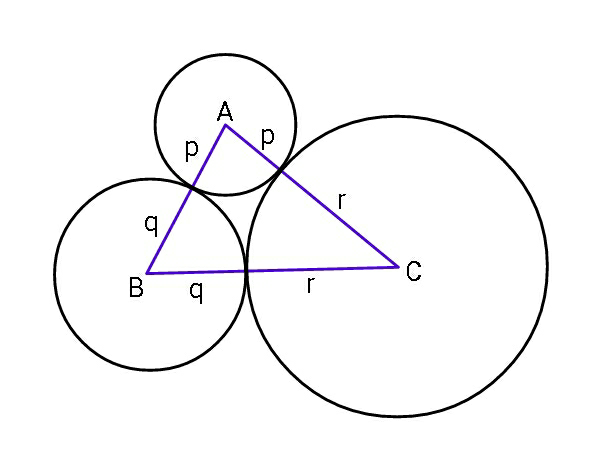
Question Number 53720 Answers: 0 Comments: 1
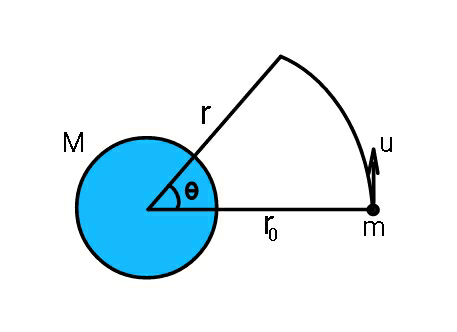
Question Number 53709 Answers: 0 Comments: 1
Question Number 53697 Answers: 0 Comments: 1
Question Number 53696 Answers: 2 Comments: 2
Question Number 53695 Answers: 1 Comments: 1
Question Number 53694 Answers: 1 Comments: 0
Question Number 53693 Answers: 1 Comments: 1
Question Number 53689 Answers: 2 Comments: 1

Question Number 53688 Answers: 2 Comments: 1
Question Number 53686 Answers: 0 Comments: 5

Question Number 53684 Answers: 1 Comments: 4

Question Number 53676 Answers: 0 Comments: 5
Question Number 53675 Answers: 2 Comments: 0
Question Number 53727 Answers: 2 Comments: 0

Question Number 53664 Answers: 1 Comments: 1

Question Number 53647 Answers: 1 Comments: 2
Question Number 53630 Answers: 1 Comments: 0
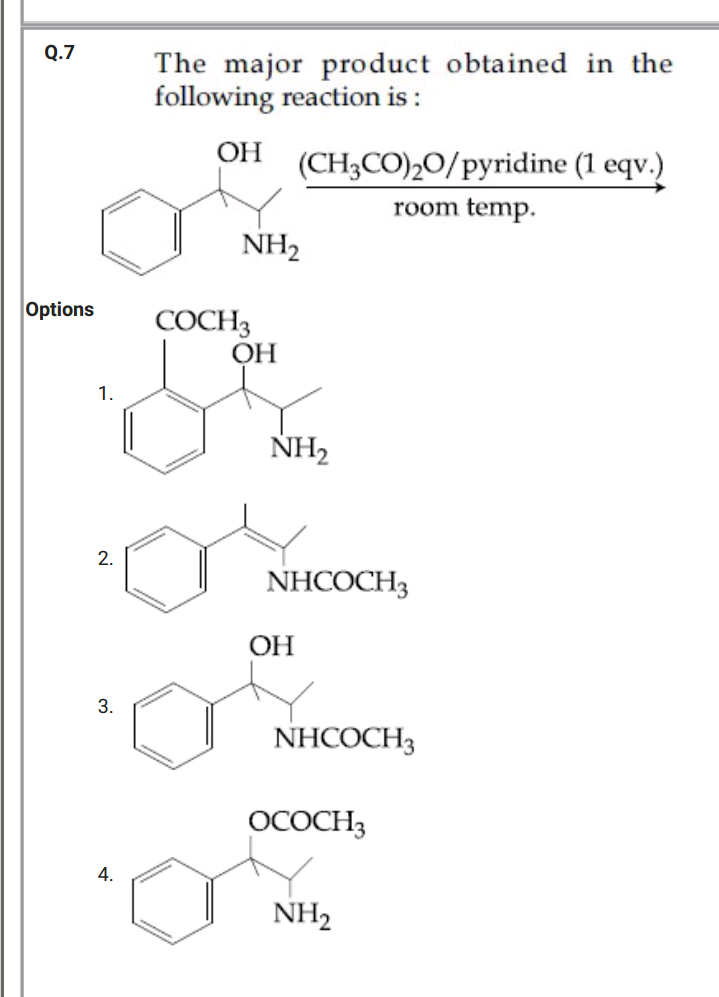
Question Number 53624 Answers: 0 Comments: 0
Question Number 53623 Answers: 1 Comments: 3
Question Number 53621 Answers: 0 Comments: 0
Question Number 53620 Answers: 1 Comments: 4
Question Number 53619 Answers: 0 Comments: 0
Question Number 53601 Answers: 1 Comments: 0
Question Number 53600 Answers: 0 Comments: 1
Question Number 53599 Answers: 0 Comments: 1
Pg 1550 Pg 1551 Pg 1552 Pg 1553 Pg 1554 Pg 1555 Pg 1556 Pg 1557 Pg 1558 Pg 1559
