Question and Answers Forum

AllQuestion and Answers: Page 1533

Question Number 55858 Answers: 0 Comments: 0

Question Number 55857 Answers: 0 Comments: 0

Let A and B are matrices in R^(2017×2017) such that A^(−1) = (A + B)^(−1) − B^(−1) and det(A^(−1) ) = 2017 Find det(B)

$$\mathrm{Let}\:{A}\:\mathrm{and}\:{B}\:\mathrm{are}\:\mathrm{matrices}\:\mathrm{in}\:\mathbb{R}^{\mathrm{2017}×\mathrm{2017}} \:\mathrm{such}\:\mathrm{that}\: \\ $$$${A}^{−\mathrm{1}} \:=\:\left({A}\:+\:{B}\right)^{−\mathrm{1}} \:−\:{B}^{−\mathrm{1}} \\ $$$$\mathrm{and}\: \\ $$$$\mathrm{det}\left({A}^{−\mathrm{1}} \right)\:=\:\mathrm{2017} \\ $$$$\mathrm{Find}\:\:\mathrm{det}\left({B}\right) \\ $$

Question Number 55856 Answers: 1 Comments: 1

For what values of p the integral ∫_0 ^1 x^p ln x dx converge?

$$\mathrm{For}\:\mathrm{what}\:\mathrm{values}\:\mathrm{of}\:{p}\:\mathrm{the}\:\mathrm{integral} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{{p}} \:\mathrm{ln}\:{x}\:{dx} \\ $$$$\mathrm{converge}? \\ $$

Question Number 55855 Answers: 0 Comments: 1

How to integrate ∫_0 ^1 ((sec^2 x)/(x(√x))) dx ?

$$\mathrm{How}\:\mathrm{to}\:\mathrm{integrate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{sec}^{\mathrm{2}} \:{x}}{{x}\sqrt{{x}}}\:{dx}\:\:? \\ $$

Question Number 55853 Answers: 0 Comments: 0

Question Number 55846 Answers: 1 Comments: 0

If x, y, z are in AP. Then the value of the determinant determinant (((a+2),(a+3),(a+2x)),((a+3),(a+4),(a+2y)),((a+4),(a+5),(a+2z))) is

$$\mathrm{If}\:{x},\:{y},\:{z}\:\mathrm{are}\:\mathrm{in}\:\mathrm{AP}.\:\mathrm{Then}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{determinant} \\ $$$$\begin{vmatrix}{{a}+\mathrm{2}}&{{a}+\mathrm{3}}&{{a}+\mathrm{2}{x}}\\{{a}+\mathrm{3}}&{{a}+\mathrm{4}}&{{a}+\mathrm{2}{y}}\\{{a}+\mathrm{4}}&{{a}+\mathrm{5}}&{{a}+\mathrm{2}{z}}\end{vmatrix}\:\mathrm{is} \\ $$

Question Number 55845 Answers: 0 Comments: 0

If A is 3×4 matrix and B is a matrix such that A′B and BA′ are both defined. Then B is of the type

$$\mathrm{If}\:{A}\:\mathrm{is}\:\mathrm{3}×\mathrm{4}\:\mathrm{matrix}\:\mathrm{and}\:{B}\:\mathrm{is}\:\mathrm{a}\:\mathrm{matrix}\:\mathrm{such} \\ $$$$\mathrm{that}\:{A}'{B}\:\mathrm{and}\:{BA}'\:\mathrm{are}\:\mathrm{both}\:\mathrm{defined}.\:\mathrm{Then} \\ $$$${B}\:\:\:\mathrm{is}\:\mathrm{of}\:\mathrm{the}\:\mathrm{type} \\ $$

Question Number 55844 Answers: 1 Comments: 0

If A is an involutory matrix, then (I+A)(I−A)=0.

$$\mathrm{If}\:{A}\:\mathrm{is}\:\mathrm{an}\:\mathrm{involutory}\:\mathrm{matrix},\:\mathrm{then}\: \\ $$$$\left({I}+{A}\right)\left({I}−{A}\right)=\mathrm{0}. \\ $$

Question Number 55843 Answers: 1 Comments: 1

Consider the system of equations a_1 x+b_1 y+c_1 z=0, a_2 x+b_2 y+c_2 z=0 , a_3 x+b_3 y+c_3 z=0 if determinant ((a_1 ,b_1 ,c_1 ),(a_2 ,b_2 ,c_2 ),(a_3 ,b_3 ,c_3 )) = 0, then the system has

$$\mathrm{Consider}\:\mathrm{the}\:\mathrm{system}\:\mathrm{of}\:\mathrm{equations} \\ $$$${a}_{\mathrm{1}} {x}+{b}_{\mathrm{1}} {y}+{c}_{\mathrm{1}} {z}=\mathrm{0},\:{a}_{\mathrm{2}} {x}+{b}_{\mathrm{2}} {y}+{c}_{\mathrm{2}} {z}=\mathrm{0}\:, \\ $$$${a}_{\mathrm{3}} {x}+{b}_{\mathrm{3}} {y}+{c}_{\mathrm{3}} {z}=\mathrm{0}\:\:\mathrm{if} \\ $$$$\begin{vmatrix}{{a}_{\mathrm{1}} }&{{b}_{\mathrm{1}} }&{{c}_{\mathrm{1}} }\\{{a}_{\mathrm{2}} }&{{b}_{\mathrm{2}} }&{{c}_{\mathrm{2}} }\\{{a}_{\mathrm{3}} }&{{b}_{\mathrm{3}} }&{{c}_{\mathrm{3}} }\end{vmatrix}\:=\:\mathrm{0},\:\mathrm{then}\:\mathrm{the}\:\mathrm{system}\:\mathrm{has} \\ $$

Question Number 55841 Answers: 1 Comments: 0

If A= [(( 4),(x+2)),((2x−3),(x+1)) ] is symmetric, then x=

$$\mathrm{If}\:{A}=\begin{bmatrix}{\:\:\:\:\mathrm{4}}&{{x}+\mathrm{2}}\\{\mathrm{2}{x}−\mathrm{3}}&{{x}+\mathrm{1}}\end{bmatrix}\:\mathrm{is}\:\mathrm{symmetric},\:\mathrm{then}\:{x}= \\ $$

Question Number 55834 Answers: 1 Comments: 0

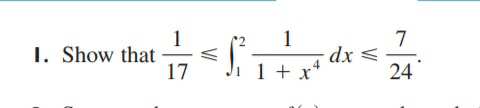
Question Number 55823 Answers: 0 Comments: 0

let f(n,m)= { (1,(n = 1)),((m+1),(n = 2)),((Σ_(i=0) ^m f(n−1,i)),(n > 2)) :} a.compute f(3,4) b.find a function tha satisfies above recursion.

$${let} \\ $$$${f}\left({n},{m}\right)=\begin{cases}{\mathrm{1}}&{{n}\:=\:\mathrm{1}}\\{{m}+\mathrm{1}}&{{n}\:=\:\mathrm{2}}\\{\underset{{i}=\mathrm{0}} {\overset{{m}} {\sum}}{f}\left({n}−\mathrm{1},{i}\right)}&{{n}\:>\:\mathrm{2}}\end{cases} \\ $$$${a}.\mathrm{compute}\:{f}\left(\mathrm{3},\mathrm{4}\right) \\ $$$${b}.\mathrm{find}\:\mathrm{a}\:\mathrm{function}\:\mathrm{tha}\:\mathrm{satisfies}\:\mathrm{above}\:\mathrm{recursion}. \\ $$

Question Number 55820 Answers: 0 Comments: 7

Question Number 55816 Answers: 1 Comments: 0

find grad log ∣r∣

$${find}\:{grad}\:{log}\:\mid\boldsymbol{{r}}\mid \\ $$

Question Number 55815 Answers: 1 Comments: 0

prove that (1+x)^n = 1+nx +((n(n−1))/(2!))x^2 +((n(n−1)(n−2))/(3!))x^3 +...n(n−n) using a suitable expansion method hence determine the expansion of (2.001)^(89)

$${prove}\:{that} \\ $$$$\left(\mathrm{1}+{x}\right)^{{n}} =\:\mathrm{1}+{nx}\:+\frac{{n}\left({n}−\mathrm{1}\right)}{\mathrm{2}!}{x}^{\mathrm{2}} +\frac{{n}\left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)}{\mathrm{3}!}{x}^{\mathrm{3}} +...{n}\left({n}−{n}\right) \\ $$$${using}\:{a}\:{suitable}\:{expansion}\:{method} \\ $$$${hence}\:{determine}\:{the}\:{expansion}\:{of} \\ $$$$\left(\mathrm{2}.\mathrm{001}\right)^{\mathrm{89}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Question Number 55813 Answers: 0 Comments: 0

((0.8)/x)=((96)/(60))⇒x=0.5⇒0.8−0.5=0.3⇒0.4−0.3=0.1

$$\frac{\mathrm{0}.\mathrm{8}}{{x}}=\frac{\mathrm{96}}{\mathrm{60}}\Rightarrow{x}=\mathrm{0}.\mathrm{5}\Rightarrow\mathrm{0}.\mathrm{8}−\mathrm{0}.\mathrm{5}=\mathrm{0}.\mathrm{3}\Rightarrow\mathrm{0}.\mathrm{4}−\mathrm{0}.\mathrm{3}=\mathrm{0}.\mathrm{1} \\ $$

Question Number 55806 Answers: 1 Comments: 0

Solve for x: x^(log_3 2) = (√x) + 1

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{x}:\:\:\:\mathrm{x}^{\mathrm{log}_{\mathrm{3}} \mathrm{2}} \:\:=\:\:\sqrt{\mathrm{x}}\:\:+\:\:\mathrm{1} \\ $$

Question Number 55805 Answers: 0 Comments: 1