
AllQuestion and Answers: Page 1529
Question Number 56818 Answers: 0 Comments: 2

Question Number 56803 Answers: 2 Comments: 2
Question Number 56795 Answers: 1 Comments: 1
Question Number 56801 Answers: 1 Comments: 0
Question Number 56800 Answers: 0 Comments: 3
Question Number 56813 Answers: 0 Comments: 0
Question Number 56785 Answers: 0 Comments: 1

Question Number 56784 Answers: 0 Comments: 0
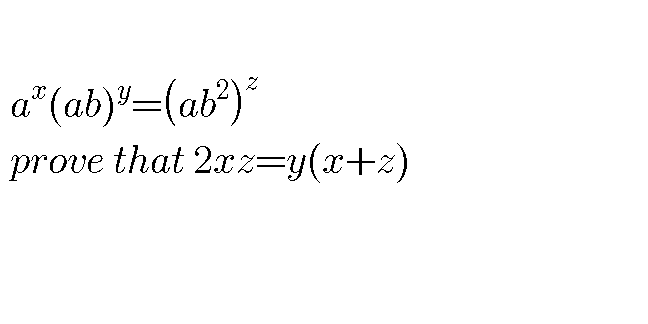
Question Number 56777 Answers: 1 Comments: 1

Question Number 56775 Answers: 0 Comments: 1
Question Number 56772 Answers: 1 Comments: 0
Question Number 56749 Answers: 1 Comments: 0
Question Number 56747 Answers: 1 Comments: 0
$$\int\:\mathrm{x}^{\mathrm{2}\:} \mathrm{e}^{\mathrm{x}^{\mathrm{2}} } \:\:\mathrm{dx} \\ $$
Question Number 56744 Answers: 1 Comments: 0
Question Number 56743 Answers: 1 Comments: 0

Question Number 56738 Answers: 1 Comments: 0
Question Number 56732 Answers: 1 Comments: 1

Question Number 56763 Answers: 1 Comments: 0
Question Number 56711 Answers: 1 Comments: 0
Question Number 56708 Answers: 0 Comments: 1
Question Number 56707 Answers: 1 Comments: 0
Question Number 56706 Answers: 0 Comments: 1
Question Number 56705 Answers: 1 Comments: 0
Question Number 56700 Answers: 1 Comments: 2
$$\:{find}\:\int\:\sqrt{{x}−\mathrm{2}\sqrt{{x}}+\mathrm{3}}{dx} \\ $$
Question Number 56699 Answers: 0 Comments: 2
Question Number 56698 Answers: 0 Comments: 2
Pg 1524 Pg 1525 Pg 1526 Pg 1527 Pg 1528 Pg 1529 Pg 1530 Pg 1531 Pg 1532 Pg 1533
