Question and Answers Forum

AllQuestion and Answers: Page 1520

Question Number 57228 Answers: 0 Comments: 1

find f(x) =∫_1 ^2 ((ln(1+xt))/t^2 ) dt with x>0

$${find}\:{f}\left({x}\right)\:=\int_{\mathrm{1}} ^{\mathrm{2}} \:\frac{{ln}\left(\mathrm{1}+{xt}\right)}{{t}^{\mathrm{2}} }\:{dt}\:\:{with}\:{x}>\mathrm{0} \\ $$

Question Number 57227 Answers: 0 Comments: 0

let f(α)=∫_0 ^1 ((arctan(αx))/(1+αx^2 )) dx with α real 1) find f(α) interms of α 2) find the values of ∫_0 ^1 ((arctan(2x))/(1+2x^2 )) dx and ∫_0 ^1 ((arctan(4x))/(1+4x^2 ))dx

$${let}\:{f}\left(\alpha\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{arctan}\left(\alpha{x}\right)}{\mathrm{1}+\alpha{x}^{\mathrm{2}} }\:{dx}\:\:\:{with}\:\alpha\:{real} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{f}\left(\alpha\right)\:{interms}\:{of}\:\alpha \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{values}\:{of}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{arctan}\left(\mathrm{2}{x}\right)}{\mathrm{1}+\mathrm{2}{x}^{\mathrm{2}} }\:{dx}\:{and}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{arctan}\left(\mathrm{4}{x}\right)}{\mathrm{1}+\mathrm{4}{x}^{\mathrm{2}} }{dx} \\ $$

Question Number 57226 Answers: 0 Comments: 0

calculate A_n =∫_0 ^1 x^n (√((1−x)/(1+x)))dx with n integr natural

$${calculate}\:{A}_{{n}} =\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{{n}} \sqrt{\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}}{dx}\:\:{with}\:{n}\:{integr}\:{natural} \\ $$

Question Number 57224 Answers: 1 Comments: 1

find the value of ∫_0 ^1 ((3t^2 −5t +1)/((t+1)(t+2)(2t+3)))dt

$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{3}{t}^{\mathrm{2}} −\mathrm{5}{t}\:+\mathrm{1}}{\left({t}+\mathrm{1}\right)\left({t}+\mathrm{2}\right)\left(\mathrm{2}{t}+\mathrm{3}\right)}{dt} \\ $$

Question Number 57225 Answers: 0 Comments: 2

1)calculate f(a) =∫_0 ^a ((2x−1)/((x^2 −x+3)(x^2 +1)))dx 1) calculate f(1)and f(2)

$$\left.\mathrm{1}\right){calculate}\:{f}\left({a}\right)\:=\int_{\mathrm{0}} ^{{a}} \:\:\:\frac{\mathrm{2}{x}−\mathrm{1}}{\left({x}^{\mathrm{2}} \:−{x}+\mathrm{3}\right)\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)}{dx} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}\left(\mathrm{1}\right){and}\:{f}\left(\mathrm{2}\right) \\ $$

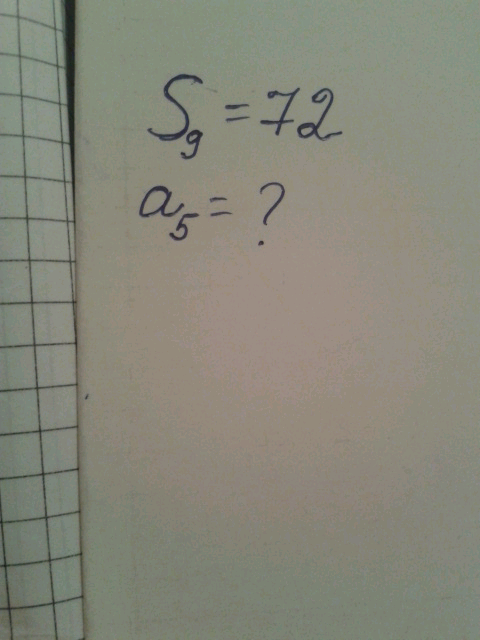
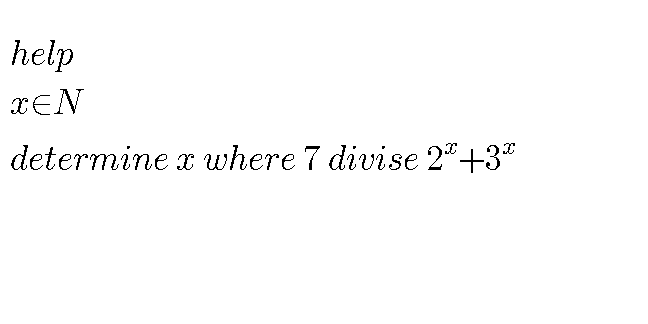
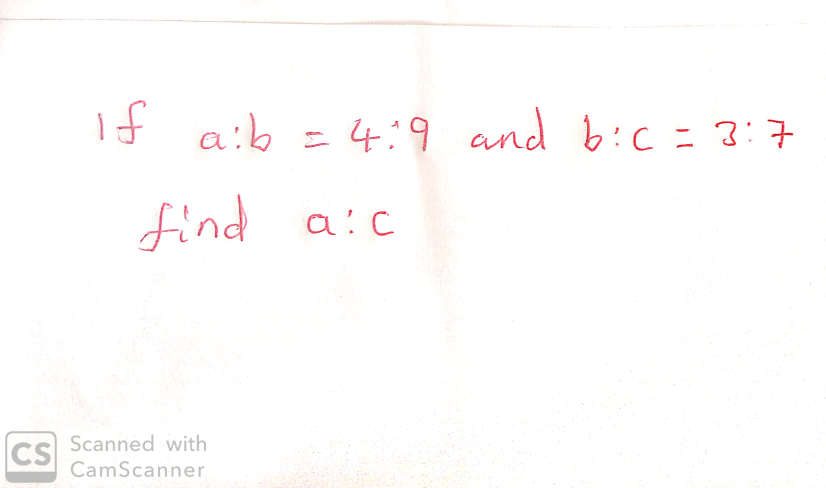
Question Number 57222 Answers: 1 Comments: 0

Express 5.27 in form of a series and show that is equal to 5 (5/(18))

$$\mathrm{Express}\:\:\:\mathrm{5}.\mathrm{27}\:\:\mathrm{in}\:\mathrm{form}\:\mathrm{of}\:\mathrm{a}\:\mathrm{series}\:\mathrm{and}\:\mathrm{show}\:\mathrm{that}\:\mathrm{is}\:\mathrm{equal} \\ $$$$\mathrm{to}\:\:\:\mathrm{5}\:\frac{\mathrm{5}}{\mathrm{18}} \\ $$

Question Number 57241 Answers: 1 Comments: 0

∫((×(√(x+1)))/(x+2))dx

$$\int\frac{×\sqrt{\mathrm{x}+\mathrm{1}}}{\mathrm{x}+\mathrm{2}}\mathrm{dx} \\ $$

Question Number 57212 Answers: 1 Comments: 0

Question Number 57194 Answers: 0 Comments: 1

let A_n =∫_n ^n (([(√(x+1))]−[(√x)])/x) dx with n natural integr and n≥1 1) find A_n interms of n 2)find nature of the serie Σ A_n

$${let}\:\:{A}_{{n}} =\int_{{n}} ^{{n}} \:\frac{\left[\sqrt{{x}+\mathrm{1}}\right]−\left[\sqrt{{x}}\right]}{{x}}\:{dx}\:\:\:{with}\:{n}\:{natural}\:{integr}\:{and}\:{n}\geqslant\mathrm{1} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{A}_{{n}} \:{interms}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right){find}\:{nature}\:{of}\:{the}\:{serie}\:\Sigma\:{A}_{{n}} \\ $$

Question Number 57186 Answers: 1 Comments: 3

Question Number 57184 Answers: 2 Comments: 1

Question Number 57237 Answers: 0 Comments: 2

let f(x) =∫_0 ^(+∞) ((sin(xt^2 −1))/(t^4 +1)) dt 1) find a explicit form of f(x) 2) let g(x) =∫_0 ^∞ ((t^2 cos(xt^2 −1))/(t^4 +1)) dt find a explicit form of g(x) 3) calculate ∫_0 ^∞ ((sin(2t^2 −1))/(t^4 +1)) dt and ∫_0 ^∞ ((t^2 cos(3t^2 −1))/(t^4 +1)) dt .

$${let}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{+\infty} \:\:\frac{{sin}\left({xt}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt} \\ $$$$\left.\mathrm{1}\right)\:{find}\:\:{a}\:{explicit}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{let}\:{g}\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{t}^{\mathrm{2}} \:{cos}\left({xt}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt} \\ $$$${find}\:{a}\:{explicit}\:{form}\:{of}\:{g}\left({x}\right) \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{sin}\left(\mathrm{2}{t}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt}\:\:{and}\:\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{t}^{\mathrm{2}} \:{cos}\left(\mathrm{3}{t}^{\mathrm{2}} −\mathrm{1}\right)}{{t}^{\mathrm{4}} \:+\mathrm{1}}\:{dt}\:. \\ $$

Question Number 57236 Answers: 1 Comments: 1

clalculate A_n = ∫_0 ^1 t^(2n) (1−t)^n dt with n integr natural .

$${clalculate}\:{A}_{{n}} =\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{t}^{\mathrm{2}{n}} \left(\mathrm{1}−{t}\right)^{{n}} {dt}\:\:\:{with}\:{n}\:{integr}\:{natural}\:. \\ $$

Question Number 57235 Answers: 1 Comments: 1

calculate ∫_0 ^(π/2) ((cosx −sinx)/(√(cos^8 x +sin^8 x))) dx

$${calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{cosx}\:−{sinx}}{\sqrt{{cos}^{\mathrm{8}} {x}\:+{sin}^{\mathrm{8}} {x}}}\:{dx} \\ $$

Question Number 57234 Answers: 0 Comments: 0

let tbe fraction F(x)=(1/(x^n −1)) with n from n and n≥2 1) find the poles of F and decompose it inside C(x) 2)decompose F(x)inside R(x) 3) calculate ∫_2 ^3 F(x)dx .

$${let}\:{tbe}\:{fraction}\:{F}\left({x}\right)=\frac{\mathrm{1}}{{x}^{{n}} −\mathrm{1}}\:\:{with}\:{n}\:{from}\:{n}\:{and}\:{n}\geqslant\mathrm{2} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{the}\:{poles}\:{of}\:{F}\:{and}\:{decompose}\:{it}\:{inside}\:{C}\left({x}\right) \\ $$$$\left.\mathrm{2}\right){decompose}\:{F}\left({x}\right){inside}\:{R}\left({x}\right) \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\int_{\mathrm{2}} ^{\mathrm{3}} {F}\left({x}\right){dx}\:. \\ $$

Question Number 57233 Answers: 0 Comments: 1

find the value of ∫_0 ^(+∞) (x^4 /((1+x^2 +x^4 )^2 ))dx

$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{+\infty} \:\:\frac{{x}^{\mathrm{4}} }{\left(\mathrm{1}+{x}^{\mathrm{2}} \:+{x}^{\mathrm{4}} \right)^{\mathrm{2}} }{dx} \\ $$

Question Number 57232 Answers: 0 Comments: 1

decompose tbe fraction F(x)=(1/(x^n (x+1))) with n integr natural.

$${decompose}\:{tbe}\:{fraction}\:{F}\left({x}\right)=\frac{\mathrm{1}}{{x}^{{n}} \left({x}+\mathrm{1}\right)}\:\:{with}\:{n}\:{integr}\:{natural}. \\ $$

Question Number 57231 Answers: 0 Comments: 1

find tbe value of ∫_(−∞) ^(+∞) ((x−3)/((x^2 +1)(x^2 −x +2)^2 )) dx

$${find}\:{tbe}\:{value}\:{of}\:\int_{−\infty} ^{+\infty} \:\:\frac{{x}−\mathrm{3}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\left({x}^{\mathrm{2}} −{x}\:+\mathrm{2}\right)^{\mathrm{2}} }\:{dx} \\ $$

Question Number 57230 Answers: 0 Comments: 0

1) find ∫ (dx/(x^2 (x+1)^3 (x^2 +1))) 2) calculate ∫_1 ^(√3) (dx/(x^2 (x+1)^3 (x^2 +1)))