
AllQuestion and Answers: Page 1517
Question Number 58079 Answers: 1 Comments: 0
Question Number 58077 Answers: 1 Comments: 0
Question Number 58076 Answers: 2 Comments: 1
Question Number 58060 Answers: 0 Comments: 3
Question Number 58056 Answers: 1 Comments: 0
Question Number 58055 Answers: 1 Comments: 0
Question Number 58051 Answers: 1 Comments: 0

Question Number 58050 Answers: 1 Comments: 0

Question Number 58049 Answers: 0 Comments: 0

Question Number 58046 Answers: 2 Comments: 0
Question Number 58043 Answers: 0 Comments: 1
Question Number 58069 Answers: 1 Comments: 4
Question Number 58067 Answers: 0 Comments: 1
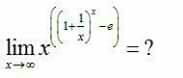
Question Number 58027 Answers: 1 Comments: 0
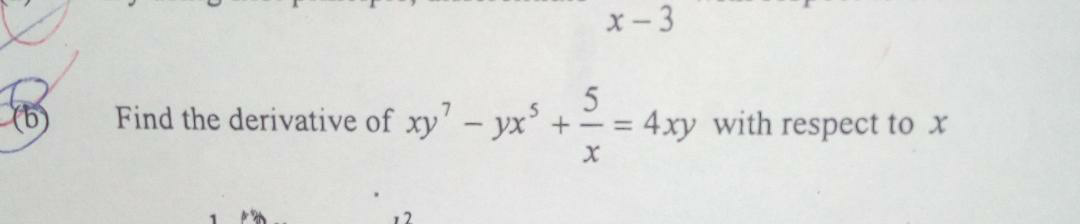
Question Number 58025 Answers: 1 Comments: 1
Question Number 58016 Answers: 2 Comments: 1
Question Number 57997 Answers: 0 Comments: 2
Question Number 57992 Answers: 1 Comments: 0

Question Number 57991 Answers: 0 Comments: 0

Question Number 57988 Answers: 0 Comments: 5
Question Number 57985 Answers: 2 Comments: 0
Question Number 57984 Answers: 0 Comments: 0

Question Number 58045 Answers: 1 Comments: 0
Question Number 57977 Answers: 0 Comments: 0
Question Number 57960 Answers: 1 Comments: 0
$${Find}\:{maximum}\:{n}\:{such}\:{that}\:\mathrm{12}^{{n}} \:{divides} \\ $$$$\mathrm{100}!. \\ $$
Question Number 57959 Answers: 1 Comments: 0

Pg 1512 Pg 1513 Pg 1514 Pg 1515 Pg 1516 Pg 1517 Pg 1518 Pg 1519 Pg 1520 Pg 1521
