Question and Answers Forum

AllQuestion and Answers: Page 1515

Question Number 59094 Answers: 1 Comments: 1

Question Number 59092 Answers: 1 Comments: 0

what % increase will it be to transform an image on a scale of 1:75 to 1:300?

$${what}\:\%\:{increase}\:{will}\:{it}\:{be}\:{to}\:{transform} \\ $$$${an}\:{image}\:{on}\:{a}\:{scale}\:{of}\:\mathrm{1}:\mathrm{75}\:{to}\:\mathrm{1}:\mathrm{300}? \\ $$

Question Number 59089 Answers: 1 Comments: 0

Question Number 59080 Answers: 0 Comments: 0

Question Number 59079 Answers: 0 Comments: 0

Question Number 59077 Answers: 0 Comments: 0

Question Number 59076 Answers: 0 Comments: 0

Question Number 59075 Answers: 0 Comments: 1

Question Number 59074 Answers: 0 Comments: 0

Question Number 59070 Answers: 3 Comments: 0

Question Number 59060 Answers: 2 Comments: 0

Question Number 59059 Answers: 0 Comments: 0

Question Number 59058 Answers: 2 Comments: 1

Question Number 59053 Answers: 0 Comments: 0

f=v v=47×48 f=?

$${f}={v} \\ $$$${v}=\mathrm{47}×\mathrm{48} \\ $$$${f}=? \\ $$

Question Number 59052 Answers: 0 Comments: 0

let f(x) =∫_0 ^(π/2) ln(cosθ +xsinθ)dθ with x fromR 1) determine a explicit form for f(x) 2) calculate ∫_0 ^(π/2) ln(cosθ +sinθ) dθ and ∫_0 ^(π/2) ln(cosθ +2sinθ)dθ .

$${let}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left({cos}\theta\:+{xsin}\theta\right){d}\theta\:\:\:{with}\:{x}\:{fromR} \\ $$$$\left.\mathrm{1}\right)\:{determine}\:{a}\:{explicit}\:{form}\:\:{for}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left({cos}\theta\:+{sin}\theta\right)\:{d}\theta\:\:{and}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {ln}\left({cos}\theta\:+\mathrm{2}{sin}\theta\right){d}\theta\:. \\ $$

Question Number 59050 Answers: 1 Comments: 0

calculate ∫_0 ^∞ ((arctan(x^2 ))/(1+x^2 )) dx

$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{arctan}\left({x}^{\mathrm{2}} \right)}{\mathrm{1}+{x}^{\mathrm{2}} }\:{dx}\: \\ $$

Question Number 59049 Answers: 0 Comments: 1

∫_( 0) ^∞ ((x log x)/((1+x^2 )^2 )) dx =

$$\:\underset{\:\mathrm{0}} {\overset{\infty} {\int}}\:\frac{{x}\:\mathrm{log}\:{x}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} }\:{dx}\:= \\ $$

Question Number 59040 Answers: 3 Comments: 0

1) ∫_0 ^( (π/4)) ((sin x+cos x)/(cos^2 x+sin^4 x))dx = ?

$$\left.\mathrm{1}\right)\:\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{sin}\:{x}+\mathrm{cos}\:{x}}{\mathrm{cos}\:^{\mathrm{2}} {x}+\mathrm{sin}\:^{\mathrm{4}} {x}}{dx}\:=\:? \\ $$

Question Number 59023 Answers: 0 Comments: 0

please solve my quens

$${please}\:{solve}\:{my}\:{quens} \\ $$

Question Number 59021 Answers: 2 Comments: 10

[Q58885 reposted] How many 4−digit numbers abcd exist which are divisible by 3 and satisfy a≤b≤c≤d?

$$\left[{Q}\mathrm{58885}\:{reposted}\right] \\ $$$${How}\:{many}\:\mathrm{4}−{digit}\:{numbers}\:{abcd}\:{exist} \\ $$$${which}\:{are}\:{divisible}\:{by}\:\mathrm{3}\:{and}\:{satisfy} \\ $$$${a}\leqslant{b}\leqslant{c}\leqslant{d}? \\ $$

Question Number 59018 Answers: 0 Comments: 1

Question Number 59012 Answers: 3 Comments: 0

Question Number 59006 Answers: 0 Comments: 1

probar con h≠0 ((sin(x+h)−sin(x))/h)=((sin(h/2))/(h/2))cos(x+(h/2))

$${probar}\:{con}\:{h}\neq\mathrm{0} \\ $$$$\frac{{sin}\left({x}+{h}\right)−{sin}\left({x}\right)}{{h}}=\frac{{sin}\left({h}/\mathrm{2}\right)}{{h}/\mathrm{2}}{cos}\left({x}+\frac{{h}}{\mathrm{2}}\right) \\ $$$$ \\ $$

Question Number 59003 Answers: 0 Comments: 0

Question Number 59002 Answers: 0 Comments: 0

Question Number 59000 Answers: 1 Comments: 0

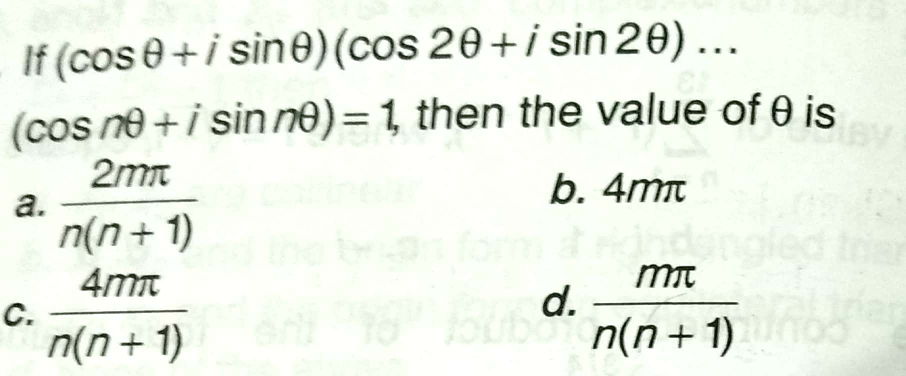
Pg 1510 Pg 1511 Pg 1512 Pg 1513 Pg 1514 Pg 1515 Pg 1516 Pg 1517 Pg 1518 Pg 1519

Contact: info@tinkutara.com