Question and Answers Forum

let f(x) =∫_0 ^∞ ((cos(πxt))/((t^2 +3x^2 )^2 )) dt with x>0 1) find a explicit form for f(x) 2) find the value of ∫_0 ^∞ ((cos(πt))/((t^2 +3)^2 ))dt 3) let U_n =f(n) find nature of Σ U_n

$${let}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{cos}\left(\pi{xt}\right)}{\left({t}^{\mathrm{2}} \:+\mathrm{3}{x}^{\mathrm{2}} \right)^{\mathrm{2}} }\:{dt}\:{with}\:{x}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{explicit}\:{form}\:{for}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{cos}\left(\pi{t}\right)}{\left({t}^{\mathrm{2}} \:+\mathrm{3}\right)^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{3}\right)\:{let}\:{U}_{{n}} ={f}\left({n}\right)\:\:{find}\:{nature}\:{of}\:\Sigma\:{U}_{{n}} \\ $$

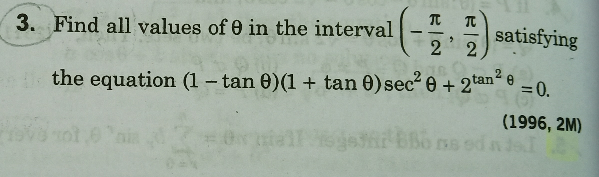
Question Number 57899 Answers: 0 Comments: 2

let f(x) =∫_0 ^(+∞) (dt/((t^2 +x^2 )^3 )) with x>0 1) find a explicit form off (x) 1) calculate ∫_0 ^∞ (dx/((t^2 +3)^3 )) and ∫_0 ^∞ (dt/((t^2 +4)^3 )) 2) find the value of A(θ) =∫_0 ^∞ (dt/((t^2 +sin^2 θ)^3 )) with 0<θ<π.

$${let}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{+\infty} \:\:\frac{{dt}}{\left({t}^{\mathrm{2}} \:+{x}^{\mathrm{2}} \right)^{\mathrm{3}} }\:\:{with}\:{x}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{explicit}\:{form}\:{off}\:\left({x}\right) \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dx}}{\left({t}^{\mathrm{2}} \:+\mathrm{3}\right)^{\mathrm{3}} }\:\:{and}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} \:+\mathrm{4}\right)^{\mathrm{3}} } \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:{A}\left(\theta\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} \:+{sin}^{\mathrm{2}} \theta\right)^{\mathrm{3}} }\:\:{with}\:\mathrm{0}<\theta<\pi. \\ $$

Question Number 57889 Answers: 0 Comments: 0

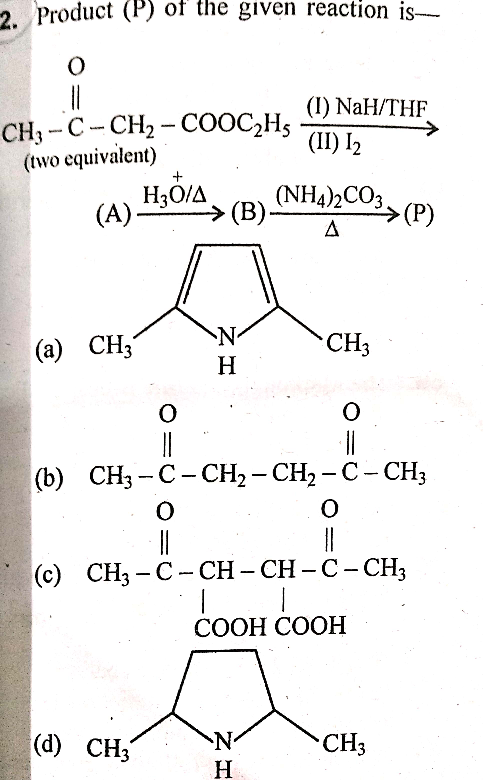
Question Number 57882 Answers: 1 Comments: 1

2[3×4+2×4]

$$\mathrm{2}\left[\mathrm{3}×\mathrm{4}+\mathrm{2}×\mathrm{4}\right] \\ $$

Question Number 57881 Answers: 0 Comments: 0

calculate(2/(13))×2(1/4)

$$\mathrm{calculate}\frac{\mathrm{2}}{\mathrm{13}}×\mathrm{2}\frac{\mathrm{1}}{\mathrm{4}} \\ $$

Question Number 57880 Answers: 0 Comments: 0

6×2

$$\mathrm{6}×\mathrm{2} \\ $$

Question Number 57873 Answers: 1 Comments: 1

If α + β+ γ =180°, show that cos (α/2) + cos (β/2)+ cos (γ/2) = 4 cos ((β + γ)/4) cos ((γ + α)/4) cos ((α + β)/4).

$$\mathrm{If}\:\alpha\:+\:\beta+\:\gamma\:=\mathrm{180}°,\:\mathrm{show}\:\mathrm{that}\:\mathrm{cos}\:\frac{\alpha}{\mathrm{2}}\:+\:\mathrm{cos}\:\frac{\beta}{\mathrm{2}}+\:\mathrm{cos}\:\frac{\gamma}{\mathrm{2}}\:=\:\mathrm{4}\:\mathrm{cos}\:\frac{\beta\:+\:\gamma}{\mathrm{4}}\:\:\mathrm{cos}\:\frac{\gamma\:+\:\alpha}{\mathrm{4}}\:\mathrm{cos}\:\frac{\alpha\:+\:\beta}{\mathrm{4}}. \\ $$

Question Number 57866 Answers: 2 Comments: 0

lim_(n→∞) ((3^(n+2) −2.5^(n+1) )/(3^n −2.5^(n−1) ))

$$\underset{{n}\rightarrow\infty} {{lim}}\:\:\frac{\mathrm{3}^{{n}+\mathrm{2}} −\mathrm{2}.\mathrm{5}^{{n}+\mathrm{1}} }{\mathrm{3}^{{n}} −\mathrm{2}.\mathrm{5}^{{n}−\mathrm{1}} } \\ $$

Question Number 57865 Answers: 2 Comments: 0

Question Number 57863 Answers: 1 Comments: 2

Question Number 57864 Answers: 1 Comments: 0

Question Number 58001 Answers: 1 Comments: 1

Question Number 57850 Answers: 1 Comments: 0

lim_(n→∞) (8^n /(2^(n+1) +3^(n+2) ))

$$\underset{{n}\rightarrow\infty} {{lim}}\:\:\frac{\mathrm{8}^{{n}} }{\mathrm{2}^{{n}+\mathrm{1}} +\mathrm{3}^{{n}+\mathrm{2}} } \\ $$

Question Number 57848 Answers: 0 Comments: 2

1)prove that arctan(a) +arctanb =arctan(((a+b)/(1−ab))) with ab≠1 2)find the value of S_N = Σ_(n=1) ^N (−1)^n arctan(((2n+1)/(n^2 +n−1)))

$$\left.\mathrm{1}\right){prove}\:{that}\:{arctan}\left({a}\right)\:+{arctanb}\:={arctan}\left(\frac{{a}+{b}}{\mathrm{1}−{ab}}\right)\: \\ $$$${with}\:{ab}\neq\mathrm{1} \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:{S}_{{N}} =\:\sum_{{n}=\mathrm{1}} ^{{N}} \left(−\mathrm{1}\right)^{{n}} \:{arctan}\left(\frac{\mathrm{2}{n}+\mathrm{1}}{{n}^{\mathrm{2}} \:+{n}−\mathrm{1}}\right) \\ $$

Question Number 57847 Answers: 0 Comments: 1

(U_n ) is a sequence wich verify u_n +u_(n+1) =(1/n^2 ) 1) find u_n interms of n 2) find lim_(n→+∞) u_n

$$\left({U}_{{n}} \right)\:{is}\:{a}\:{sequence}\:{wich}\:{verify}\: \\ $$$${u}_{{n}} +{u}_{{n}+\mathrm{1}} =\frac{\mathrm{1}}{{n}^{\mathrm{2}} } \\ $$$$\left.\mathrm{1}\right)\:{find}\:{u}_{{n}} \:{interms}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{lim}_{{n}\rightarrow+\infty} \:{u}_{{n}} \\ $$

Question Number 57840 Answers: 0 Comments: 0

A uniform rod ABC, weight 50N and length 4m rests with one end A on rough horizontal ground and is supported by a smooth peg at B where AB=2.5m. The peg is 2m from the ground. Find the reactions at A and at the peg.

$$\mathrm{A}\:\mathrm{uniform}\:\mathrm{rod}\:\mathrm{ABC},\:\mathrm{weight}\:\mathrm{50N}\:\mathrm{and}\: \\ $$$$\mathrm{length}\:\mathrm{4m}\:\mathrm{rests}\:\mathrm{with}\:\mathrm{one}\:\mathrm{end}\:\mathrm{A}\:\mathrm{on}\:\mathrm{rough} \\ $$$$\mathrm{horizontal}\:\mathrm{ground}\:\mathrm{and}\:\mathrm{is}\:\mathrm{supported}\:\mathrm{by}\: \\ $$$$\mathrm{a}\:\mathrm{smooth}\:\mathrm{peg}\:\mathrm{at}\:\mathrm{B}\:\mathrm{where}\:\mathrm{AB}=\mathrm{2}.\mathrm{5m}. \\ $$$$\mathrm{The}\:\mathrm{peg}\:\mathrm{is}\:\mathrm{2m}\:\mathrm{from}\:\mathrm{the}\:\mathrm{ground}.\:\mathrm{Find}\:\mathrm{the} \\ $$$$\mathrm{reactions}\:\mathrm{at}\:\mathrm{A}\:\mathrm{and}\:\mathrm{at}\:\mathrm{the}\:\mathrm{peg}. \\ $$

Pg 1509 Pg 1510 Pg 1511 Pg 1512 Pg 1513 Pg 1514 Pg 1515 Pg 1516 Pg 1517 Pg 1518

Contact: info@tinkutara.com