Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1512

Question Number 58974 Answers: 1 Comments: 0

Question Number 58972 Answers: 0 Comments: 0

Question Number 58971 Answers: 1 Comments: 1

Question Number 58965 Answers: 0 Comments: 0

if a,b ∈C ∣ ∣a∣<1,∣b∣<1 ⇒∣((a−b)/(1−a^ b))∣<1^

$$\mathrm{if}\:{a},{b}\:\in\mathbb{C}\:\mid\:\mid{a}\mid<\mathrm{1},\mid{b}\mid<\mathrm{1} \\ $$$$\Rightarrow\mid\frac{{a}−{b}}{\mathrm{1}−\bar {{a}b}}\mid<\overset{} {\mathrm{1}} \\ $$

Question Number 58964 Answers: 1 Comments: 0

a + b + c = 1 a, b, c ≤ 1 Prove that (1/(a^2 + 1)) + (1/(b^2 + 1)) + (1/(c^2 + 1)) ≤ ((27)/(10))

$${a}\:+\:{b}\:+\:{c}\:\:=\:\:\mathrm{1} \\ $$$${a},\:{b},\:{c}\:\:\leqslant\:\:\mathrm{1} \\ $$$${Prove}\:\:{that} \\ $$$$\:\:\:\:\:\:\frac{\mathrm{1}}{{a}^{\mathrm{2}} \:+\:\mathrm{1}}\:\:+\:\:\frac{\mathrm{1}}{{b}^{\mathrm{2}} \:+\:\mathrm{1}}\:\:+\:\:\frac{\mathrm{1}}{{c}^{\mathrm{2}} \:+\:\mathrm{1}}\:\:\leqslant\:\:\frac{\mathrm{27}}{\mathrm{10}} \\ $$

Question Number 58963 Answers: 1 Comments: 0

solve x^2 −2(1+i)x−5+14i=0

$${solve}\:{x}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{1}+{i}\right){x}−\mathrm{5}+\mathrm{14}{i}=\mathrm{0} \\ $$

Question Number 58962 Answers: 0 Comments: 0

you are welcome sir.

$${you}\:{are}\:{welcome}\:{sir}. \\ $$

Question Number 59037 Answers: 0 Comments: 1

solve (dy/dt)=t^2 +y^2

$${solve}\:\:\frac{{dy}}{{dt}}={t}^{\mathrm{2}} +{y}^{\mathrm{2}} \\ $$

Question Number 58948 Answers: 0 Comments: 3

lim_(x→+∞) ((√x)/(√(x+(√(x+(√x))))))

$$\underset{{x}\rightarrow+\infty} {{lim}}\:\frac{\sqrt{{x}}}{\sqrt{{x}+\sqrt{{x}+\sqrt{{x}}}}} \\ $$

Question Number 58943 Answers: 1 Comments: 0

Add 469+143

$$\mathrm{Add}\:\mathrm{469}+\mathrm{143} \\ $$

Question Number 58942 Answers: 1 Comments: 0

4×(1/(10))

$$\mathrm{4}×\frac{\mathrm{1}}{\mathrm{10}} \\ $$

Question Number 58941 Answers: 1 Comments: 0

6h=30

$$\mathrm{6h}=\mathrm{30} \\ $$

Question Number 58940 Answers: 1 Comments: 3

e^(i∫_(−2) ^2 (x^2 sinx+(√(1−(x^2 /4))))dx) +lim_(x→2) ((∫_2 ^x log(x+8)dx)/(x−2))=?

$${e}^{{i}\int_{−\mathrm{2}} ^{\mathrm{2}} \left({x}^{\mathrm{2}} {sinx}+\sqrt{\mathrm{1}−\frac{{x}^{\mathrm{2}} }{\mathrm{4}}}\right){dx}} +\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\frac{\int_{\mathrm{2}} ^{{x}} {log}\left({x}+\mathrm{8}\right){dx}}{{x}−\mathrm{2}}=? \\ $$

Question Number 58937 Answers: 0 Comments: 2

∫_0 ^2 lim_((1/n)→0) (((2−x)(x+x^n ))/(1+x^n ))dx= ?

$$\int_{\mathrm{0}} ^{\mathrm{2}} \underset{\frac{\mathrm{1}}{{n}}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left(\mathrm{2}−{x}\right)\left({x}+{x}^{{n}} \right)}{\mathrm{1}+{x}^{{n}} }{dx}=\:? \\ $$

Question Number 58936 Answers: 1 Comments: 0

2+{[5+6]}×2

$$\mathrm{2}+\left\{\left[\mathrm{5}+\mathrm{6}\right]\right\}×\mathrm{2} \\ $$

Question Number 58934 Answers: 1 Comments: 0

856×16

$$\mathrm{856}×\mathrm{16} \\ $$

Question Number 58932 Answers: 0 Comments: 1

6h=18

$$\mathrm{6h}=\mathrm{18} \\ $$

Question Number 58928 Answers: 0 Comments: 2

Question Number 58915 Answers: 2 Comments: 2

reposting this: x^8 −8x^7 −16x^6 +208x^5 −152x^4 −928x^3 +704x^2 +1088x−368=0 nobody wants to try? it′s beautiful...

$$\mathrm{reposting}\:\mathrm{this}: \\ $$$${x}^{\mathrm{8}} −\mathrm{8}{x}^{\mathrm{7}} −\mathrm{16}{x}^{\mathrm{6}} +\mathrm{208}{x}^{\mathrm{5}} −\mathrm{152}{x}^{\mathrm{4}} −\mathrm{928}{x}^{\mathrm{3}} +\mathrm{704}{x}^{\mathrm{2}} +\mathrm{1088}{x}−\mathrm{368}=\mathrm{0} \\ $$$$\mathrm{nobody}\:\mathrm{wants}\:\mathrm{to}\:\mathrm{try}?\:\mathrm{it}'\mathrm{s}\:\mathrm{beautiful}... \\ $$

Question Number 58899 Answers: 1 Comments: 4

Solve for x: x^(x^x ) = 729

$$\boldsymbol{\mathrm{S}}\mathrm{olve}\:\mathrm{for}\:\:\mathrm{x}:\:\:\:\:\:\mathrm{x}^{\mathrm{x}^{\mathrm{x}} \:\:} =\:\:\mathrm{729} \\ $$

Question Number 58896 Answers: 2 Comments: 0

11) lg_4 lg_4 lg_2 16−lg_2 lg_2 (√3) 12. (5lg_3 3−lg_4 1)^2 +(((1/(lg_2 8))×lg_3 27)/(lg_(√2) (1/2)))

$$\left.\mathrm{11}\right)\:\:\mathrm{lg}_{\mathrm{4}} \mathrm{lg}_{\mathrm{4}} \mathrm{lg}_{\mathrm{2}} \mathrm{16}−\mathrm{lg}_{\mathrm{2}} \mathrm{lg}_{\mathrm{2}} \sqrt{\mathrm{3}} \\ $$$$\mathrm{12}.\:\left(\mathrm{5lg}_{\mathrm{3}} \mathrm{3}−\mathrm{lg}_{\mathrm{4}} \mathrm{1}\right)^{\mathrm{2}} +\frac{\frac{\mathrm{1}}{\mathrm{lg}_{\mathrm{2}} \mathrm{8}}×\mathrm{lg}_{\mathrm{3}} \mathrm{27}}{\mathrm{lg}_{\sqrt{\mathrm{2}}} \frac{\mathrm{1}}{\mathrm{2}}} \\ $$

Question Number 58885 Answers: 0 Comments: 2

How many 4−digits number abcd that satisfy a ≤ b ≤ c ≤ d multiply of 3 .

$${How}\:\:{many}\:\:\mathrm{4}−{digits}\:\:{number}\:\:\:{abcd}\:\:\:{that} \\ $$$${satisfy}\:\:\:\:{a}\:\:\leqslant\:\:{b}\:\:\leqslant\:\:{c}\:\:\leqslant\:\:{d}\:\:\:\:{multiply}\:\:\:{of}\:\:\:\mathrm{3}\:.\: \\ $$

Question Number 58880 Answers: 0 Comments: 1

t=(1/(1−4^(1/4) )) find the value of t

$${t}=\frac{\mathrm{1}}{\mathrm{1}−\mathrm{4}^{\frac{\mathrm{1}}{\mathrm{4}}} }\:\:\:\:\:\:{find}\:{the}\:{value}\:{of}\:{t} \\ $$

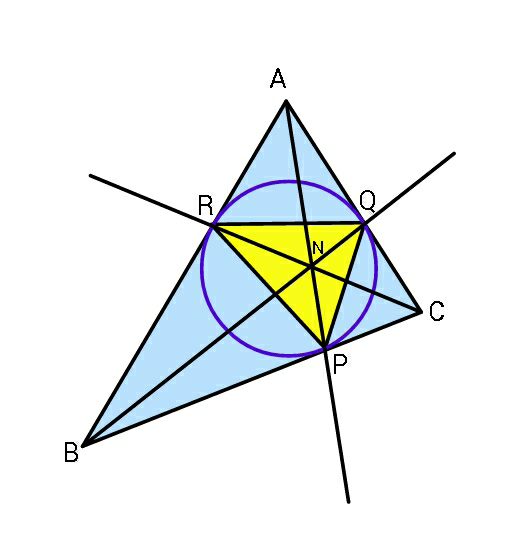
Question Number 58879 Answers: 1 Comments: 0

Question Number 58877 Answers: 1 Comments: 2

Question Number 58876 Answers: 1 Comments: 4

Pg 1507 Pg 1508 Pg 1509 Pg 1510 Pg 1511 Pg 1512 Pg 1513 Pg 1514 Pg 1515 Pg 1516

Contact: info@tinkutara.com