Question and Answers Forum

AllQuestion and Answers: Page 1509

Question Number 58521 Answers: 1 Comments: 0

sin 16^° =? cos 16^° =? without using cos(3θ) algebric method please

$$\boldsymbol{{sin}}\:\mathrm{16}^{°} \:=? \\ $$$$\boldsymbol{{cos}}\:\mathrm{16}^{°} \:=? \\ $$$$\boldsymbol{{without}}\:\boldsymbol{{using}}\:\boldsymbol{{cos}}\left(\mathrm{3}\theta\right) \\ $$$$\boldsymbol{{algebric}}\:\boldsymbol{{method}}\:\boldsymbol{{please}} \\ $$

Question Number 58519 Answers: 1 Comments: 1

Question Number 58512 Answers: 1 Comments: 1

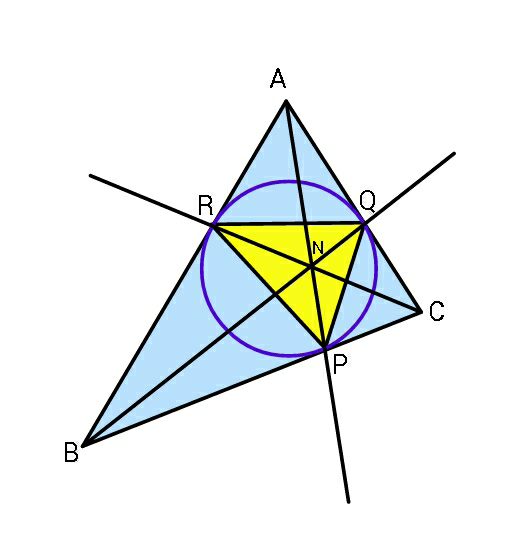
Question Number 58508 Answers: 1 Comments: 0

Question Number 58501 Answers: 2 Comments: 0

a and b are roots of this equation : x^(2018) − 2x + 1 = 0 Calculate the value of 2 + (a+b) + (a^2 +b^2 ) + (a^3 + b^3 ) + … + (a^(2017) + b^(2017) )

$${a}\:\:{and}\:\:{b}\:\:{are}\:\:{roots}\:\:{of}\:\:{this}\:\:{equation}\:\:: \\ $$$$\:\:\:\:\:\:\:\:{x}^{\mathrm{2018}} \:−\:\mathrm{2}{x}\:+\:\mathrm{1}\:\:=\:\:\mathrm{0} \\ $$$${Calculate}\:\:{the}\:\:{value}\:\:\:{of} \\ $$$$\:\:\mathrm{2}\:+\:\left({a}+{b}\right)\:+\:\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\:+\:\left({a}^{\mathrm{3}} \:+\:{b}^{\mathrm{3}} \right)\:+\:\ldots\:+\:\left({a}^{\mathrm{2017}} \:+\:{b}^{\mathrm{2017}} \right) \\ $$

Question Number 58500 Answers: 2 Comments: 0

change in simplest form : tan^(−1) (((√(1+x^2 ))+(√(1−x^2 )))/((√(1+x^2 ))−(√(1−x^2 ))))

$${change}\:{in}\:{simplest}\:{form}\:: \\ $$$$\mathrm{tan}^{−\mathrm{1}} \frac{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }+\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }−\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }} \\ $$

Question Number 58493 Answers: 1 Comments: 0

Question Number 58488 Answers: 0 Comments: 2

let f(x) =∫ (dt/(x +cost +cos(2t))) (x real) 1) find a explicit form of f(x) 2)determine also ∫ (dt/((x+cost +cos(2t))^2 )) 3) find ∫ (dt/(1+cos(t)+cos(2t))) and ∫ (dt/((3 +cos(t)+cos(2t))^2 ))

$${let}\:{f}\left({x}\right)\:=\int\:\:\:\frac{{dt}}{{x}\:+{cost}\:+{cos}\left(\mathrm{2}{t}\right)}\:\:\left({x}\:{real}\right) \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{explicit}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right){determine}\:{also}\:\int\:\:\frac{{dt}}{\left({x}+{cost}\:+{cos}\left(\mathrm{2}{t}\right)\right)^{\mathrm{2}} } \\ $$$$\left.\mathrm{3}\right)\:{find}\:\int\:\:\:\frac{{dt}}{\mathrm{1}+{cos}\left({t}\right)+{cos}\left(\mathrm{2}{t}\right)}\:{and} \\ $$$$\int\:\:\:\frac{{dt}}{\left(\mathrm{3}\:+{cos}\left({t}\right)+{cos}\left(\mathrm{2}{t}\right)\right)^{\mathrm{2}} } \\ $$

Question Number 58487 Answers: 0 Comments: 3

let f(x) =∫_(π/4) ^(π/3) (dt/(2+xsint)) 1) find a explicit form of f(x) 2)determine also g(x)=∫_(π/4) ^(π/3) ((sint)/((2+xsint)^2 ))dt 3) find the value of ∫_(π/4) ^(π/3) (dt/(2+3sint)) and ∫_(π/4) ^(π/3) ((sint)/((2+3sint)^2 ))dt

$${let}\:{f}\left({x}\right)\:=\int_{\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{3}}} \:\:\:\frac{{dt}}{\mathrm{2}+{xsint}} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{a}\:{explicit}\:{form}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{2}\right){determine}\:{also}\:{g}\left({x}\right)=\int_{\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{3}}} \:\:\:\frac{{sint}}{\left(\mathrm{2}+{xsint}\right)^{\mathrm{2}} }{dt} \\ $$$$\left.\mathrm{3}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{3}}} \:\:\frac{{dt}}{\mathrm{2}+\mathrm{3}{sint}} \\ $$$${and}\:\int_{\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{3}}} \:\:\:\frac{{sint}}{\left(\mathrm{2}+\mathrm{3}{sint}\right)^{\mathrm{2}} }{dt} \\ $$

Question Number 58480 Answers: 0 Comments: 2

Question Number 58479 Answers: 0 Comments: 1

Question Number 58478 Answers: 2 Comments: 1

{1} ∫((x^2 −2)/(x^4 +8x^2 +4)) dx = ? {2} Shortest distance between the parabolas y^2 =4x and y^2 =2x−6 is ?

$$\left\{\mathrm{1}\right\}\:\:\:\int\frac{{x}^{\mathrm{2}} −\mathrm{2}}{{x}^{\mathrm{4}} +\mathrm{8}{x}^{\mathrm{2}} +\mathrm{4}}\:{dx}\:=\:? \\ $$$$\left\{\mathrm{2}\right\}\:\:{Shortest}\:{distance}\:{between}\:{the} \\ $$$${parabolas}\:{y}^{\mathrm{2}} =\mathrm{4}{x}\:{and}\:{y}^{\mathrm{2}} =\mathrm{2}{x}−\mathrm{6}\:{is}\:? \\ $$

Question Number 58467 Answers: 1 Comments: 3

Question Number 58462 Answers: 1 Comments: 0

a, b, c, d ∈ R^+ a + b + c + d = 1 Prove that : abc + bcd + cda + dab ≤ (1/(27)) + ((176)/(27)) abcd

$${a},\:{b},\:{c},\:{d}\:\:\in\:\:\mathbb{R}^{+} \\ $$$${a}\:+\:{b}\:+\:{c}\:+\:{d}\:\:=\:\:\mathrm{1} \\ $$$${Prove}\:\:{that}\:\:: \\ $$$${abc}\:+\:{bcd}\:+\:{cda}\:+\:{dab}\:\:\leqslant\:\:\frac{\mathrm{1}}{\mathrm{27}}\:\:+\:\:\frac{\mathrm{176}}{\mathrm{27}}\:{abcd} \\ $$

Question Number 58454 Answers: 0 Comments: 0

Question Number 58447 Answers: 2 Comments: 1

Question Number 58438 Answers: 1 Comments: 4

lim_(x→∞) (((x−1)/x))^x

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\left(\frac{{x}−\mathrm{1}}{{x}}\right)^{{x}} \\ $$

Question Number 58422 Answers: 1 Comments: 0

Question Number 58406 Answers: 2 Comments: 0

1)Value of 20!+((21!)/(1!))+((22!)/(2!))+....+((60!)/(40!)) is ? 2) Sum of all solutions of eq^n : cos 3θ=sin 2θ in interval [−(π/2),(π/2)] is ?

$$\left.\mathrm{1}\right){Value}\:{of}\:\mathrm{20}!+\frac{\mathrm{21}!}{\mathrm{1}!}+\frac{\mathrm{22}!}{\mathrm{2}!}+....+\frac{\mathrm{60}!}{\mathrm{40}!}\:{is}\:\:? \\ $$$$\left.\mathrm{2}\right)\:{Sum}\:{of}\:{all}\:{solutions}\:{of}\:{eq}^{{n}} \:: \\ $$$$\mathrm{cos}\:\mathrm{3}\theta=\mathrm{sin}\:\mathrm{2}\theta\:{in}\:{interval}\:\left[−\frac{\pi}{\mathrm{2}},\frac{\pi}{\mathrm{2}}\right]\:{is}\:? \\ $$

Question Number 58405 Answers: 3 Comments: 2

1) lim_(x→0) ((e^(ax) −bx−1)/x^2 )=2 . find a,b ? 2) 6 balls are placed randomly into 6 cells. Then the probability that exactly one cell remains empty is ?

$$\left.\mathrm{1}\right)\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{e}^{{ax}} −{bx}−\mathrm{1}}{{x}^{\mathrm{2}} }=\mathrm{2}\:. \\ $$$${find}\:{a},{b}\:? \\ $$$$ \\ $$$$\left.\mathrm{2}\right)\:\mathrm{6}\:{balls}\:{are}\:{placed}\:{randomly}\:{into} \\ $$$$\mathrm{6}\:{cells}.\:{Then}\:{the}\:{probability}\:{that}\:{exactly} \\ $$$${one}\:{cell}\:{remains}\:{empty}\:{is}\:? \\ $$

Question Number 58409 Answers: 0 Comments: 3

Prove without mathematical induction that the expression (1 + (√2))^(2n) + (1 − (√2))^(2n) is even for every natural number n.

$$\mathrm{Prove}\:\mathrm{without}\:\mathrm{mathematical}\:\mathrm{induction}\:\mathrm{that}\:\mathrm{the}\: \\ $$$$\mathrm{expression}\:\:\:\left(\mathrm{1}\:+\:\sqrt{\mathrm{2}}\right)^{\mathrm{2n}} \:+\:\left(\mathrm{1}\:−\:\sqrt{\mathrm{2}}\right)^{\mathrm{2n}} \:\:\mathrm{is}\:\mathrm{even}\:\mathrm{for}\:\mathrm{every} \\ $$$$\mathrm{natural}\:\mathrm{number}\:\:\mathrm{n}. \\ $$

Question Number 58402 Answers: 2 Comments: 2

The imaginary part of ((1/2)+(1/2)i)^(10) is ?

$${The}\:{imaginary}\:{part}\:{of}\:\left(\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}{i}\right)^{\mathrm{10}} {is}\:? \\ $$

Question Number 58400 Answers: 1 Comments: 1

If parabola y=−x^2 −2x+k touches the parabola y=−(1/2)x^2 −4x+3 , then the value of k is ? a) 1 b)2 c)3 d)4

$${If}\:{parabola}\:{y}=−{x}^{\mathrm{2}} −\mathrm{2}{x}+{k}\:{touches} \\ $$$${the}\:{parabola}\:{y}=−\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{2}} −\mathrm{4}{x}+\mathrm{3}\:,\:{then} \\ $$$${the}\:{value}\:{of}\:{k}\:{is}\:? \\ $$$$\left.{a}\left.\right)\left.\:\left.\mathrm{1}\:\:\:\:{b}\right)\mathrm{2}\:\:\:\:\:{c}\right)\mathrm{3}\:\:\:\:\:{d}\right)\mathrm{4} \\ $$

Question Number 58410 Answers: 1 Comments: 0

Show that the sum of the cube of three consecutive number gives a multiple of 9.

$$\mathrm{Show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{cube}\:\mathrm{of}\:\mathrm{three}\:\mathrm{consecutive} \\ $$$$\mathrm{number}\:\mathrm{gives}\:\mathrm{a}\:\mathrm{multiple}\:\mathrm{of}\:\:\mathrm{9}. \\ $$

Question Number 58526 Answers: 0 Comments: 1

Question Number 58390 Answers: 2 Comments: 2

write without roots in denominator if possible (1) (1/(√a)) (2) (1/((√a)+(√b))) (3) (1/((√a)+(√b)+(√c))) (4) (1/((√a)+(√b)+(√c)+(√d))) (5) (1/((√a)+(√b)+(√c)+(√d)+(√e)))

$$\mathrm{write}\:\mathrm{without}\:\mathrm{roots}\:\mathrm{in}\:\mathrm{denominator}\:\mathrm{if}\:\mathrm{possible} \\ $$$$\left(\mathrm{1}\right)\:\frac{\mathrm{1}}{\sqrt{{a}}} \\ $$$$\left(\mathrm{2}\right)\:\frac{\mathrm{1}}{\sqrt{{a}}+\sqrt{{b}}} \\ $$$$\left(\mathrm{3}\right)\:\frac{\mathrm{1}}{\sqrt{{a}}+\sqrt{{b}}+\sqrt{{c}}} \\ $$$$\left(\mathrm{4}\right)\:\frac{\mathrm{1}}{\sqrt{{a}}+\sqrt{{b}}+\sqrt{{c}}+\sqrt{{d}}} \\ $$$$\left(\mathrm{5}\right)\:\frac{\mathrm{1}}{\sqrt{{a}}+\sqrt{{b}}+\sqrt{{c}}+\sqrt{{d}}+\sqrt{{e}}} \\ $$

Pg 1504 Pg 1505 Pg 1506 Pg 1507 Pg 1508 Pg 1509 Pg 1510 Pg 1511 Pg 1512 Pg 1513

Contact: info@tinkutara.com