
AllQuestion and Answers: Page 1488
Question Number 60854 Answers: 2 Comments: 4
Question Number 60853 Answers: 2 Comments: 0
$$\sqrt{\mathrm{5}−\mathrm{12}{i}}+\sqrt{\mathrm{5}+\mathrm{12}{i}}=? \\ $$
Question Number 60849 Answers: 1 Comments: 5
Question Number 61612 Answers: 0 Comments: 7
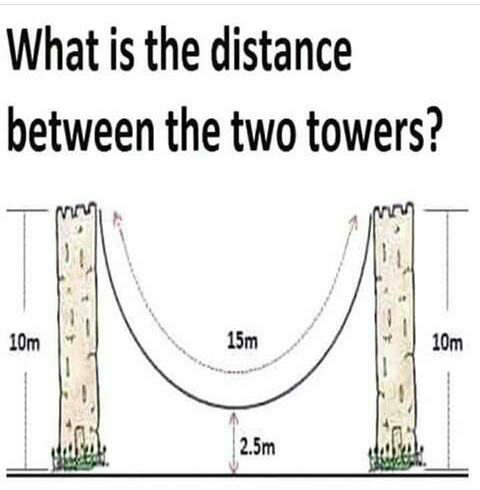
Question Number 60817 Answers: 4 Comments: 2
Question Number 60816 Answers: 1 Comments: 0
Question Number 60814 Answers: 1 Comments: 0
Question Number 60812 Answers: 0 Comments: 5

Question Number 60797 Answers: 0 Comments: 2
$$\int\frac{{e}^{{w}} }{{w}^{{n}+\mathrm{1}} }{dw},\:{n}\in\mathbb{N} \\ $$
Question Number 60791 Answers: 1 Comments: 2
$$\int\frac{{e}^{{n}} }{{x}^{{n}+\mathrm{1}} }{dx},\:\mathrm{n}\in\mathbb{N} \\ $$
Question Number 60788 Answers: 2 Comments: 1
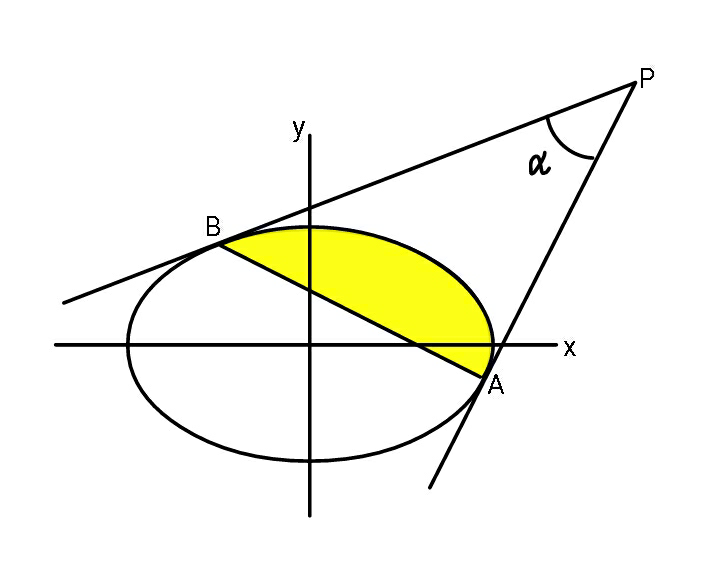
Question Number 60783 Answers: 0 Comments: 2
Question Number 60775 Answers: 0 Comments: 1
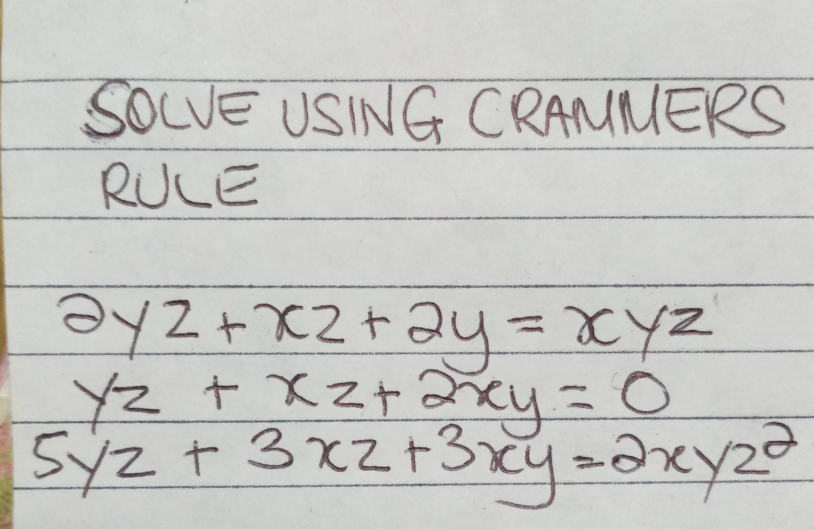
Question Number 60765 Answers: 0 Comments: 2
Question Number 60756 Answers: 1 Comments: 1
Question Number 60748 Answers: 1 Comments: 1

Question Number 60745 Answers: 4 Comments: 5

Question Number 60739 Answers: 1 Comments: 4
Question Number 60735 Answers: 3 Comments: 0
Question Number 60734 Answers: 1 Comments: 4
Question Number 60731 Answers: 0 Comments: 0
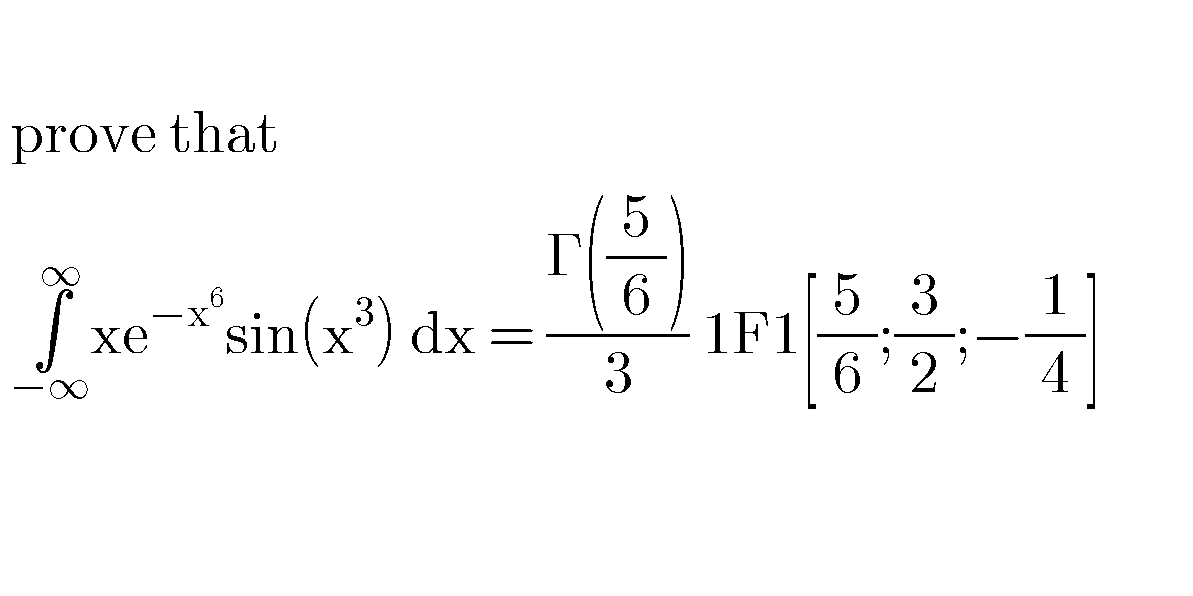
Question Number 60728 Answers: 0 Comments: 0
Question Number 60727 Answers: 0 Comments: 2
Question Number 60725 Answers: 0 Comments: 2
Question Number 60723 Answers: 0 Comments: 10
Question Number 60717 Answers: 0 Comments: 1

Pg 1483 Pg 1484 Pg 1485 Pg 1486 Pg 1487 Pg 1488 Pg 1489 Pg 1490 Pg 1491 Pg 1492
