
AllQuestion and Answers: Page 1487
Question Number 61202 Answers: 1 Comments: 1

Question Number 61205 Answers: 0 Comments: 4
Question Number 60987 Answers: 1 Comments: 1
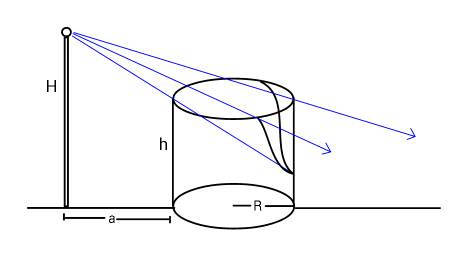
Question Number 60984 Answers: 2 Comments: 9
Question Number 60981 Answers: 2 Comments: 6
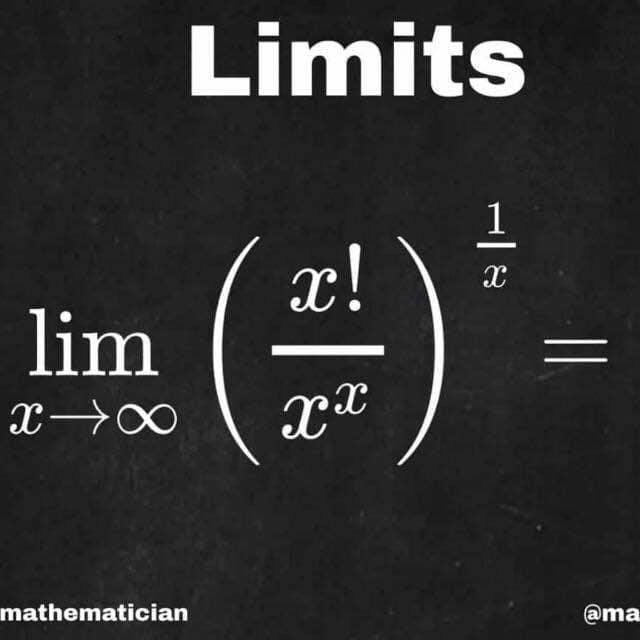
Question Number 60976 Answers: 0 Comments: 1
Question Number 60980 Answers: 1 Comments: 2
Question Number 60967 Answers: 0 Comments: 4
Question Number 60960 Answers: 0 Comments: 2
Question Number 60955 Answers: 1 Comments: 4

Question Number 60948 Answers: 0 Comments: 1
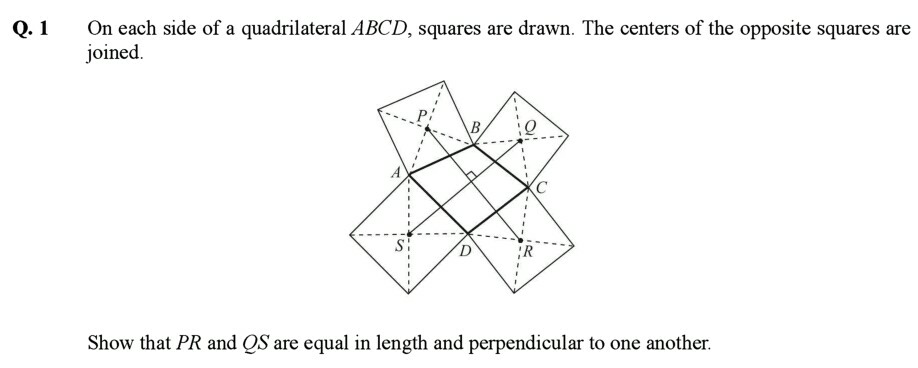
Question Number 60946 Answers: 3 Comments: 4
$$\mathrm{Find}\:\mathrm{x}:\:\:\:\:\:\:\mathrm{x}^{\mathrm{x}} \:\:=\:\:\mathrm{2x} \\ $$
Question Number 60944 Answers: 1 Comments: 1
Question Number 60938 Answers: 0 Comments: 1
Question Number 60921 Answers: 1 Comments: 0
Question Number 60915 Answers: 0 Comments: 0
Question Number 60906 Answers: 0 Comments: 1

Question Number 60905 Answers: 3 Comments: 0
Question Number 60901 Answers: 1 Comments: 0
$${find}\:\int\:\:{arctan}\left(\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\right){dx}\: \\ $$
Question Number 60894 Answers: 0 Comments: 0
Question Number 60893 Answers: 1 Comments: 1
Question Number 60910 Answers: 1 Comments: 7
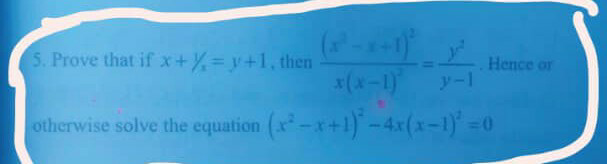
Question Number 60888 Answers: 1 Comments: 1
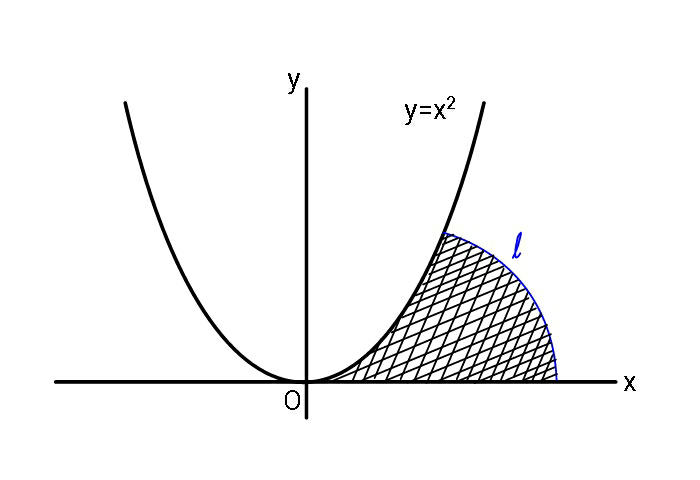
Question Number 60873 Answers: 2 Comments: 5
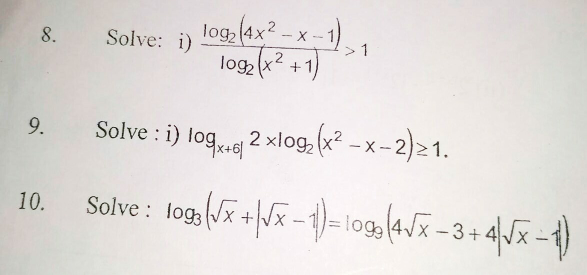
Question Number 60881 Answers: 0 Comments: 3
Question Number 60862 Answers: 0 Comments: 0

Pg 1482 Pg 1483 Pg 1484 Pg 1485 Pg 1486 Pg 1487 Pg 1488 Pg 1489 Pg 1490 Pg 1491
