Question and Answers Forum

AllQuestion and Answers: Page 1486

Question Number 61750 Answers: 0 Comments: 0

find ∫ ((x^2 −(√(x−1)))/(2(√(x^2 +3)))) dx

$$\:{find}\:\int\:\:\frac{{x}^{\mathrm{2}} −\sqrt{{x}−\mathrm{1}}}{\mathrm{2}\sqrt{{x}^{\mathrm{2}} +\mathrm{3}}}\:{dx} \\ $$

Question Number 61749 Answers: 0 Comments: 0

find ∫ (dx/(cos(2x)+tan(x)))

$${find}\:\int\:\:\frac{{dx}}{{cos}\left(\mathrm{2}{x}\right)+{tan}\left({x}\right)} \\ $$

Question Number 61744 Answers: 0 Comments: 7

3xy^2 +x^3 =9 −−−−−(1) 3x^2 y+y^3 =18−−−−(2) Find x and y

$$\mathrm{3}{xy}^{\mathrm{2}} +{x}^{\mathrm{3}} =\mathrm{9}\:−−−−−\left(\mathrm{1}\right) \\ $$$$\mathrm{3}{x}^{\mathrm{2}} {y}+{y}^{\mathrm{3}} =\mathrm{18}−−−−\left(\mathrm{2}\right) \\ $$$${Find}\:{x}\:{and}\:{y} \\ $$

Question Number 61738 Answers: 2 Comments: 0

Question Number 61735 Answers: 0 Comments: 0

∫_(−1) ^1 (((sin(x))/(sinh^(−1) (x))))(((sin^(−1) (x))/(sinh(x)))) dx =?

$$\underset{−\mathrm{1}} {\overset{\mathrm{1}} {\int}}\left(\frac{{sin}\left({x}\right)}{{sinh}^{−\mathrm{1}} \left({x}\right)}\right)\left(\frac{{sin}^{−\mathrm{1}} \left({x}\right)}{{sinh}\left({x}\right)}\right)\:{dx}\:=?\: \\ $$$$ \\ $$

Question Number 61733 Answers: 0 Comments: 1

Find the maximum volume tetrahedron inside an ellipsoid, parameters a,b,c .

$${Find}\:{the}\:{maximum} \\ $$$${volume}\:{tetrahedron}\:{inside}\:{an} \\ $$$${ellipsoid},\:{parameters}\:\boldsymbol{{a}},\boldsymbol{{b}},\boldsymbol{{c}}\:. \\ $$

Question Number 61721 Answers: 0 Comments: 0

calculate A =∫_0 ^∞ cos(x^n )dx and B =∫_0 ^∞ sin(x^n )dx with n≥2 (n integr natural)

$${calculate}\:{A}\:=\int_{\mathrm{0}} ^{\infty} \:{cos}\left({x}^{{n}} \right){dx} \\ $$$${and}\:{B}\:=\int_{\mathrm{0}} ^{\infty} \:{sin}\left({x}^{{n}} \right){dx}\:\:{with} \\ $$$${n}\geqslant\mathrm{2}\:\:\left({n}\:{integr}\:{natural}\right) \\ $$

Question Number 61719 Answers: 0 Comments: 1

∫(dx/(2+sin(x)))

$$\int\frac{{dx}}{\mathrm{2}+{sin}\left({x}\right)} \\ $$

Question Number 61708 Answers: 0 Comments: 1

8+(4+3×2)

$$\mathrm{8}+\left(\mathrm{4}+\mathrm{3}×\mathrm{2}\right) \\ $$

Question Number 61707 Answers: 0 Comments: 1

6^(−3)

$$\mathrm{6}^{−\mathrm{3}} \\ $$

Question Number 61706 Answers: 0 Comments: 1

(7^(10) /7^7 )

$$\frac{\mathrm{7}^{\mathrm{10}} }{\mathrm{7}^{\mathrm{7}} } \\ $$

Question Number 61694 Answers: 1 Comments: 1

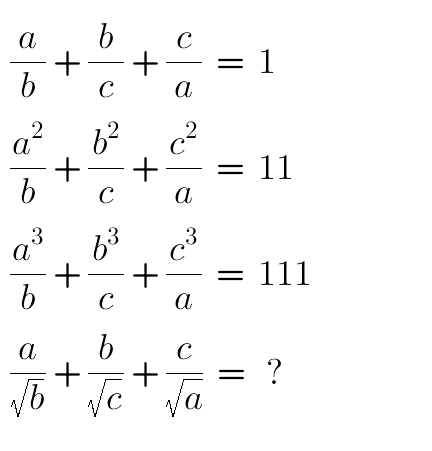
Question Number 61692 Answers: 0 Comments: 0

if(3/2)≤x≤5, prove:2(√(x+1))+(√(2x−3))+(√(15−3x))<2(√(19))

$${if}\frac{\mathrm{3}}{\mathrm{2}}\leqslant{x}\leqslant\mathrm{5}, \\ $$$${prove}:\mathrm{2}\sqrt{{x}+\mathrm{1}}+\sqrt{\mathrm{2}{x}−\mathrm{3}}+\sqrt{\mathrm{15}−\mathrm{3}{x}}<\mathrm{2}\sqrt{\mathrm{19}} \\ $$

Question Number 61691 Answers: 0 Comments: 0

f(x)=(√(x^4 −3x^2 +4))+(√(x^4 −3x^2 −8x+20)) find the minimum value of f(x)

$${f}\left({x}\right)=\sqrt{{x}^{\mathrm{4}} −\mathrm{3}{x}^{\mathrm{2}} +\mathrm{4}}+\sqrt{{x}^{\mathrm{4}} −\mathrm{3}{x}^{\mathrm{2}} −\mathrm{8}{x}+\mathrm{20}} \\ $$$${find}\:{the}\:{minimum}\:{value}\:{of}\:{f}\left({x}\right) \\ $$

Question Number 61700 Answers: 1 Comments: 0

Question Number 61724 Answers: 0 Comments: 6

Σ_(n≥0) n^2 x^n

$$\underset{{n}\geqslant\mathrm{0}} {\sum}{n}^{\mathrm{2}} {x}^{{n}} \\ $$$$ \\ $$

Question Number 61662 Answers: 0 Comments: 1

calculate ∫_(−(π/4)) ^(π/4) ((cosx)/(e^(1/x) +1)) dx

$${calculate}\:\int_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{{cosx}}{{e}^{\frac{\mathrm{1}}{{x}}} \:+\mathrm{1}}\:{dx}\: \\ $$

Question Number 61661 Answers: 0 Comments: 1

1) calculate ∫∫_R^+^2 ((dxdy)/((1+x^2 )(1+y^2 ))) 2) find the value of ∫_0 ^∞ ((ln(x))/(x^2 −1)) dx .

$$\left.\mathrm{1}\right)\:{calculate}\:\int\int_{{R}^{+^{\mathrm{2}} } } \:\:\:\:\:\frac{{dxdy}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left(\mathrm{1}+{y}^{\mathrm{2}} \right)} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\infty} \:\frac{{ln}\left({x}\right)}{{x}^{\mathrm{2}} −\mathrm{1}}\:{dx}\:. \\ $$

Question Number 61660 Answers: 0 Comments: 1

let U_n = ∫_0 ^∞ (dt/((1+t^3 )^n )) dt (n≥1) 1) calculate (U_(n+1) /U_n ) 2) study the serie Σln((U_(n+1) /U_n )) and prove that lim_(n→+∞) U_n =0

$${let}\:{U}_{{n}} =\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{dt}}{\left(\mathrm{1}+{t}^{\mathrm{3}} \right)^{{n}} }\:{dt}\:\:\:\:\left({n}\geqslant\mathrm{1}\right) \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:\frac{{U}_{{n}+\mathrm{1}} }{{U}_{{n}} } \\ $$$$\left.\mathrm{2}\right)\:{study}\:{the}\:{serie}\:\Sigma{ln}\left(\frac{{U}_{{n}+\mathrm{1}} }{{U}_{{n}} }\right)\:\:{and}\:{prove}\:\:{that}\:{lim}_{{n}\rightarrow+\infty} {U}_{{n}} =\mathrm{0} \\ $$

Question Number 61657 Answers: 0 Comments: 0

U_n and V_n are two sequences verify U_n =Σ_(k=0) ^n C_n ^k V_k determine V_n interms of U_k ,0≤k≤n

$${U}_{{n}} \:{and}\:{V}_{{n}} \:\:{are}\:{two}\:{sequences}\:\:{verify}\:\:{U}_{{n}} =\sum_{{k}=\mathrm{0}} ^{{n}} \:{C}_{{n}} ^{{k}} \:{V}_{{k}} \\ $$$${determine}\:{V}_{{n}} \:\:{interms}\:{of}\:\:{U}_{{k}} \:\:\:\:\:\:\:,\mathrm{0}\leqslant{k}\leqslant{n} \\ $$

Question Number 61675 Answers: 1 Comments: 1

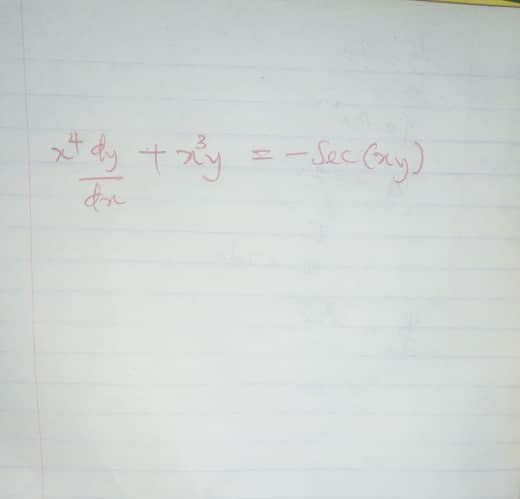
Question Number 61674 Answers: 1 Comments: 4

a.∫_( 0) ^( (𝛑/4)) (√(1+tgx)) dx=? b.∫_( 0) ^( 1) (√(1+lnx)) dx=?

$$\mathrm{a}.\underset{\:\:\:\mathrm{0}} {\overset{\:\:\:\:\:\:\:\frac{\boldsymbol{\pi}}{\mathrm{4}}} {\int}}\sqrt{\mathrm{1}+\boldsymbol{\mathrm{tgx}}}\:\boldsymbol{\mathrm{dx}}=? \\ $$$$\mathrm{b}.\underset{\:\:\mathrm{0}} {\overset{\:\:\:\:\:\:\:\:\mathrm{1}} {\int}}\sqrt{\mathrm{1}+\boldsymbol{\mathrm{lnx}}}\:\boldsymbol{\mathrm{dx}}=? \\ $$

Question Number 61654 Answers: 0 Comments: 0

∫_0 ^∞ e^(−e^x ) ln(x) dx = 0.27634

$$\int_{\mathrm{0}} ^{\infty} {e}^{−{e}^{{x}} } {ln}\left({x}\right)\:{dx}\:=\:\mathrm{0}.\mathrm{27634} \\ $$

Question Number 61646 Answers: 0 Comments: 0

let f(x) =e^(−ax) arctan(3x) with a>0 1) calculate f^((n)) (x) and f^((n)) (0) 2) developp f (x) at integr serie . 3) calculate ∫_0 ^∞ f(x)dx .

$${let}\:{f}\left({x}\right)\:={e}^{−{ax}} \:{arctan}\left(\mathrm{3}{x}\right)\:\:\:{with}\:{a}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}^{\left({n}\right)} \left({x}\right)\:{and}\:{f}^{\left({n}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{2}\right)\:{developp}\:{f}\:\left({x}\right)\:{at}\:{integr}\:{serie}\:. \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\int_{\mathrm{0}} ^{\infty} \:{f}\left({x}\right){dx}\:. \\ $$

Question Number 61645 Answers: 0 Comments: 1

calculate ∫∫_D ∫(√(x^2 +y^2 +z^2 ))dxdydz with D ={(x,y,z) / 0≤x≤1 ,1≤y≤2 , 2≤z≤3 }

$${calculate}\:\int\int_{{D}} \int\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} }{dxdydz} \\ $$$${with}\:{D}\:=\left\{\left({x},{y},{z}\right)\:/\:\mathrm{0}\leqslant{x}\leqslant\mathrm{1}\:\:,\mathrm{1}\leqslant{y}\leqslant\mathrm{2}\:\:,\:\mathrm{2}\leqslant{z}\leqslant\mathrm{3}\:\right\} \\ $$

Question Number 61652 Answers: 1 Comments: 1

solve inside C z^4 =((1−i)/(1+i(√3)))

$${solve}\:{inside}\:{C}\:\:{z}^{\mathrm{4}} \:=\frac{\mathrm{1}−{i}}{\mathrm{1}+{i}\sqrt{\mathrm{3}}} \\ $$

Pg 1481 Pg 1482 Pg 1483 Pg 1484 Pg 1485 Pg 1486 Pg 1487 Pg 1488 Pg 1489 Pg 1490

Contact: info@tinkutara.com