
AllQuestion and Answers: Page 1485
Question Number 61297 Answers: 0 Comments: 0
Question Number 61284 Answers: 0 Comments: 0
Question Number 61283 Answers: 1 Comments: 0
Question Number 61274 Answers: 1 Comments: 0
Question Number 61272 Answers: 0 Comments: 0

Question Number 61273 Answers: 2 Comments: 2
Question Number 61270 Answers: 2 Comments: 0

Question Number 61269 Answers: 3 Comments: 0
Question Number 61268 Answers: 0 Comments: 0
Question Number 61267 Answers: 0 Comments: 0
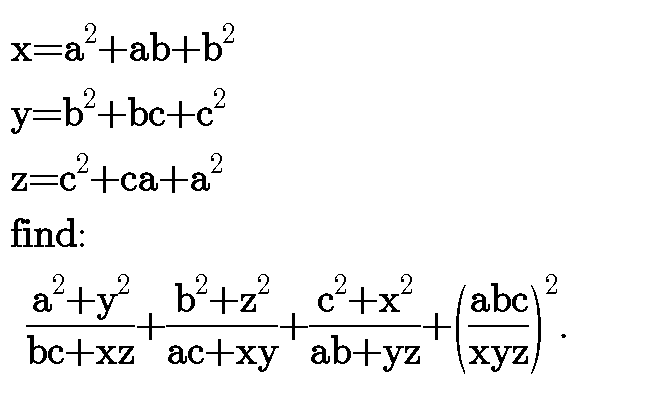
Question Number 61261 Answers: 1 Comments: 0
Question Number 61258 Answers: 1 Comments: 2
Question Number 61241 Answers: 5 Comments: 0

Question Number 61240 Answers: 1 Comments: 3
Question Number 61237 Answers: 0 Comments: 0

Question Number 61235 Answers: 0 Comments: 6

Question Number 61232 Answers: 0 Comments: 3
Question Number 61229 Answers: 1 Comments: 0
Question Number 61215 Answers: 2 Comments: 0
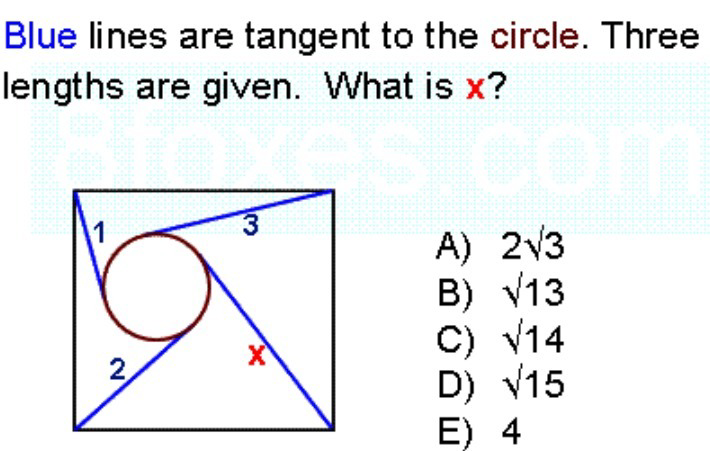
Question Number 61208 Answers: 0 Comments: 8
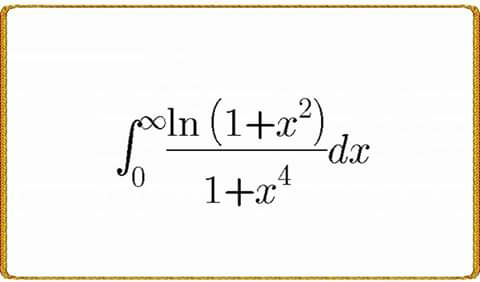
Question Number 61211 Answers: 1 Comments: 3
Question Number 61210 Answers: 2 Comments: 1
$${for}\:{what}\:{value}\:{of}\:\theta,\:\:{e}^{{i}\theta} =\mathrm{0}\:\: \\ $$
Question Number 61180 Answers: 1 Comments: 0
$${solve}\:{y}^{''} \:+\mathrm{3}{y}^{'} −{y}\:={sin}\left(\mathrm{2}{x}\right) \\ $$
Question Number 61181 Answers: 0 Comments: 0
Question Number 61169 Answers: 1 Comments: 1

Question Number 61165 Answers: 1 Comments: 0

Pg 1480 Pg 1481 Pg 1482 Pg 1483 Pg 1484 Pg 1485 Pg 1486 Pg 1487 Pg 1488 Pg 1489
