Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1477

Question Number 62133 Answers: 0 Comments: 0

5(2+3+1)

$$\mathrm{5}\left(\mathrm{2}+\mathrm{3}+\mathrm{1}\right) \\ $$

Question Number 62132 Answers: 0 Comments: 0

(3×2)^2

$$\left(\mathrm{3}×\mathrm{2}\right)^{\mathrm{2}} \\ $$

Question Number 62131 Answers: 0 Comments: 0

(1/3)+3(1/4)

$$\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{3}\frac{\mathrm{1}}{\mathrm{4}} \\ $$

Question Number 62130 Answers: 1 Comments: 0

(√(α^2 −β^2 )) simplify

$$\sqrt{\alpha^{\mathrm{2}} −\beta^{\mathrm{2}} } \\ $$$${simplify}\: \\ $$$$ \\ $$

Question Number 62129 Answers: 0 Comments: 4

let f(x)=ln(x+1−2(√x)) 1) find D_f 2) determine f^(−1) 3) calculate ∫f (x)dx and ∫ f^(−1) (x)dx

$${let}\:{f}\left({x}\right)={ln}\left({x}+\mathrm{1}−\mathrm{2}\sqrt{{x}}\right) \\ $$$$\left.\mathrm{1}\right)\:{find}\:{D}_{{f}} \\ $$$$\left.\mathrm{2}\right)\:{determine}\:{f}^{−\mathrm{1}} \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\:\int{f}\:\left({x}\right){dx}\:{and}\:\:\int\:{f}^{−\mathrm{1}} \left({x}\right){dx} \\ $$

Question Number 62128 Answers: 0 Comments: 3

let U_n = ∫_0 ^∞ ((cos(nx))/((x^2 +n^2 )^3 ))dx with n≥1 1) calculate U_n intrems of n 2) find lim_(n→+∞) n U_n 3) calculate lim_(n→+∞) n^2 U_n 4) study the convervence of U_n

$${let}\:{U}_{{n}} =\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({nx}\right)}{\left({x}^{\mathrm{2}} \:+{n}^{\mathrm{2}} \right)^{\mathrm{3}} }{dx}\:\:{with}\:{n}\geqslant\mathrm{1} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{U}_{{n}} \:{intrems}\:{of}\:{n} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{lim}_{{n}\rightarrow+\infty} {n}\:{U}_{{n}} \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:{lim}_{{n}\rightarrow+\infty} {n}^{\mathrm{2}} \:{U}_{{n}} \\ $$$$\left.\mathrm{4}\right)\:{study}\:{the}\:{convervence}\:{of}\:{U}_{{n}} \\ $$

Question Number 62124 Answers: 0 Comments: 0

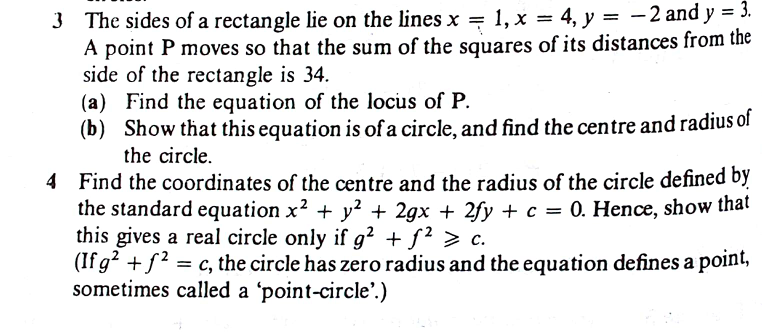
Question Number 62122 Answers: 0 Comments: 4

∫e^(cos(x)) sin(sin(x)) dx

$$\int{e}^{{cos}\left({x}\right)} {sin}\left({sin}\left({x}\right)\right)\:{dx}\: \\ $$

Question Number 62121 Answers: 2 Comments: 0

A cube of unit edge length is held before a plane. Prove that the sum of the squares of the projected lengths of edges of the cube on the plane (irrespective of the orientation of the cube) is 8.

$${A}\:{cube}\:{of}\:{unit}\:{edge}\:{length}\: \\ $$$${is}\:{held}\:{before}\:{a}\:{plane}.\:{Prove}\:{that} \\ $$$${the}\:{sum}\:{of}\:{the}\:{squares}\:{of}\:{the} \\ $$$${projected}\:{lengths}\:{of}\:{edges}\:{of}\:{the}\:{cube} \\ $$$${on}\:{the}\:{plane}\:\left({irrespective}\:{of}\right. \\ $$$$\left.{the}\:{orientation}\:{of}\:{the}\:{cube}\right)\:{is}\:\mathrm{8}. \\ $$

Question Number 62112 Answers: 2 Comments: 0

Question Number 62109 Answers: 1 Comments: 2

Given that (1+(√(1+x)))tan x=(1+(√(1−x))). Then find sin 4x.

$${Given}\:{that} \\ $$$$\left(\mathrm{1}+\sqrt{\mathrm{1}+{x}}\right)\mathrm{tan}\:{x}=\left(\mathrm{1}+\sqrt{\mathrm{1}−{x}}\right). \\ $$$${Then}\:{find}\:\:\:\mathrm{sin}\:\mathrm{4}{x}. \\ $$

Question Number 62102 Answers: 1 Comments: 0

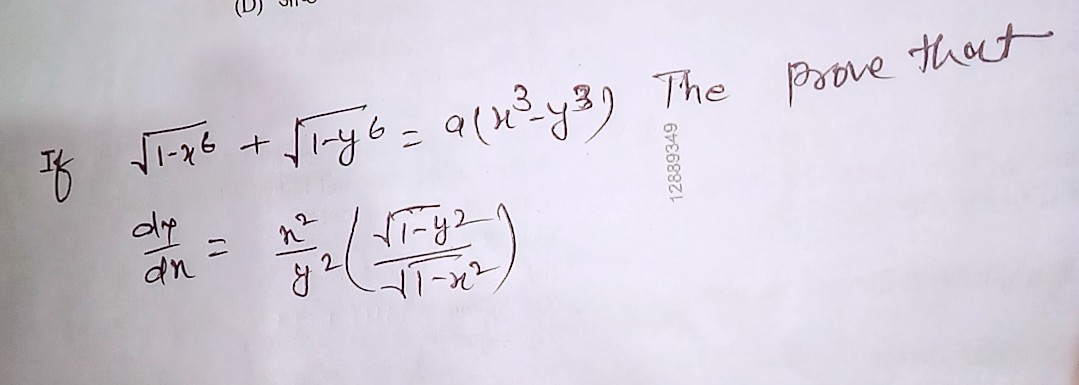
Question Number 62096 Answers: 0 Comments: 0

Question Number 62094 Answers: 0 Comments: 2

Question Number 62093 Answers: 0 Comments: 1

∫ (dx/(sin^3 x + cos^3 x)) = p

$$\int\:\:\frac{{dx}}{\mathrm{sin}^{\mathrm{3}} \:{x}\:+\:\mathrm{cos}^{\mathrm{3}} \:{x}}\:\:=\:\:{p} \\ $$

Question Number 62092 Answers: 0 Comments: 1

MATH MEME: 3+x = 1+8 :) ((3+x)/+) = ((1+8)/+) cancel the plus sign 3x = 18 ((3x)/3) = ((18)/3) x = 6 am i correct?

$$\mathrm{MATH}\:\mathrm{MEME}: \\ $$$$\mathrm{3}+\mathrm{x}\:=\:\mathrm{1}+\mathrm{8} \\ $$$$\left.:\right) \\ $$$$\frac{\mathrm{3}+\mathrm{x}}{+}\:=\:\frac{\mathrm{1}+\mathrm{8}}{+} \\ $$$${cancel}\:{the}\:{plus}\:{sign} \\ $$$$\mathrm{3}{x}\:=\:\mathrm{18} \\ $$$$\frac{\mathrm{3}{x}}{\mathrm{3}}\:=\:\frac{\mathrm{18}}{\mathrm{3}} \\ $$$$\boldsymbol{{x}}\:=\:\mathrm{6} \\ $$$$\boldsymbol{{am}}\:\boldsymbol{{i}}\:\boldsymbol{{correct}}? \\ $$

Question Number 62088 Answers: 0 Comments: 3

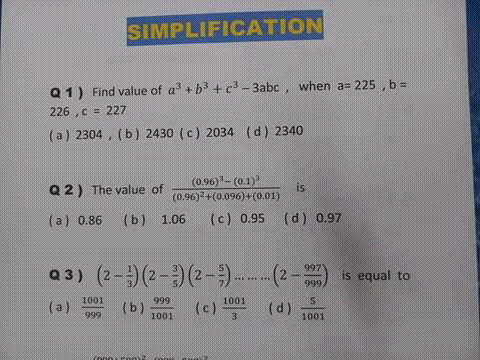
Question Number 62086 Answers: 1 Comments: 0

The coefficient of x^m in (1+x)^p +(1+x)^(p+1) +...+(1+x)^n , p≤ m≤ n is

$$\mathrm{The}\:\mathrm{coefficient}\:\mathrm{of}\:{x}^{{m}} \:\mathrm{in} \\ $$$$\left(\mathrm{1}+{x}\right)^{{p}} +\left(\mathrm{1}+{x}\right)^{{p}+\mathrm{1}} +...+\left(\mathrm{1}+{x}\right)^{{n}} ,\:{p}\leqslant\:{m}\leqslant\:{n} \\ $$$$\mathrm{is}\: \\ $$

Question Number 62081 Answers: 0 Comments: 0

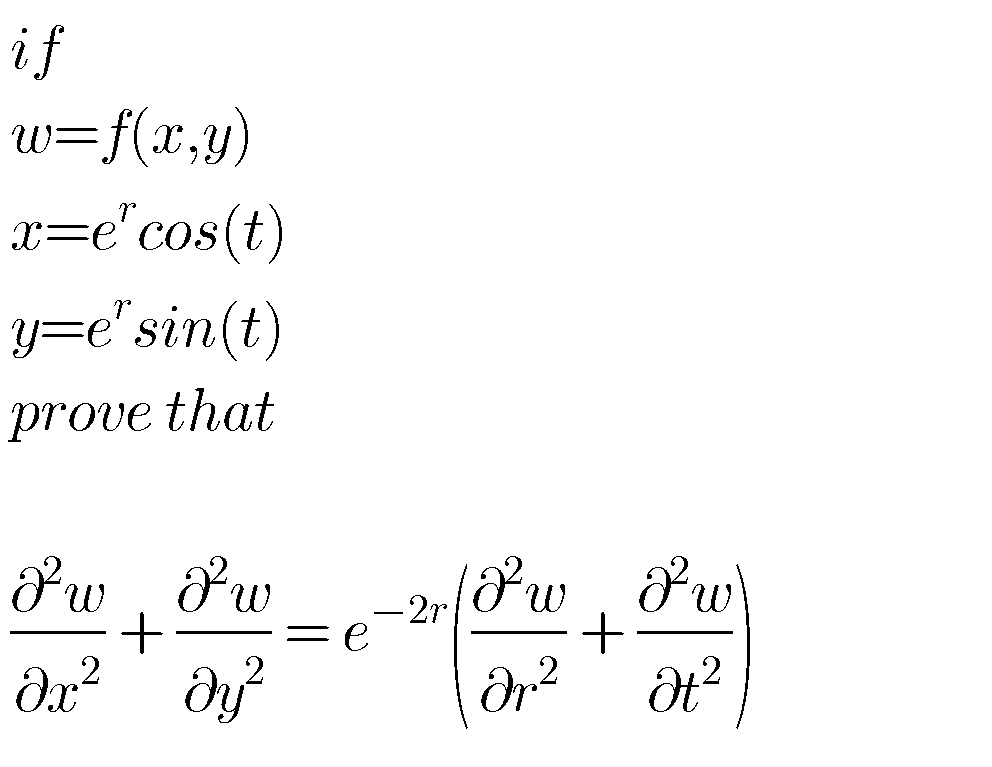
Question Number 62077 Answers: 1 Comments: 0

∫_( 1) ^( ∞) (((ln x)/x))^(2018) dx = ? Any trick(s) to solve it ?

$$\underset{\:\:\mathrm{1}} {\overset{\:\:\:\:\:\:\:\:\:\:\:\:\infty} {\int}}\:\left(\frac{\mathrm{ln}\:{x}}{{x}}\right)^{\mathrm{2018}} \:{dx}\:\:=\:\:? \\ $$$${Any}\:\:{trick}\left({s}\right)\:\:{to}\:\:{solve}\:\:{it}\:? \\ $$

Question Number 62067 Answers: 2 Comments: 3

lim_(x → −1) ((x^3 + 2x^2 − 1)/((x + 1)^2 )) = ?

$$\underset{{x}\:\rightarrow\:−\mathrm{1}} {\mathrm{lim}}\:\:\frac{{x}^{\mathrm{3}} \:+\:\mathrm{2}{x}^{\mathrm{2}} \:−\:\mathrm{1}}{\left({x}\:+\:\mathrm{1}\right)^{\mathrm{2}} }\:\:=\:\:? \\ $$

Question Number 62057 Answers: 1 Comments: 2

2^(−2)

$$\mathrm{2}^{−\mathrm{2}} \\ $$

Question Number 62056 Answers: 0 Comments: 0

1) calculate I =∫ln(1+ix^2 )dx and J = ∫ln(1−ix^2 )dx 2) calculate A =∫ ln(1+x^4 )dx .

$$\left.\mathrm{1}\right)\:{calculate}\:{I}\:=\int{ln}\left(\mathrm{1}+{ix}^{\mathrm{2}} \right){dx}\:{and}\:{J}\:=\:\int{ln}\left(\mathrm{1}−{ix}^{\mathrm{2}} \right){dx} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:{A}\:=\int\:{ln}\left(\mathrm{1}+{x}^{\mathrm{4}} \right){dx}\:. \\ $$

Question Number 62055 Answers: 0 Comments: 0

$8+$3

$$\$\mathrm{8}+\$\mathrm{3} \\ $$

Question Number 62054 Answers: 0 Comments: 1

484×27

$$\mathrm{484}×\mathrm{27} \\ $$

Question Number 62053 Answers: 0 Comments: 1

m5=25

$$\mathrm{m5}=\mathrm{25} \\ $$

Pg 1472 Pg 1473 Pg 1474 Pg 1475 Pg 1476 Pg 1477 Pg 1478 Pg 1479 Pg 1480 Pg 1481

Terms of Service

Contact: info@tinkutara.com