
AllQuestion and Answers: Page 1467
Question Number 63588 Answers: 1 Comments: 1
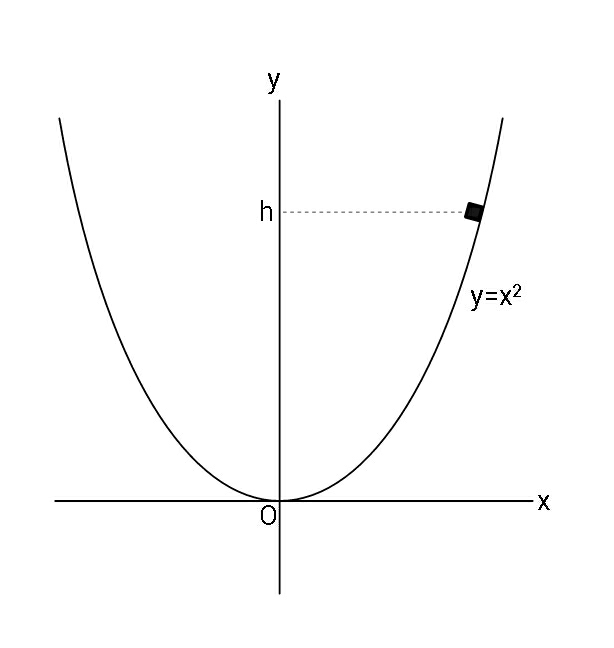
Question Number 63574 Answers: 0 Comments: 12
Question Number 63573 Answers: 0 Comments: 0
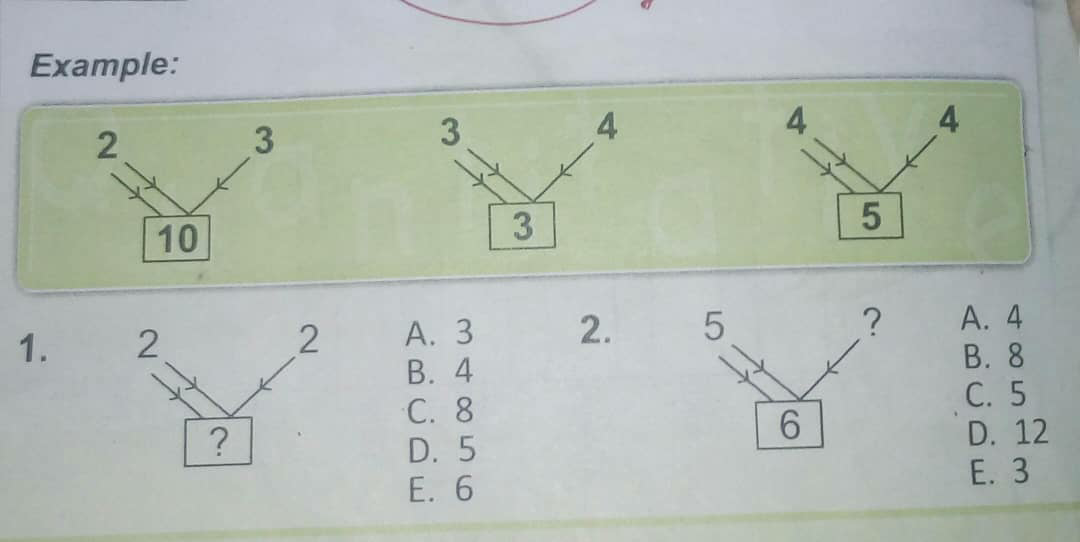
Question Number 63570 Answers: 1 Comments: 3

Question Number 63566 Answers: 0 Comments: 2
Question Number 63565 Answers: 0 Comments: 0
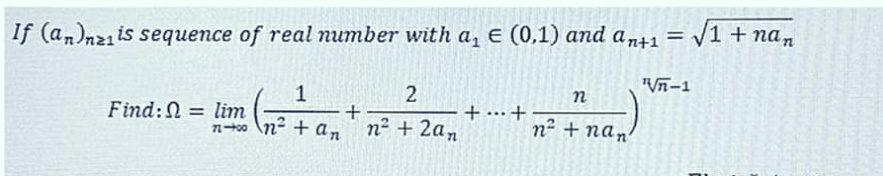
Question Number 63564 Answers: 1 Comments: 0
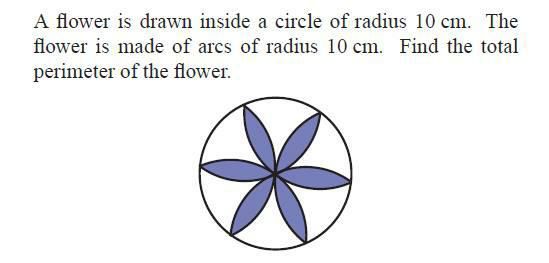
Question Number 63561 Answers: 1 Comments: 0
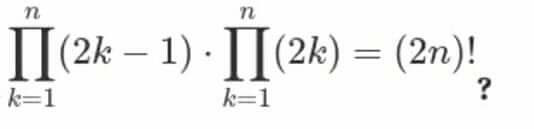
Question Number 63560 Answers: 0 Comments: 2
Question Number 63552 Answers: 1 Comments: 1
Question Number 63539 Answers: 1 Comments: 2
Question Number 63536 Answers: 0 Comments: 1
Question Number 63534 Answers: 1 Comments: 0
Question Number 63532 Answers: 1 Comments: 0
Question Number 63522 Answers: 0 Comments: 2

Question Number 63519 Answers: 0 Comments: 4
Question Number 63517 Answers: 1 Comments: 0
Question Number 63510 Answers: 0 Comments: 1
Question Number 63509 Answers: 1 Comments: 1
Question Number 63508 Answers: 0 Comments: 4
Question Number 63507 Answers: 1 Comments: 0
Question Number 63490 Answers: 2 Comments: 1
Question Number 63489 Answers: 0 Comments: 0
Question Number 63485 Answers: 1 Comments: 0
Question Number 63499 Answers: 1 Comments: 0
$${given}\:{that}\:\:\:{a}\mid{b},\:{show}\:{that}\:−{a}\mid{b}. \\ $$
Question Number 63481 Answers: 1 Comments: 1
Pg 1462 Pg 1463 Pg 1464 Pg 1465 Pg 1466 Pg 1467 Pg 1468 Pg 1469 Pg 1470 Pg 1471
