Question and Answers Forum

AllQuestion and Answers: Page 1459

Question Number 64354 Answers: 1 Comments: 0

some one write the statement a ≡−a(mod m) show that this statement is not generally true.! giving a counter example

$${some}\:{one}\:{write}\:{the}\:{statement} \\ $$$$\:{a}\:\equiv−{a}\left({mod}\:{m}\right)\: \\ $$$${show}\:{that}\:{this}\:{statement}\:{is}\:{not}\:{generally}\:{true}.!\:{giving}\:{a}\:{counter} \\ $$$${example} \\ $$

Question Number 64350 Answers: 0 Comments: 3

Given that f(x) = determinant ((x,x^2 ,x^3 ),(1,(2x),(3x^2 )),(0,2,(6x))), find f ′ (x)

$${Given}\:{that}\:{f}\left({x}\right)\:=\:\begin{vmatrix}{{x}}&{{x}^{\mathrm{2}} }&{{x}^{\mathrm{3}} }\\{\mathrm{1}}&{\mathrm{2}{x}}&{\mathrm{3}{x}^{\mathrm{2}} }\\{\mathrm{0}}&{\mathrm{2}}&{\mathrm{6}{x}}\end{vmatrix},\:{find}\:{f}\:'\:\left({x}\right) \\ $$

Question Number 64348 Answers: 1 Comments: 0

the vectors a and b are such that ∣a∣ =3 , ∣b∣=5 and a.b=−14 find ∣a−b∣

$${the}\:{vectors}\:\boldsymbol{{a}}\:{and}\:\boldsymbol{{b}}\:{are}\:{such}\:{that}\:\mid\boldsymbol{{a}}\mid\:=\mathrm{3}\:,\:\mid\boldsymbol{{b}}\mid=\mathrm{5}\:{and}\:\boldsymbol{{a}}.\boldsymbol{{b}}=−\mathrm{14} \\ $$$${find}\:\mid\boldsymbol{{a}}−\boldsymbol{{b}}\mid \\ $$

Question Number 64347 Answers: 0 Comments: 0

Two consecutive integers between which a root of the equation 1)x^3 +x−16=0 2) x^2 −3x+2=0 lies are;

$${Two}\:{consecutive}\:{integers}\:{between}\:{which}\:{a}\:{root}\:{of}\:{the}\:{equation} \\ $$$$\left.\:\mathrm{1}\right){x}^{\mathrm{3}} +{x}−\mathrm{16}=\mathrm{0}\: \\ $$$$\left.\mathrm{2}\right)\:{x}^{\mathrm{2}} −\mathrm{3}{x}+\mathrm{2}=\mathrm{0} \\ $$$${lies}\:{are}; \\ $$

Question Number 64335 Answers: 1 Comments: 0

∫_( 0) ^π x sin x cos^4 x dx =

$$\underset{\:\mathrm{0}} {\overset{\pi} {\int}}\:\:{x}\:\mathrm{sin}\:{x}\:\mathrm{cos}^{\mathrm{4}} {x}\:{dx}\:= \\ $$

Question Number 64334 Answers: 0 Comments: 1

∫_a ^b ∣ f(x) ∣ dx = 0 ⇒ ∫_a ^b (f(x))^2 dx = 0

$$\underset{{a}} {\overset{{b}} {\int}}\:\:\:\mid\:{f}\left({x}\right)\:\mid\:{dx}\:=\:\mathrm{0}\:\Rightarrow\:\underset{{a}} {\overset{{b}} {\int}}\:\:\left({f}\left({x}\right)\right)^{\mathrm{2}} \:{dx}\:=\:\mathrm{0} \\ $$

Question Number 64333 Answers: 2 Comments: 2

∫_( 0) ^(π/2) (1/(1+tan x)) dx =

$$\underset{\:\mathrm{0}} {\overset{\pi/\mathrm{2}} {\int}}\:\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{tan}\:{x}}\:{dx}\:= \\ $$

Question Number 64332 Answers: 0 Comments: 2

∫_(π/6) ^(π/3) (1/(sin 2x)) dx =

$$\underset{\pi/\mathrm{6}} {\overset{\pi/\mathrm{3}} {\int}}\:\:\frac{\mathrm{1}}{\mathrm{sin}\:\mathrm{2}{x}}\:{dx}\:= \\ $$

Question Number 64331 Answers: 0 Comments: 1

∫_(−1) ^1 sin^(11) x dx =

$$\underset{−\mathrm{1}} {\overset{\mathrm{1}} {\int}}\:\mathrm{sin}^{\mathrm{11}} {x}\:{dx}\:= \\ $$

Question Number 64330 Answers: 0 Comments: 0

If ∫_( 0) ^∞ e^(−x) sin^n x dx = ((24)/(125)), then n=

$$\mathrm{If}\:\underset{\:\mathrm{0}} {\overset{\infty} {\int}}\:{e}^{−{x}} \mathrm{sin}\:^{{n}} {x}\:{dx}\:=\:\frac{\mathrm{24}}{\mathrm{125}},\:\mathrm{then}\:{n}= \\ $$

Question Number 64329 Answers: 0 Comments: 0

If I=∫_( 3) ^4 (1/((log x))^(1/3) ) dx , then

$$\mathrm{If}\:{I}=\underset{\:\mathrm{3}} {\overset{\mathrm{4}} {\int}}\:\frac{\mathrm{1}}{\sqrt[{\mathrm{3}}]{\mathrm{log}\:{x}}}\:{dx}\:,\:\mathrm{then}\: \\ $$

Question Number 64328 Answers: 0 Comments: 0

Let f : R→R, g : R→R be continuous functions. Then ∫_(−π/2) ^(π/2) [ f(x)+f(−x) ] [ g(x)(g(−x) ] dx =

$$\mathrm{Let}\:\:{f}\::\:{R}\rightarrow{R},\:{g}\::\:{R}\rightarrow{R}\:\:\mathrm{be}\:\mathrm{continuous} \\ $$$$\mathrm{functions}.\:\mathrm{Then} \\ $$$$\underset{−\pi/\mathrm{2}} {\overset{\pi/\mathrm{2}} {\int}}\:\left[\:{f}\left({x}\right)+{f}\left(−{x}\right)\:\right]\:\left[\:{g}\left({x}\right)\left({g}\left(−{x}\right)\:\right]\:{dx}\:=\right. \\ $$

Question Number 64327 Answers: 0 Comments: 1

(d/dx) ( ∫_(f(x)) ^(g(x)) φ(t) dt ) =

$$\frac{{d}}{{dx}}\:\:\left(\:\underset{{f}\left({x}\right)} {\overset{{g}\left({x}\right)} {\int}}\:\phi\left({t}\right)\:{dt}\:\right)\:= \\ $$

Question Number 64320 Answers: 1 Comments: 1

without beta function ∫cos^3 t sin^2 t dt

$${without}\:{beta}\:{function} \\ $$$$\int{cos}^{\mathrm{3}} {t}\:{sin}^{\mathrm{2}} {t}\:{dt}\: \\ $$

Question Number 64311 Answers: 3 Comments: 1

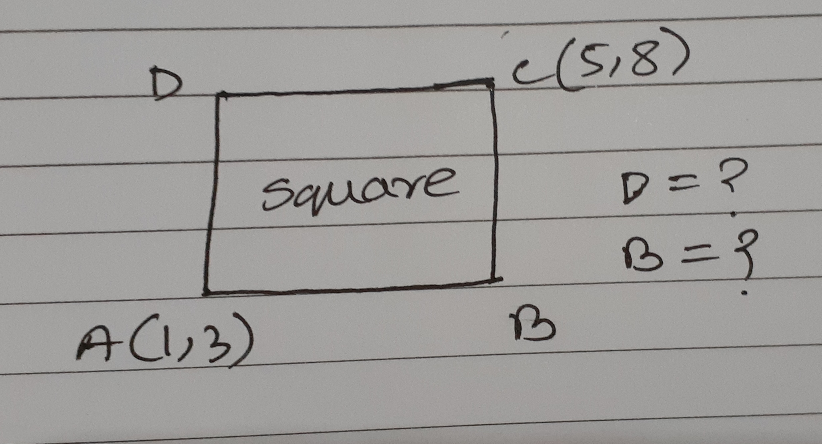
Question Number 64309 Answers: 0 Comments: 1

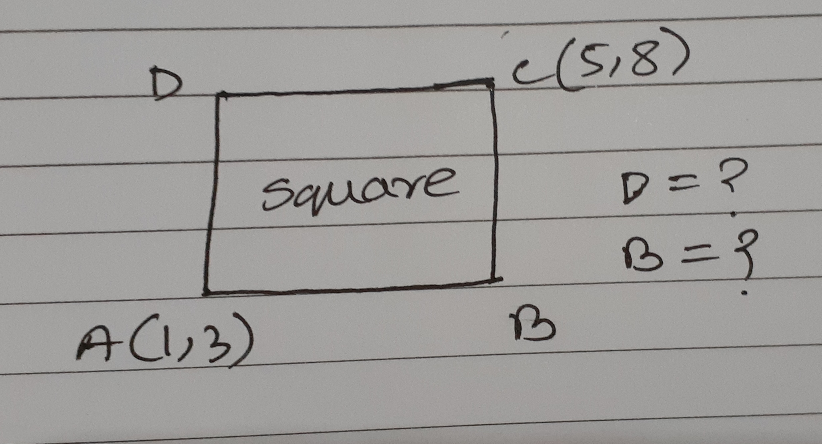
Question Number 64305 Answers: 0 Comments: 6

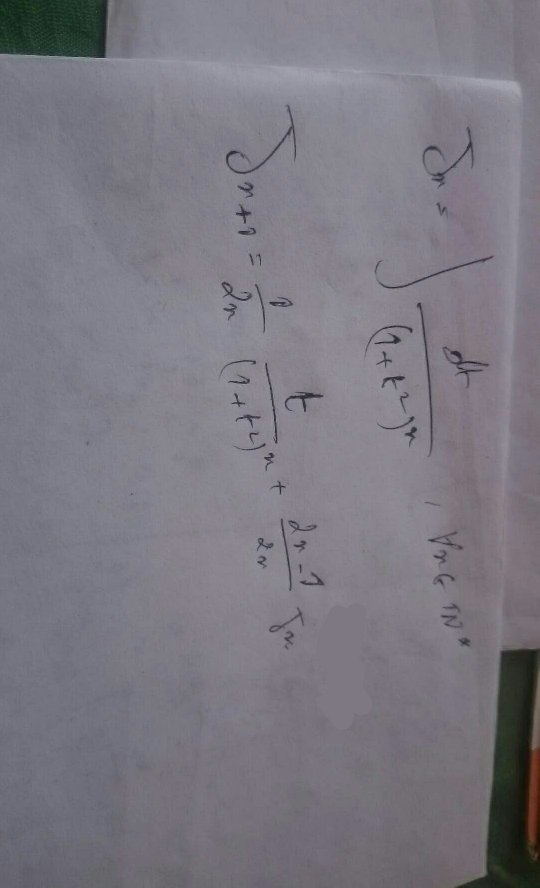
Question Number 64302 Answers: 0 Comments: 0

Question Number 64300 Answers: 0 Comments: 0

Question Number 64299 Answers: 0 Comments: 0

Question Number 64297 Answers: 0 Comments: 0

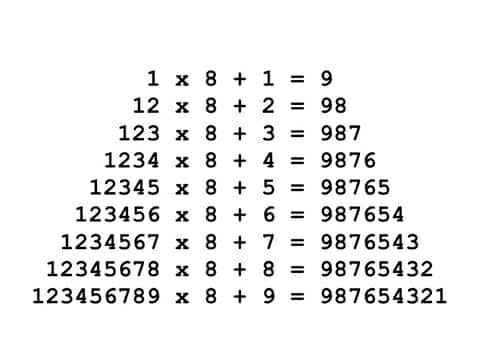
Question Number 64295 Answers: 0 Comments: 0

Question Number 64289 Answers: 2 Comments: 2

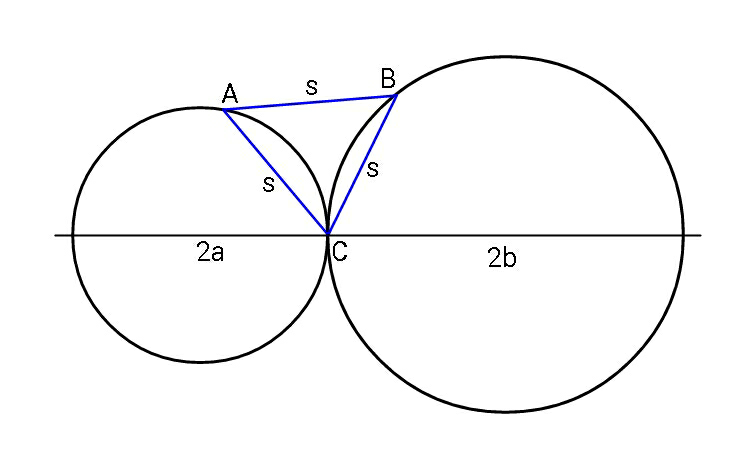
Question Number 64287 Answers: 0 Comments: 3

Question Number 64284 Answers: 0 Comments: 0

Find all the integer solution 3x^2 + 1 = 4y^3

$$\mathrm{Find}\:\mathrm{all}\:\mathrm{the}\:\mathrm{integer}\:\mathrm{solution}\:\:\:\:\:\:\:\mathrm{3x}^{\mathrm{2}} \:+\:\mathrm{1}\:\:=\:\:\mathrm{4y}^{\mathrm{3}} \\ $$

Question Number 64253 Answers: 0 Comments: 1

Pg 1454 Pg 1455 Pg 1456 Pg 1457 Pg 1458 Pg 1459 Pg 1460 Pg 1461 Pg 1462 Pg 1463

Contact: info@tinkutara.com