
AllQuestion and Answers: Page 1457
Question Number 64475 Answers: 2 Comments: 6
Question Number 64471 Answers: 1 Comments: 0
Question Number 64469 Answers: 1 Comments: 0

Question Number 64465 Answers: 0 Comments: 0
Question Number 64463 Answers: 0 Comments: 1
Question Number 64459 Answers: 0 Comments: 2
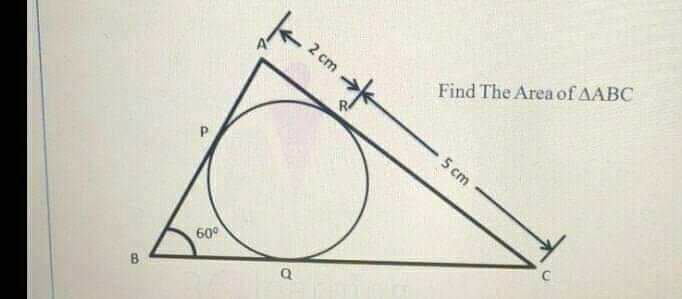
Question Number 64452 Answers: 1 Comments: 5

Question Number 64456 Answers: 0 Comments: 1

Question Number 64448 Answers: 1 Comments: 1
Question Number 64447 Answers: 1 Comments: 0
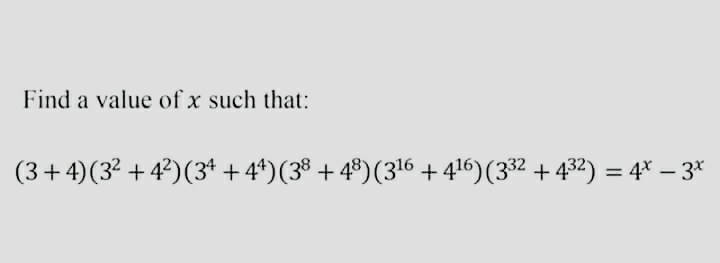
Question Number 64445 Answers: 0 Comments: 0
$${calculate}\:\:{W}_{{n}} =\sum_{\mathrm{1}\leqslant{i}\leqslant{j}\leqslant{n}} \:{i}×{j} \\ $$
Question Number 64444 Answers: 0 Comments: 2
Question Number 64443 Answers: 0 Comments: 0
Question Number 64436 Answers: 2 Comments: 1
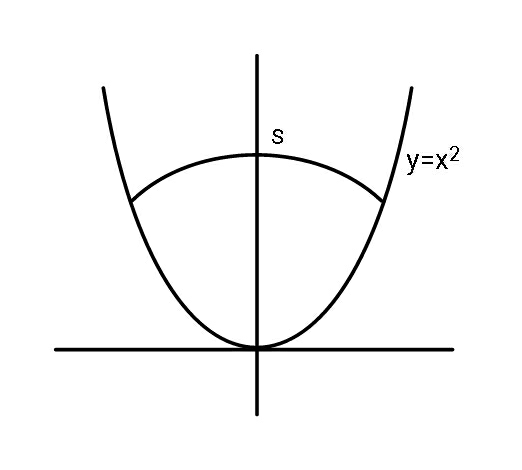
Question Number 64429 Answers: 0 Comments: 5
Question Number 64425 Answers: 0 Comments: 4

Question Number 64419 Answers: 0 Comments: 1
Question Number 64418 Answers: 0 Comments: 3
Question Number 64414 Answers: 1 Comments: 0
Question Number 64410 Answers: 1 Comments: 2
$$\int\mathrm{1}/\left(\mathrm{1}+{ysin}\theta\right){d}\theta \\ $$
Question Number 64406 Answers: 0 Comments: 1

Question Number 64404 Answers: 0 Comments: 4
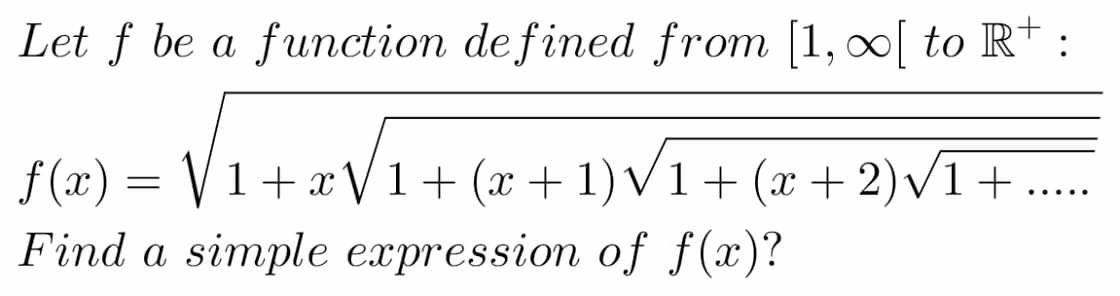
Question Number 64397 Answers: 1 Comments: 0
$$\frac{\mathrm{7}}{\mathrm{4x}−\mathrm{1}}−\frac{\mathrm{5}}{\mathrm{4x}−\mathrm{1}} \\ $$
Question Number 64396 Answers: 1 Comments: 0
$$\left(\mathrm{x}+\mathrm{2}\right)\left(\mathrm{x}−\mathrm{2}\right) \\ $$
Question Number 64392 Answers: 0 Comments: 2
Question Number 64391 Answers: 0 Comments: 1
$$\frac{\mathrm{6}}{\mathrm{a}+\mathrm{5}}+\frac{\mathrm{4}}{\mathrm{a}+\mathrm{5}} \\ $$
Pg 1452 Pg 1453 Pg 1454 Pg 1455 Pg 1456 Pg 1457 Pg 1458 Pg 1459 Pg 1460 Pg 1461
