Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1448

Question Number 66318 Answers: 0 Comments: 4

find lim_(x→0) (((1+x)/(1−x)))^(1/(sinx))

$${find}\:{lim}_{{x}\rightarrow\mathrm{0}} \left(\frac{\mathrm{1}+{x}}{\mathrm{1}−{x}}\right)^{\frac{\mathrm{1}}{{sinx}}} \\ $$

Question Number 66317 Answers: 0 Comments: 2

calculate lim_(x→0) ((ln(cosx))/(1−cos(2x)))

$${calculate}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\frac{{ln}\left({cosx}\right)}{\mathrm{1}−{cos}\left(\mathrm{2}{x}\right)} \\ $$

Question Number 66316 Answers: 1 Comments: 1

lim_(x→(π/2)) ((ln(sin^2 x))/(((π/2)−x)^2 ))

$${lim}_{{x}\rightarrow\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{ln}\left({sin}^{\mathrm{2}} {x}\right)}{\left(\frac{\pi}{\mathrm{2}}−{x}\right)^{\mathrm{2}} } \\ $$$$ \\ $$

Question Number 66325 Answers: 0 Comments: 3

calculate ∫_0 ^(π/4) cos^4 x sin^2 x dx

$${calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:{cos}^{\mathrm{4}} {x}\:{sin}^{\mathrm{2}} {x}\:{dx} \\ $$

Question Number 66308 Answers: 0 Comments: 1

find ∫ (dx/((x+3)(√(−x^2 −4x))))

$${find}\:\int\:\:\:\frac{{dx}}{\left({x}+\mathrm{3}\right)\sqrt{−{x}^{\mathrm{2}} −\mathrm{4}{x}}} \\ $$

Question Number 66310 Answers: 1 Comments: 1

Question Number 66309 Answers: 0 Comments: 2

calculate ∫ (dx/((x^2 −1)(√(x^2 +2))))

$${calculate}\:\:\int\:\:\:\:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} −\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}} \\ $$

Question Number 66304 Answers: 1 Comments: 0

calculate lim_(x→0) ((ln(x+1+sin(πx)))/(xsin(2x)))

$${calculate}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\:\:\frac{{ln}\left({x}+\mathrm{1}+{sin}\left(\pi{x}\right)\right)}{{xsin}\left(\mathrm{2}{x}\right)} \\ $$

Question Number 66302 Answers: 0 Comments: 3

solved the general quintic, despite whatever proof that it cant be solved in a simple way!

$${solved}\:{the}\:{general}\:{quintic}, \\ $$$${despite}\:{whatever}\:{proof}\:{that}\:{it} \\ $$$${cant}\:{be}\:{solved}\:{in}\:{a}\:{simple}\:{way}! \\ $$

Question Number 66293 Answers: 0 Comments: 3

What do we mean by ∫_(−∞) ^(+∞) f(x) dx?

$${What}\:{do}\:{we}\:{mean}\:{by}\:\: \\ $$$$\:\:\int_{−\infty} ^{+\infty} {f}\left({x}\right)\:{dx}? \\ $$

Question Number 66290 Answers: 1 Comments: 0

(x^2 )^(1/(√3)) + x^(√3) − 392 = 0

$$\:\sqrt[{\sqrt{\mathrm{3}}}]{\boldsymbol{\mathrm{x}}^{\mathrm{2}} }\:+\:\boldsymbol{\mathrm{x}}^{\sqrt{\mathrm{3}}} \:−\:\mathrm{392}\:=\:\mathrm{0} \\ $$

Question Number 66287 Answers: 1 Comments: 0

10^(10^(10^(.∙^(.10) ) ) ) =?

$$\mathrm{10}^{\mathrm{10}^{\mathrm{10}^{.\centerdot^{.\mathrm{10}} } } } =?\: \\ $$

Question Number 66285 Answers: 2 Comments: 0

What is the difference between lim_(x→2^− ) and lim_(x→2^+ )

$${What}\:{is}\:{the}\:{difference}\:{between} \\ $$$$\:\:\underset{{x}\rightarrow\mathrm{2}^{−} } {{lim}}\:\:{and} \\ $$$$\underset{{x}\rightarrow\mathrm{2}^{+} } {{lim}} \\ $$

Question Number 66279 Answers: 1 Comments: 1

Value of maximum f(x)=^2 log(x+5)+^2 log(3−x) is... a.4 b.8 c. 12 d. 15 e. 16

$$\mathrm{Value}\:\mathrm{of}\:\mathrm{maximum} \\ $$$${f}\left({x}\right)=^{\mathrm{2}} \mathrm{log}\left({x}+\mathrm{5}\right)+^{\mathrm{2}} \mathrm{log}\left(\mathrm{3}−{x}\right) \\ $$$$\mathrm{is}... \\ $$$$\mathrm{a}.\mathrm{4} \\ $$$$\mathrm{b}.\mathrm{8} \\ $$$$\mathrm{c}.\:\mathrm{12} \\ $$$$\mathrm{d}.\:\mathrm{15} \\ $$$$\mathrm{e}.\:\mathrm{16} \\ $$

Question Number 66276 Answers: 1 Comments: 0

If a and b is root equation 3^(log_3 (4x^2 +3)) +4^(log_2 (x^2 −1)) =49 then a+b=.. a. 3 b. 2 c. 1 d. 0 e. −1

$$\mathrm{If}\:{a}\:\mathrm{and}\:{b}\:\mathrm{is}\:\mathrm{root}\:\mathrm{equation} \\ $$$$\mathrm{3}^{\mathrm{log}_{\mathrm{3}} \left(\mathrm{4x}^{\mathrm{2}} +\mathrm{3}\right)} +\mathrm{4}^{\mathrm{log}_{\mathrm{2}} \left(\mathrm{x}^{\mathrm{2}} −\mathrm{1}\right)} =\mathrm{49} \\ $$$$\mathrm{then} \\ $$$${a}+{b}=.. \\ $$$${a}.\:\mathrm{3} \\ $$$${b}.\:\mathrm{2} \\ $$$${c}.\:\mathrm{1} \\ $$$${d}.\:\mathrm{0} \\ $$$${e}.\:−\mathrm{1} \\ $$$$ \\ $$

Question Number 66274 Answers: 0 Comments: 1

Question Number 66275 Answers: 0 Comments: 2

Prove that for p,q,r∈N ((lcm(p,q,r))/(gcd(p,q,r)))=((p×q×r)/(gcd(p,q)×gcd(q,r)×gcd(r,p)))

$${Prove}\:{that}\:{for}\:{p},{q},{r}\in\mathbb{N} \\ $$$$\frac{\mathrm{lcm}\left({p},{q},{r}\right)}{\mathrm{gcd}\left({p},{q},{r}\right)}=\frac{{p}×{q}×{r}}{\mathrm{gcd}\left({p},{q}\right)×\mathrm{gcd}\left({q},{r}\right)×\mathrm{gcd}\left({r},{p}\right)} \\ $$

Question Number 66267 Answers: 0 Comments: 1

Question Number 66264 Answers: 0 Comments: 0

for x>0 what is the relation between Γ(x) and Γ((1/x))?

$${for}\:{x}>\mathrm{0}\:{what}\:{is}\:{the}\:{relation}\:{between}\:\Gamma\left({x}\right)\:{and}\:\Gamma\left(\frac{\mathrm{1}}{{x}}\right)? \\ $$

Question Number 66263 Answers: 0 Comments: 1

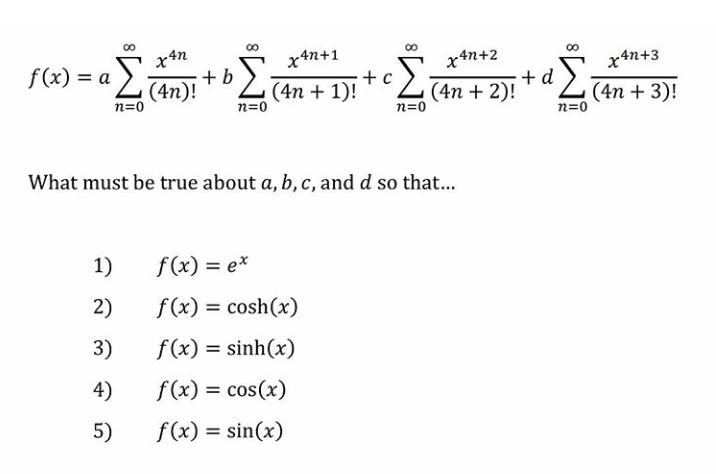
Question Number 66262 Answers: 0 Comments: 3

show that ^n c_(r+1) +^n c_(r ) =^(n+1) c_(r+1)

$$\boldsymbol{{show}}\:\boldsymbol{{that}}\: \\ $$$$\:^{\boldsymbol{{n}}} \boldsymbol{{c}}_{\boldsymbol{{r}}+\mathrm{1}} +^{\boldsymbol{{n}}} \boldsymbol{{c}}_{\boldsymbol{{r}}\:\:} =^{\boldsymbol{{n}}+\mathrm{1}} \boldsymbol{{c}}_{\boldsymbol{{r}}+\mathrm{1}} \\ $$

Question Number 66256 Answers: 0 Comments: 7

Find all points (a, b) of R^2 such that through (a, b) pass two tangent lines to the graph of f(x)=x^2 .

$${Find}\:{all}\:{points}\:\left({a},\:{b}\right)\:{of}\:\mathbb{R}^{\mathrm{2}} \:{such}\:{that}\: \\ $$$${through}\:\left({a},\:{b}\right)\:{pass}\:{two}\:{tangent}\:{lines} \\ $$$${to}\:{the}\:{graph}\:{of}\:{f}\left({x}\right)={x}^{\mathrm{2}} . \\ $$

Question Number 66253 Answers: 0 Comments: 1

prove by Rieman sum that ∫_0 ^1 xdx =(1/2)

$${prove}\:{by}\:{Rieman}\:{sum}\:{that}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{xdx}\:=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Question Number 66249 Answers: 1 Comments: 0

The npn transistor in the voltage amplifier circuit operates satisfctorily on a quiescent collector current of 3mA. if the battery supply (V_(cc) ) is 6v, calculate the value of ; (a) the load resistor R_L (b) the base current for the quiescent collector−emitter voltage V_(ce) to be half the battery voltage. Th transistor dc current is 100. please help.

$$\mathrm{The}\:\mathrm{npn}\:\mathrm{transistor}\:\mathrm{in}\:\mathrm{the}\:\mathrm{voltage}\: \\ $$$$\mathrm{amplifier}\:\mathrm{circuit}\:\mathrm{operates}\:\mathrm{satisfctorily} \\ $$$$\mathrm{on}\:\mathrm{a}\:\mathrm{quiescent}\:\mathrm{collector}\:\mathrm{current}\:\mathrm{of}\:\mathrm{3mA}. \\ $$$$\mathrm{if}\:\mathrm{the}\:\mathrm{battery}\:\mathrm{supply}\:\left(\mathrm{V}_{\mathrm{cc}} \right)\:\mathrm{is}\:\mathrm{6v},\:\mathrm{calculate} \\ $$$$\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:; \\ $$$$\left(\mathrm{a}\right)\:\mathrm{the}\:\mathrm{load}\:\mathrm{resistor}\:\mathrm{R}_{\mathrm{L}} \\ $$$$\left(\mathrm{b}\right)\:\mathrm{the}\:\mathrm{base}\:\mathrm{current}\:\mathrm{for}\:\mathrm{the}\:\mathrm{quiescent} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{collector}−\mathrm{emitter}\:\mathrm{voltage}\:\mathrm{V}_{\mathrm{ce}} \:\mathrm{to} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{be}\:\mathrm{half}\:\mathrm{the}\:\mathrm{battery}\:\mathrm{voltage}. \\ $$$$\mathrm{Th}\:\mathrm{transistor}\:\mathrm{dc}\:\mathrm{current}\:\mathrm{is}\:\mathrm{100}. \\ $$$$ \\ $$$$\mathrm{please}\:\mathrm{help}. \\ $$

Question Number 66245 Answers: 1 Comments: 2

prove that ∫e^x dx = e^x + c

$${prove}\:{that} \\ $$$$ \\ $$$$\int{e}^{{x}} \:{dx}\:=\:{e}^{{x}} \:+\:{c} \\ $$

Question Number 66228 Answers: 0 Comments: 3

prove that ∫_2 ^4 ((6x +1)/((2x−3)(3x−2)))dx = ln 10

$${prove}\:{that}\: \\ $$$$\int_{\mathrm{2}} ^{\mathrm{4}} \frac{\mathrm{6}{x}\:+\mathrm{1}}{\left(\mathrm{2}{x}−\mathrm{3}\right)\left(\mathrm{3}{x}−\mathrm{2}\right)}{dx}\:=\:{ln}\:\mathrm{10} \\ $$

Pg 1443 Pg 1444 Pg 1445 Pg 1446 Pg 1447 Pg 1448 Pg 1449 Pg 1450 Pg 1451 Pg 1452

Terms of Service

Contact: info@tinkutara.com