
AllQuestion and Answers: Page 1440
Question Number 65971 Answers: 2 Comments: 0
Question Number 65970 Answers: 1 Comments: 0
Question Number 65961 Answers: 1 Comments: 0
Question Number 65951 Answers: 0 Comments: 1

Question Number 65950 Answers: 4 Comments: 0
$$\int_{\mathrm{0}} ^{\:\pi/\mathrm{2}} \mathrm{tan}\:^{\mathrm{3}} {xdx}\:=\:? \\ $$
Question Number 68063 Answers: 1 Comments: 0

Question Number 65981 Answers: 1 Comments: 0

Question Number 65980 Answers: 3 Comments: 1
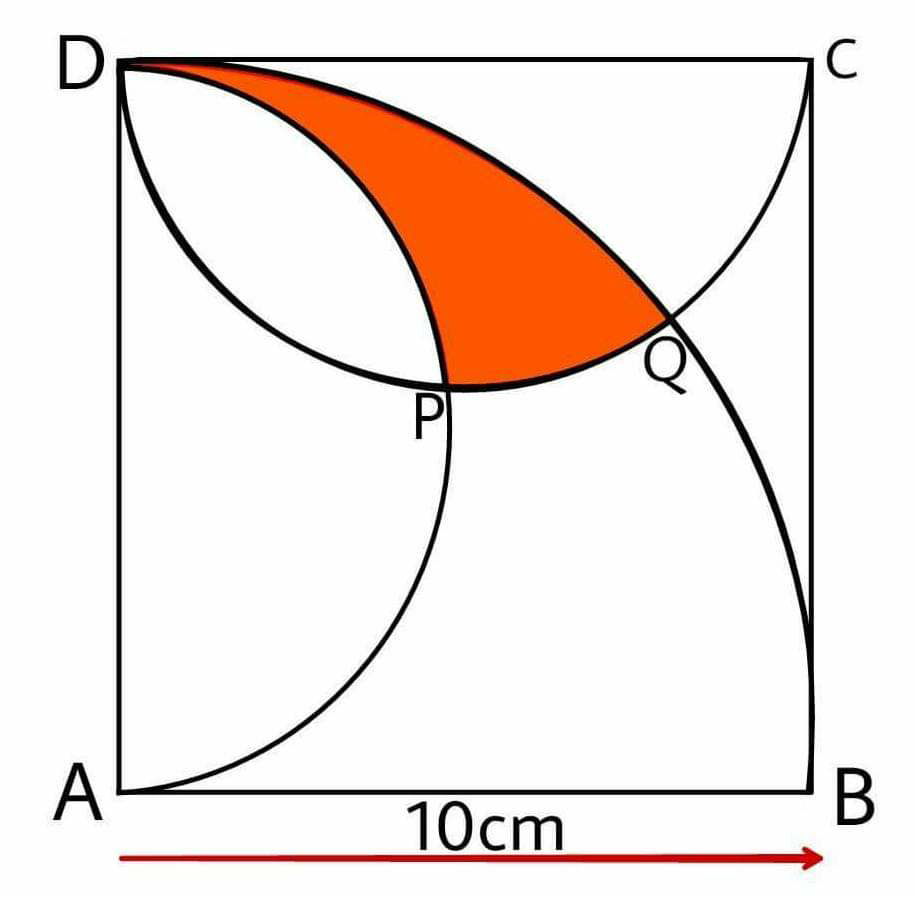
Question Number 65945 Answers: 0 Comments: 2
Question Number 65959 Answers: 0 Comments: 0
Question Number 65934 Answers: 3 Comments: 0
Question Number 65931 Answers: 1 Comments: 0
Question Number 65930 Answers: 1 Comments: 0
Question Number 65929 Answers: 1 Comments: 2

Question Number 65926 Answers: 0 Comments: 0
Question Number 65925 Answers: 0 Comments: 3
Question Number 65924 Answers: 0 Comments: 1
Question Number 65923 Answers: 0 Comments: 0
Question Number 65922 Answers: 0 Comments: 1
Question Number 65920 Answers: 1 Comments: 1
$${fnd}\:\int\:\frac{{dx}}{{x}+\mathrm{2}−\sqrt{\mathrm{3}+{x}^{\mathrm{2}} }} \\ $$
Question Number 65919 Answers: 1 Comments: 1
Question Number 65918 Answers: 1 Comments: 1
Question Number 65917 Answers: 0 Comments: 0
Question Number 65916 Answers: 0 Comments: 0
Question Number 65915 Answers: 0 Comments: 0
Question Number 65914 Answers: 0 Comments: 0
Pg 1435 Pg 1436 Pg 1437 Pg 1438 Pg 1439 Pg 1440 Pg 1441 Pg 1442 Pg 1443 Pg 1444
