Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1436

Question Number 67069 Answers: 0 Comments: 1

Question Number 67059 Answers: 0 Comments: 1

find the area abounded y=(√(x−2)) and y=x−2 ?

$${find}\:{the}\:{area}\:{abounded}\:{y}=\sqrt{{x}−\mathrm{2}} \\ $$$${and}\:{y}={x}−\mathrm{2}\:? \\ $$

Question Number 67058 Answers: 0 Comments: 0

find the area abounded y=(√(x−2)) and y=x−2 ?

$${find}\:{the}\:{area}\:{abounded}\:{y}=\sqrt{{x}−\mathrm{2}} \\ $$$${and}\:{y}={x}−\mathrm{2}\:? \\ $$

Question Number 67057 Answers: 1 Comments: 0

(1)find ∩_(n=1) ^∞ [0, (1/n)) (2)find ∪_(n=2) ^∞ [(1/n), 1−(1/n)]

$$\left(\mathrm{1}\right){find}\:\cap_{{n}=\mathrm{1}} ^{\infty} \left[\mathrm{0},\:\frac{\mathrm{1}}{{n}}\right) \\ $$$$\left(\mathrm{2}\right){find}\:\cup_{{n}=\mathrm{2}} ^{\infty} \left[\frac{\mathrm{1}}{{n}},\:\mathrm{1}−\frac{\mathrm{1}}{{n}}\right] \\ $$

Question Number 67055 Answers: 0 Comments: 0

let Z_+ =N∪{0}, f: Z_+ ×Z_+ →Z_+ f(m, n)=(((m+n)(m+n+1))/2)+m prove that f is a one-to-one function and also an onto function

$${let}\:\mathbb{Z}_{+} =\mathbb{N}\cup\left\{\mathrm{0}\right\},\:{f}:\:\mathbb{Z}_{+} ×\mathbb{Z}_{+} \rightarrow\mathbb{Z}_{+} \\ $$$${f}\left({m},\:{n}\right)=\frac{\left({m}+{n}\right)\left({m}+{n}+\mathrm{1}\right)}{\mathrm{2}}+{m} \\ $$$${prove}\:{that}\:{f}\:{is}\:{a}\:{one}-{to}-{one}\:{function} \\ $$$${and}\:{also}\:{an}\:{onto}\:{function} \\ $$

Question Number 67038 Answers: 1 Comments: 1

calculate ∫_(−1) ^1 (x^(2n) /(1+2^(sinx) ))dx with n integr.

$${calculate}\:\:\int_{−\mathrm{1}} ^{\mathrm{1}} \:\frac{{x}^{\mathrm{2}{n}} }{\mathrm{1}+\mathrm{2}^{{sinx}} }{dx}\:\:\:{with}\:{n}\:{integr}. \\ $$

Question Number 67035 Answers: 1 Comments: 2

Question Number 67034 Answers: 0 Comments: 2

calculate Σ_(n=1) ^∞ ((cos(2nx))/n)

$${calculate}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{{cos}\left(\mathrm{2}{nx}\right)}{{n}} \\ $$

Question Number 67033 Answers: 0 Comments: 5

Question Number 67032 Answers: 0 Comments: 0

Question Number 67031 Answers: 0 Comments: 4

lim_(x→−1) ((((x^5 )^(1/7) +1)/(1+(x^7 )^(1/(9 )) )))^(1/3) =?

$$\: \\ $$$$\:\underset{\boldsymbol{\mathrm{x}}\rightarrow−\mathrm{1}} {\boldsymbol{\mathrm{lim}}}\sqrt[{\mathrm{3}}]{\frac{\sqrt[{\mathrm{7}}]{\boldsymbol{\mathrm{x}}^{\mathrm{5}} }+\mathrm{1}}{\mathrm{1}+\sqrt[{\mathrm{9}\:}]{\boldsymbol{\mathrm{x}}^{\mathrm{7}} }}}=? \\ $$$$\: \\ $$

Question Number 67026 Answers: 0 Comments: 5

Question Number 67025 Answers: 0 Comments: 3

Question Number 67023 Answers: 1 Comments: 1

find the sequence U_n wich verify U_n +U_(n+1) =sin(n) ∀n from n

$${find}\:{the}\:{sequence}\:{U}_{{n}} \:{wich}\:{verify}\:\:{U}_{{n}} +{U}_{{n}+\mathrm{1}} ={sin}\left({n}\right)\:\:\forall{n}\:{from}\:{n} \\ $$

Question Number 67022 Answers: 0 Comments: 0

find ∫ (1+(√x))(√(x^2 +3))dx

$${find}\:\int\:\left(\mathrm{1}+\sqrt{{x}}\right)\sqrt{{x}^{\mathrm{2}} +\mathrm{3}}{dx} \\ $$

Question Number 67021 Answers: 0 Comments: 1

find f(x) =∫_0 ^1 ln(x +e^(−t) )dt with x>0

$${find}\:{f}\left({x}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} {ln}\left({x}\:+{e}^{−{t}} \right){dt}\:\:\:{with}\:{x}>\mathrm{0} \\ $$

Question Number 67020 Answers: 0 Comments: 1

find f(x) = ∫_0 ^1 arctan(1+xt)dt with x real

$${find}\:{f}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{arctan}\left(\mathrm{1}+{xt}\right){dt}\:\:{with}\:{x}\:{real} \\ $$

Question Number 67019 Answers: 0 Comments: 0

calculate ∫_0 ^∞ e^(−x^2 ) arctan(x^2 )dx

$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:{e}^{−{x}^{\mathrm{2}} } {arctan}\left({x}^{\mathrm{2}} \right){dx}\: \\ $$

Question Number 67018 Answers: 0 Comments: 1

find ∫_0 ^∞ e^(−x) ln(1+x)dx

$${find}\:\:\int_{\mathrm{0}} ^{\infty} \:\:{e}^{−{x}} {ln}\left(\mathrm{1}+{x}\right){dx} \\ $$

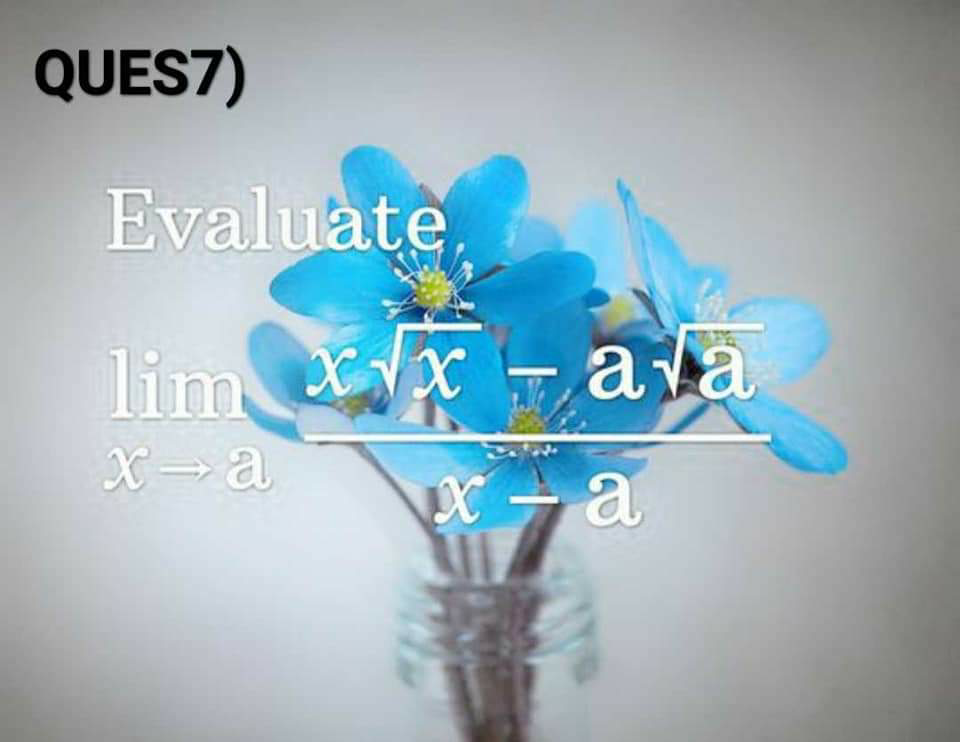
Question Number 67017 Answers: 0 Comments: 1

find ∫ arctan(1+(√(x+1)))dx

$${find}\:\int\:\:{arctan}\left(\mathrm{1}+\sqrt{{x}+\mathrm{1}}\right){dx} \\ $$

Question Number 67016 Answers: 0 Comments: 0

find ∫ arctan(1+(√x)+(√(x+1)))dx

$${find}\:\int\:{arctan}\left(\mathrm{1}+\sqrt{{x}}+\sqrt{{x}+\mathrm{1}}\right){dx} \\ $$

Question Number 67015 Answers: 0 Comments: 0

let f(x) =arctan(1+e^(−(√(1+x^2 ))) ) calculate f^′ (x) and f^(′′) (x). 1)find lim_(x→+∞) f(x) and lim_(x→−∞) f(x) 3)study the variation of f(x) 4)give the equation of tangent to C_f at A(1,f(1))

$${let}\:{f}\left({x}\right)\:={arctan}\left(\mathrm{1}+{e}^{−\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \right) \\ $$$${calculate}\:{f}^{'} \left({x}\right)\:\:{and}\:{f}^{''} \left({x}\right). \\ $$$$\left.\mathrm{1}\right){find}\:{lim}_{{x}\rightarrow+\infty} {f}\left({x}\right)\:{and}\:{lim}_{{x}\rightarrow−\infty} \:\:\:{f}\left({x}\right) \\ $$$$\left.\mathrm{3}\right){study}\:{the}\:{variation}\:{of}\:{f}\left({x}\right) \\ $$$$\left.\mathrm{4}\right){give}\:{the}\:{equation}\:{of}\:{tangent}\:{to}\:{C}_{{f}} \:{at}\:\:{A}\left(\mathrm{1},{f}\left(\mathrm{1}\right)\right) \\ $$

Question Number 67014 Answers: 0 Comments: 1

solve y^(′′) +x^2 y^′ =e^(−x) sin(3x)

$${solve}\:{y}^{''} +{x}^{\mathrm{2}} {y}^{'} ={e}^{−{x}} {sin}\left(\mathrm{3}{x}\right) \\ $$

Question Number 67013 Answers: 0 Comments: 1

find the value of Σ_(n=1) ^∞ (((−1)^n )/((n+1)n^3 ))

$${find}\:{the}\:{value}\:{of}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left({n}+\mathrm{1}\right){n}^{\mathrm{3}} } \\ $$

Question Number 67012 Answers: 0 Comments: 1

find ∫_1 ^(+∞) ((arctan([x]))/x^3 )dx

$${find}\:\int_{\mathrm{1}} ^{+\infty} \:\frac{{arctan}\left(\left[{x}\right]\right)}{{x}^{\mathrm{3}} }{dx} \\ $$

Question Number 67011 Answers: 0 Comments: 1

calculate U_n =∫_1 ^(+∞) ((arctan(n[x]))/x^2 )dx

$${calculate}\:{U}_{{n}} =\int_{\mathrm{1}} ^{+\infty} \:\:\frac{{arctan}\left({n}\left[{x}\right]\right)}{{x}^{\mathrm{2}} }{dx} \\ $$

Pg 1431 Pg 1432 Pg 1433 Pg 1434 Pg 1435 Pg 1436 Pg 1437 Pg 1438 Pg 1439 Pg 1440

Contact: info@tinkutara.com