Question and Answers Forum

AllQuestion and Answers: Page 1423

Question Number 68636 Answers: 2 Comments: 0

((−a^2 +a+1)/( a^2 +a+1))=((−b^2 +b+1)/( b^2 +b+1)) = (( 2a^2 −2ab+(b−a))/(−2a^2 +2ab+(b−a))) =((−2ab+(a+b)+2)/( 2ab+(a+b)+2)) Solve for a.

$$\frac{−{a}^{\mathrm{2}} +{a}+\mathrm{1}}{\:\:\:\:{a}^{\mathrm{2}} +{a}+\mathrm{1}}=\frac{−{b}^{\mathrm{2}} +{b}+\mathrm{1}}{\:\:\:\:{b}^{\mathrm{2}} +{b}+\mathrm{1}} \\ $$$$\:\:=\:\frac{\:\:\:\:\mathrm{2}{a}^{\mathrm{2}} −\mathrm{2}{ab}+\left({b}−{a}\right)}{−\mathrm{2}{a}^{\mathrm{2}} +\mathrm{2}{ab}+\left({b}−{a}\right)} \\ $$$$\:\:=\frac{−\mathrm{2}{ab}+\left({a}+{b}\right)+\mathrm{2}}{\:\:\:\:\mathrm{2}{ab}+\left({a}+{b}\right)+\mathrm{2}} \\ $$$${Solve}\:{for}\:\boldsymbol{{a}}. \\ $$$$ \\ $$

Question Number 68629 Answers: 1 Comments: 0

Question Number 68626 Answers: 0 Comments: 0

lim ((−n(2−a)^n )/((2−a))) n→∞

$$\mathrm{l}{im}\:\:\frac{−{n}\left(\mathrm{2}−{a}\right)^{{n}} }{\left(\mathrm{2}−{a}\right)} \\ $$$${n}\rightarrow\infty \\ $$

Question Number 68624 Answers: 2 Comments: 0

Question Number 68618 Answers: 1 Comments: 0

solve for x the following equations a) log x^3 − 2log x^2 + 2log x + 2log (√x) = 3 b) log_x 24 −3log_x 4 + 2log_x 3 =−3

$${solve}\:{for}\:{x}\:{the}\:{following}\:{equations} \\ $$$$\left.{a}\right)\:{log}\:{x}^{\mathrm{3}} \:−\:\mathrm{2}{log}\:{x}^{\mathrm{2}} \:+\:\mathrm{2}{log}\:{x}\:\:+\:\mathrm{2}{log}\:\sqrt{{x}}\:=\:\mathrm{3} \\ $$$$\left.{b}\right)\:{log}_{{x}} \mathrm{24}\:−\mathrm{3}{log}_{{x}} \mathrm{4}\:\:+\:\mathrm{2}{log}_{{x}} \mathrm{3}\:=−\mathrm{3} \\ $$

Question Number 68617 Answers: 0 Comments: 1

find the value of p given that 3^p × 3^(−1) × 5 × 3^(p−1) = 2 × 3^4

$${find}\:{the}\:{value}\:{of}\:{p}\:{given}\:{that} \\ $$$$\mathrm{3}^{{p}} \:×\:\mathrm{3}^{−\mathrm{1}} \:×\:\mathrm{5}\:×\:\mathrm{3}^{{p}−\mathrm{1}} \:=\:\mathrm{2}\:×\:\mathrm{3}^{\mathrm{4}} \\ $$

Question Number 68616 Answers: 2 Comments: 0

given that a,b and c are positive numbers other than 1 , show that log_b a × log_c b × log_a c = 1 hence, evaluate log_(10) 25 × log_2 10 × log_5 4

$${given}\:{that}\:{a},{b}\:{and}\:{c}\:{are}\:{positive}\:{numbers}\:{other}\:{than}\:\mathrm{1} \\ $$$$,\:{show}\:{that}\:\:{log}_{{b}} {a}\:×\:{log}_{{c}} {b}\:×\:{log}_{{a}} {c}\:=\:\mathrm{1} \\ $$$${hence},\:{evaluate}\:\:\:{log}_{\mathrm{10}} \mathrm{25}\:×\:{log}_{\mathrm{2}} \mathrm{10}\:×\:{log}_{\mathrm{5}} \mathrm{4} \\ $$

Question Number 68611 Answers: 1 Comments: 0

Question Number 68609 Answers: 1 Comments: 1

Question Number 68608 Answers: 0 Comments: 0

Question Number 68601 Answers: 0 Comments: 4

ABCD is a side square 1. B, F and E are collinear. FDE is a right triangle with hypotenuse 1 and the DE cathetus is worth x. What is the value of x? (Solve with algebra)

$${ABCD}\:\mathrm{is}\:\mathrm{a}\:\mathrm{side}\:\mathrm{square}\:\mathrm{1}.\: \\ $$$${B},\:{F}\:\mathrm{and}\:{E}\:\mathrm{are}\:\mathrm{collinear}. \\ $$$${FDE}\:\mathrm{is}\:\mathrm{a}\:\mathrm{right}\:\mathrm{triangle}\:\mathrm{with}\:\mathrm{hypotenuse}\:\mathrm{1} \\ $$$$\mathrm{and}\:\mathrm{the}\:{DE}\:\mathrm{cathetus}\:\mathrm{is}\:\mathrm{worth}\:\boldsymbol{{x}}.\: \\ $$$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\boldsymbol{{x}}? \\ $$$$\left(\mathrm{Solve}\:\mathrm{with}\:\mathrm{algebra}\right) \\ $$

Question Number 68600 Answers: 0 Comments: 1

1)calculatef(a)= ∫_0 ^∞ ((cos(arctanx))/(a+x^2 ))dx with a>0 2)?calculste g(a) =∫_0 ^∞ ((cos(arctanx))/((a+x^2 )^2 )) 3)find the value if integrals ∫_0 ^∞ ((cos(arctanx))/(2+x^2 )) and ∫_0 ^∞ ((cos(arctanx))/((1+x^2 )^2 ))

$$\left.\mathrm{1}\right){calculatef}\left({a}\right)=\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({arctanx}\right)}{{a}+{x}^{\mathrm{2}} }{dx}\:{with}\:{a}>\mathrm{0} \\ $$$$\left.\mathrm{2}\right)?{calculste}\:\:{g}\left({a}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\frac{{cos}\left({arctanx}\right)}{\left({a}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$$$\left.\mathrm{3}\right){find}\:{the}\:{value}\:{if}\:{integrals} \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({arctanx}\right)}{\mathrm{2}+{x}^{\mathrm{2}} }\:{and}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({arctanx}\right)}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{2}} } \\ $$

Question Number 68598 Answers: 0 Comments: 2

find ∫ (dx/(x^3 −4x +3))

$$\:{find}\:\int\:\:\:\:\frac{{dx}}{{x}^{\mathrm{3}} −\mathrm{4}{x}\:+\mathrm{3}} \\ $$

Question Number 68597 Answers: 0 Comments: 1

calculate ∫_0 ^∞ ((arctan(e^x^2 ))/(x^2 +8))dx

$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{arctan}\left({e}^{{x}^{\mathrm{2}} } \right)}{{x}^{\mathrm{2}} \:+\mathrm{8}}{dx} \\ $$

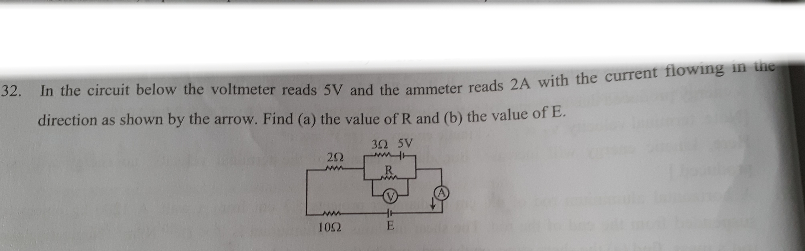
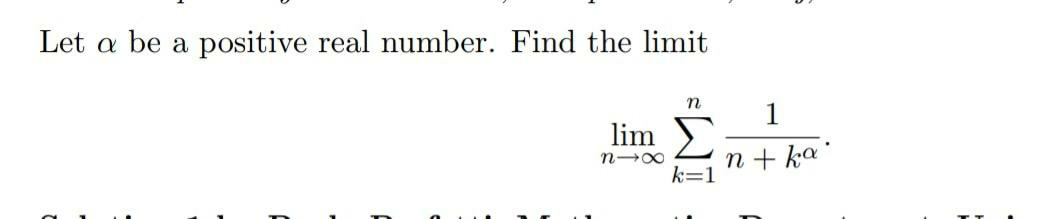
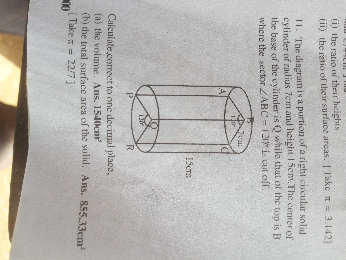
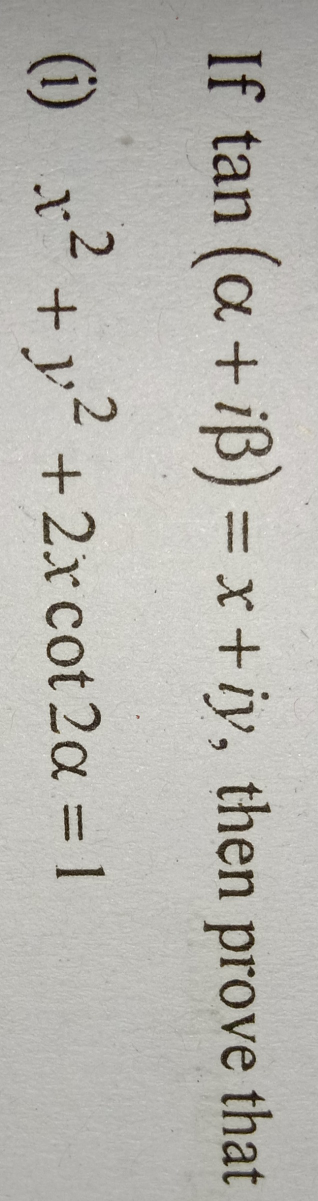
Question Number 68596 Answers: 0 Comments: 1

calculate ∫_(π/2) ^(π/3) ((xdx)/(3+cosx))

$${calculate}\:\int_{\frac{\pi}{\mathrm{2}}} ^{\frac{\pi}{\mathrm{3}}} \:\:\:\:\frac{{xdx}}{\mathrm{3}+{cosx}} \\ $$

Question Number 68595 Answers: 0 Comments: 1

calculate A_λ =∫_0 ^∞ (e^(−λx^2 ) /(x^4 +1))dx with λ>0 and find ∫_0 ^1 A_λ dλ