
AllQuestion and Answers: Page 1420
Question Number 67977 Answers: 1 Comments: 1

Question Number 67974 Answers: 0 Comments: 2
Question Number 67973 Answers: 0 Comments: 5

Question Number 67972 Answers: 2 Comments: 0
Question Number 67969 Answers: 0 Comments: 0
Question Number 67963 Answers: 1 Comments: 1

Question Number 67960 Answers: 0 Comments: 2
Question Number 67959 Answers: 0 Comments: 1
$$\int\sqrt{{e}^{{y}^{\mathrm{2}} } \:\:}\:{dy}\:\:{pleas}\:{sir}\:{can}\:{you}\:{help}\:{me}? \\ $$
Question Number 67958 Answers: 0 Comments: 0
Question Number 67948 Answers: 0 Comments: 0
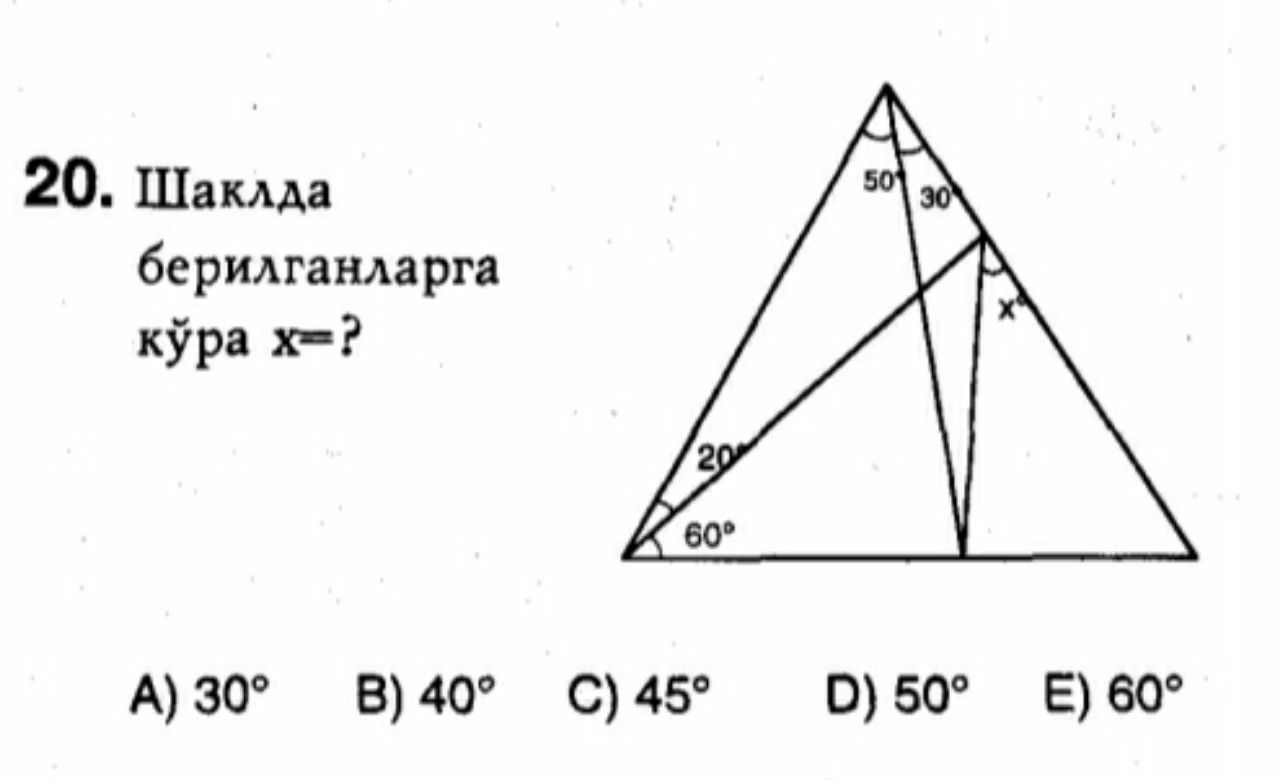
Question Number 67946 Answers: 0 Comments: 3
Question Number 67943 Answers: 0 Comments: 0
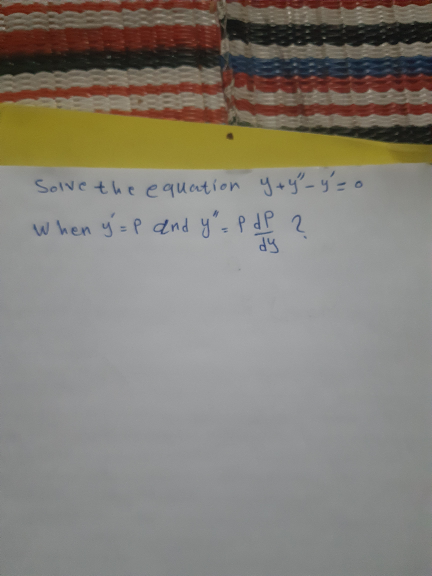
Question Number 67942 Answers: 0 Comments: 1
Question Number 67939 Answers: 1 Comments: 2
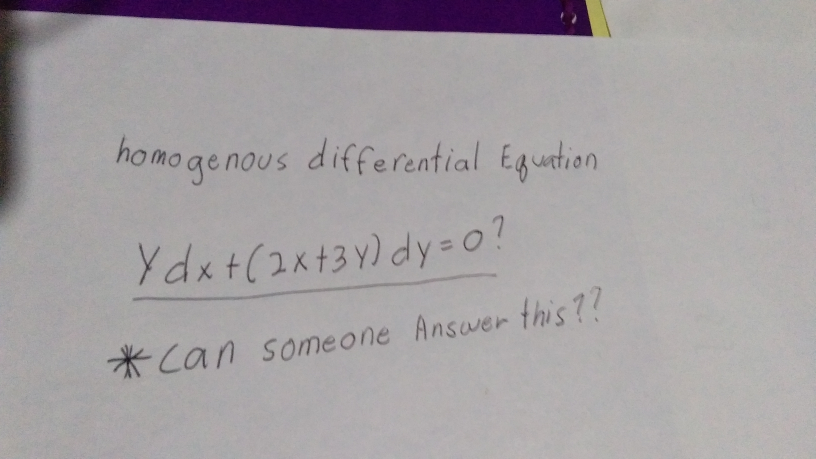
Question Number 67937 Answers: 0 Comments: 1
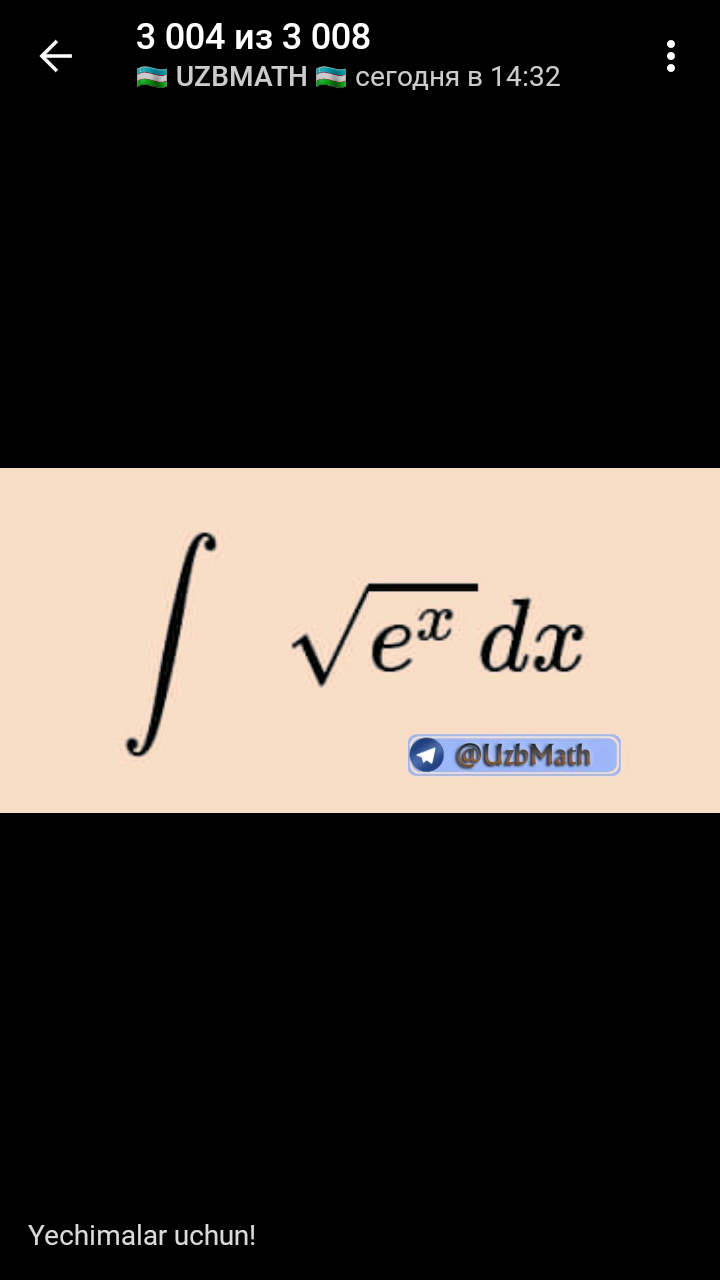
Question Number 67932 Answers: 1 Comments: 4
Question Number 67931 Answers: 0 Comments: 0
Question Number 67927 Answers: 0 Comments: 9
Question Number 67919 Answers: 0 Comments: 0
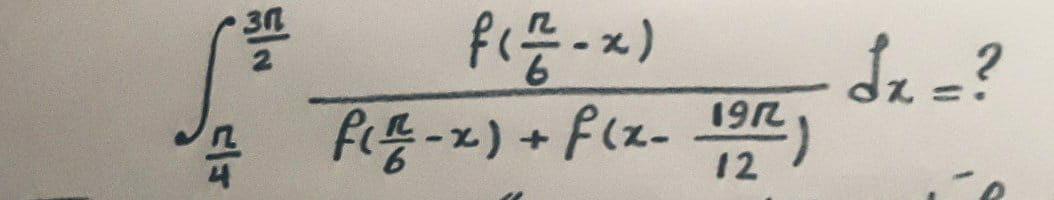
Question Number 67918 Answers: 1 Comments: 0

Question Number 68226 Answers: 0 Comments: 1
Question Number 67921 Answers: 0 Comments: 0
Question Number 67920 Answers: 0 Comments: 0

Question Number 67907 Answers: 1 Comments: 1

Question Number 67903 Answers: 2 Comments: 0

Question Number 67902 Answers: 0 Comments: 0
Pg 1415 Pg 1416 Pg 1417 Pg 1418 Pg 1419 Pg 1420 Pg 1421 Pg 1422 Pg 1423 Pg 1424
