
AllQuestion and Answers: Page 1419
Question Number 68510 Answers: 1 Comments: 0
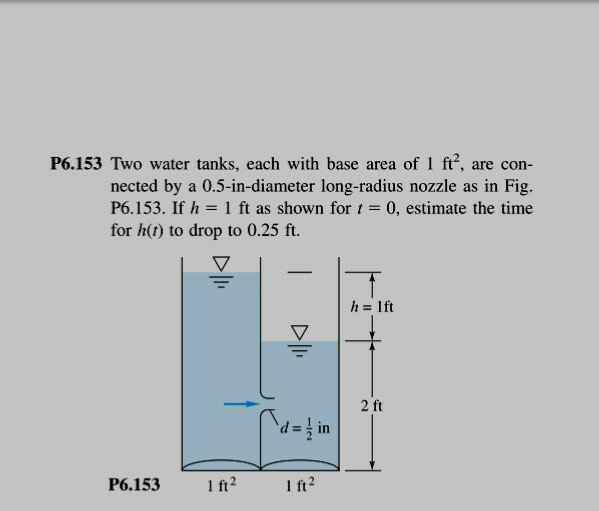
Question Number 68509 Answers: 0 Comments: 0

Question Number 68508 Answers: 0 Comments: 0

Question Number 68506 Answers: 0 Comments: 0
Question Number 68503 Answers: 0 Comments: 8

Question Number 68524 Answers: 1 Comments: 0

Question Number 68528 Answers: 0 Comments: 0
Question Number 68495 Answers: 1 Comments: 1
Question Number 68493 Answers: 1 Comments: 0
Question Number 68482 Answers: 0 Comments: 1
Question Number 68481 Answers: 0 Comments: 6
Question Number 68576 Answers: 1 Comments: 1
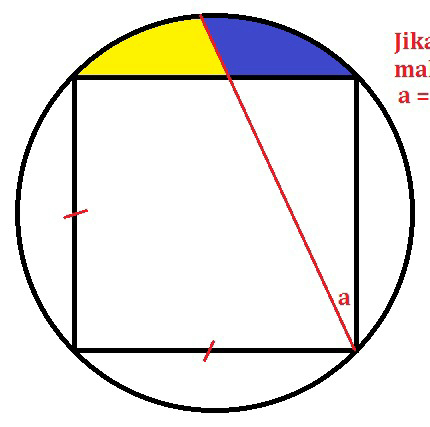
Question Number 68470 Answers: 0 Comments: 3
Question Number 68466 Answers: 1 Comments: 2
Question Number 68473 Answers: 0 Comments: 1
Question Number 68451 Answers: 1 Comments: 7

Question Number 68446 Answers: 0 Comments: 1
Question Number 68434 Answers: 1 Comments: 0

Question Number 68433 Answers: 0 Comments: 0
Question Number 68487 Answers: 1 Comments: 2

Question Number 68425 Answers: 1 Comments: 2

Question Number 68422 Answers: 1 Comments: 0

Question Number 68418 Answers: 1 Comments: 3

Question Number 68414 Answers: 2 Comments: 1
Question Number 68409 Answers: 1 Comments: 3
Question Number 68405 Answers: 1 Comments: 3
Pg 1414 Pg 1415 Pg 1416 Pg 1417 Pg 1418 Pg 1419 Pg 1420 Pg 1421 Pg 1422 Pg 1423
