
AllQuestion and Answers: Page 1416
Question Number 68783 Answers: 1 Comments: 5

Question Number 68782 Answers: 0 Comments: 0
Question Number 68775 Answers: 1 Comments: 0

Question Number 68774 Answers: 0 Comments: 0

Question Number 68773 Answers: 0 Comments: 0

Question Number 68768 Answers: 1 Comments: 1
Question Number 68767 Answers: 0 Comments: 1

Question Number 68761 Answers: 1 Comments: 1
Question Number 68740 Answers: 1 Comments: 1
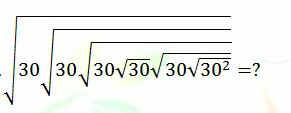
Question Number 68721 Answers: 1 Comments: 1

Question Number 68732 Answers: 0 Comments: 0
Question Number 68714 Answers: 1 Comments: 0

Question Number 68712 Answers: 0 Comments: 3
Question Number 68710 Answers: 0 Comments: 3

Question Number 68703 Answers: 0 Comments: 5
Question Number 68699 Answers: 1 Comments: 2
Question Number 68695 Answers: 1 Comments: 0
Question Number 68693 Answers: 0 Comments: 2

Question Number 68788 Answers: 0 Comments: 1
$$\int\mathrm{1}/\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{{n}} \:{dx} \\ $$
Question Number 68676 Answers: 0 Comments: 2
Question Number 68675 Answers: 0 Comments: 3
Question Number 68673 Answers: 0 Comments: 8
Question Number 68664 Answers: 1 Comments: 0
Question Number 68660 Answers: 0 Comments: 2
Question Number 68666 Answers: 1 Comments: 2

Question Number 68642 Answers: 0 Comments: 3
Pg 1411 Pg 1412 Pg 1413 Pg 1414 Pg 1415 Pg 1416 Pg 1417 Pg 1418 Pg 1419 Pg 1420
