
AllQuestion and Answers: Page 1411
Question Number 69429 Answers: 0 Comments: 2

Question Number 69423 Answers: 0 Comments: 1

Question Number 69418 Answers: 0 Comments: 0

Question Number 69416 Answers: 1 Comments: 1

Question Number 69413 Answers: 0 Comments: 1

Question Number 69398 Answers: 2 Comments: 1

Question Number 69393 Answers: 0 Comments: 2

Question Number 69390 Answers: 0 Comments: 0
Question Number 69389 Answers: 0 Comments: 0
$${find}\:\int_{\mid{z}+{i}\mid=\mathrm{3}} \:\:\frac{{sinz}}{{z}+{i}}{dz} \\ $$
Question Number 69379 Answers: 0 Comments: 1
Question Number 69377 Answers: 0 Comments: 0
Question Number 69376 Answers: 0 Comments: 1
Question Number 69375 Answers: 0 Comments: 0
Question Number 69374 Answers: 0 Comments: 1
Question Number 69373 Answers: 0 Comments: 2
Question Number 69585 Answers: 0 Comments: 0
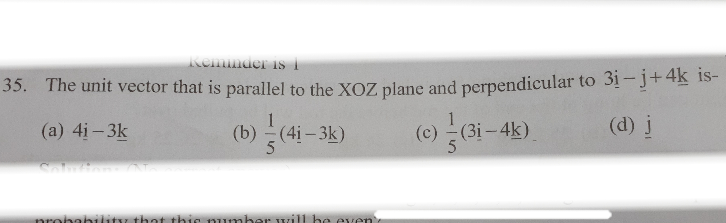
Question Number 69355 Answers: 1 Comments: 0
Question Number 69347 Answers: 1 Comments: 0
Question Number 69338 Answers: 1 Comments: 1

Question Number 69412 Answers: 1 Comments: 1

Question Number 69411 Answers: 0 Comments: 1

Question Number 69328 Answers: 0 Comments: 1
Question Number 69482 Answers: 1 Comments: 1
$$\underset{{n}\rightarrow\infty} {{lim}}\frac{\mathrm{2}+{cosn}}{\mathrm{4}{n}+{sinn}}\:=\:? \\ $$
Question Number 74637 Answers: 1 Comments: 1
Question Number 69319 Answers: 0 Comments: 4
Question Number 69385 Answers: 2 Comments: 0

Pg 1406 Pg 1407 Pg 1408 Pg 1409 Pg 1410 Pg 1411 Pg 1412 Pg 1413 Pg 1414 Pg 1415
