
AllQuestion and Answers: Page 1408
Question Number 69847 Answers: 1 Comments: 0
Question Number 69846 Answers: 1 Comments: 0
Question Number 69836 Answers: 1 Comments: 2

Question Number 69829 Answers: 2 Comments: 2

Question Number 69827 Answers: 2 Comments: 0
Question Number 69809 Answers: 2 Comments: 2
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:{x}\mathrm{sin}\:\frac{\pi}{{x}} \\ $$
Question Number 69803 Answers: 0 Comments: 2
Question Number 69795 Answers: 0 Comments: 1
Question Number 69794 Answers: 1 Comments: 2
Question Number 69792 Answers: 1 Comments: 1
Question Number 69790 Answers: 0 Comments: 1
Question Number 69789 Answers: 0 Comments: 0
Question Number 69786 Answers: 0 Comments: 1
Question Number 69784 Answers: 0 Comments: 0
Question Number 69778 Answers: 2 Comments: 5
Question Number 69766 Answers: 0 Comments: 4
Question Number 69765 Answers: 1 Comments: 0
Question Number 69764 Answers: 1 Comments: 1
Question Number 69763 Answers: 1 Comments: 2
Question Number 69762 Answers: 1 Comments: 1
Question Number 69754 Answers: 0 Comments: 2

Question Number 69746 Answers: 1 Comments: 1

Question Number 69741 Answers: 2 Comments: 4
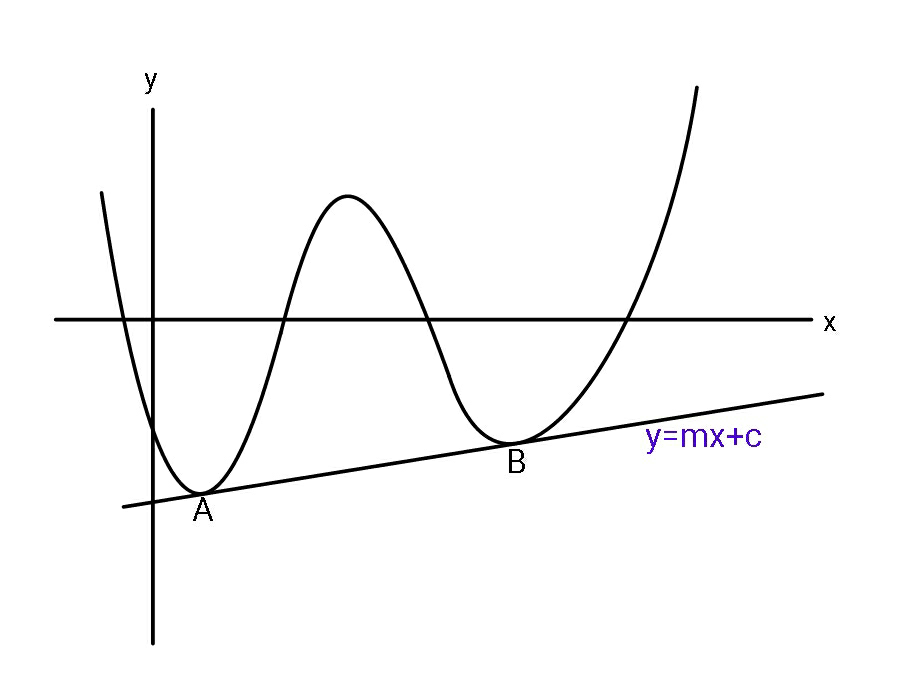
Question Number 69738 Answers: 1 Comments: 0
Question Number 69735 Answers: 1 Comments: 1
Question Number 69734 Answers: 0 Comments: 1
Pg 1403 Pg 1404 Pg 1405 Pg 1406 Pg 1407 Pg 1408 Pg 1409 Pg 1410 Pg 1411 Pg 1412
