
AllQuestion and Answers: Page 1407
Question Number 70159 Answers: 1 Comments: 1

Question Number 70150 Answers: 0 Comments: 1
Question Number 70147 Answers: 1 Comments: 4
Question Number 70145 Answers: 1 Comments: 0
Question Number 70138 Answers: 1 Comments: 0
Question Number 70135 Answers: 0 Comments: 1
Question Number 70132 Answers: 1 Comments: 1
$$\underset{{n}=\mathrm{1}} {\overset{\mathrm{3050}} {\sum}}\:{i}^{{n}} \\ $$
Question Number 70121 Answers: 1 Comments: 0
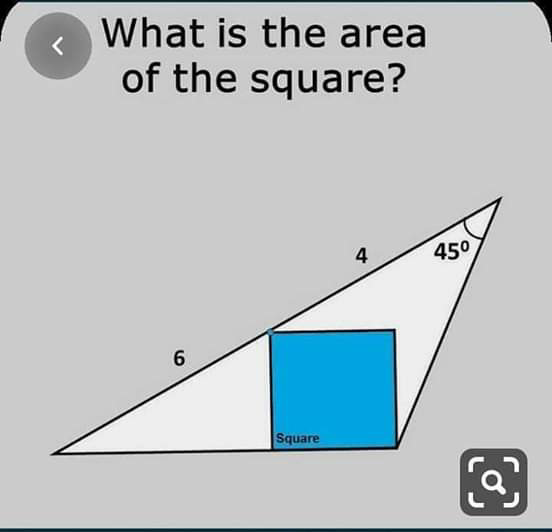
Question Number 70108 Answers: 1 Comments: 0
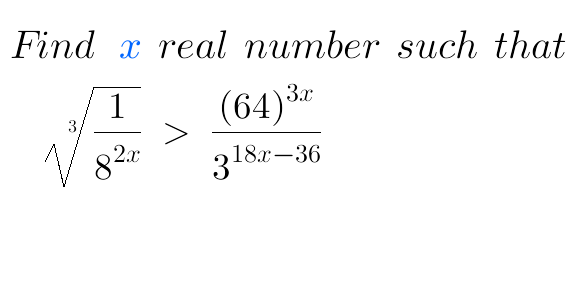
Question Number 70103 Answers: 2 Comments: 0
Question Number 70075 Answers: 0 Comments: 3
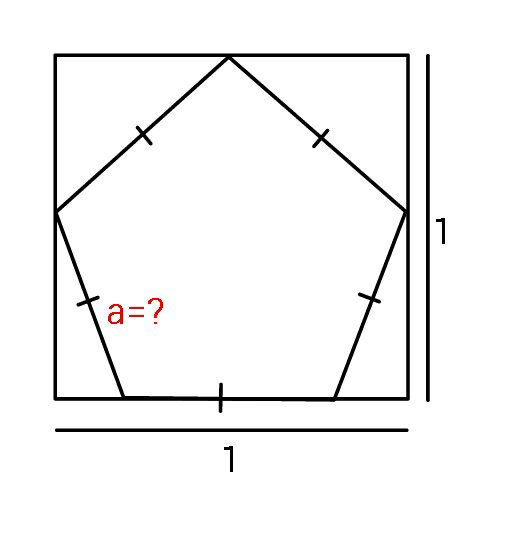
Question Number 70074 Answers: 1 Comments: 1
Question Number 70069 Answers: 1 Comments: 2
Question Number 70066 Answers: 2 Comments: 0
Question Number 70048 Answers: 1 Comments: 6

Question Number 70044 Answers: 0 Comments: 1
Question Number 70040 Answers: 1 Comments: 3
Question Number 70035 Answers: 0 Comments: 4

Question Number 70031 Answers: 0 Comments: 0
Question Number 70030 Answers: 1 Comments: 0
Question Number 70052 Answers: 1 Comments: 0
Question Number 70051 Answers: 2 Comments: 0
Question Number 70025 Answers: 0 Comments: 1
Question Number 70022 Answers: 0 Comments: 1
Question Number 70021 Answers: 0 Comments: 2
Question Number 70008 Answers: 1 Comments: 0
Pg 1402 Pg 1403 Pg 1404 Pg 1405 Pg 1406 Pg 1407 Pg 1408 Pg 1409 Pg 1410 Pg 1411
