
AllQuestion and Answers: Page 1406
Question Number 70361 Answers: 0 Comments: 0
Question Number 70312 Answers: 1 Comments: 1
Question Number 70364 Answers: 0 Comments: 3
Question Number 70310 Answers: 3 Comments: 1
Question Number 70277 Answers: 2 Comments: 0

Question Number 70270 Answers: 1 Comments: 0
Question Number 70262 Answers: 0 Comments: 1
Question Number 70256 Answers: 0 Comments: 1
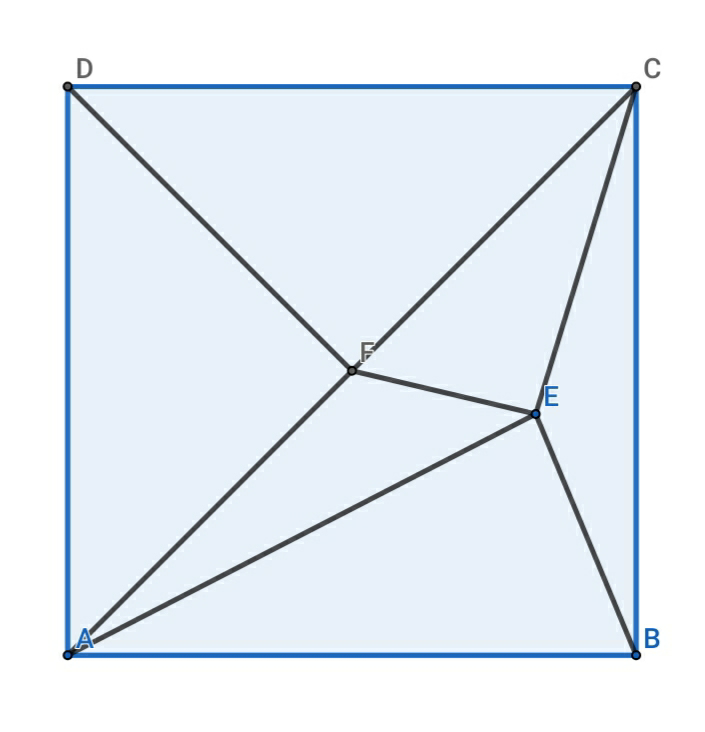
Question Number 70253 Answers: 0 Comments: 3
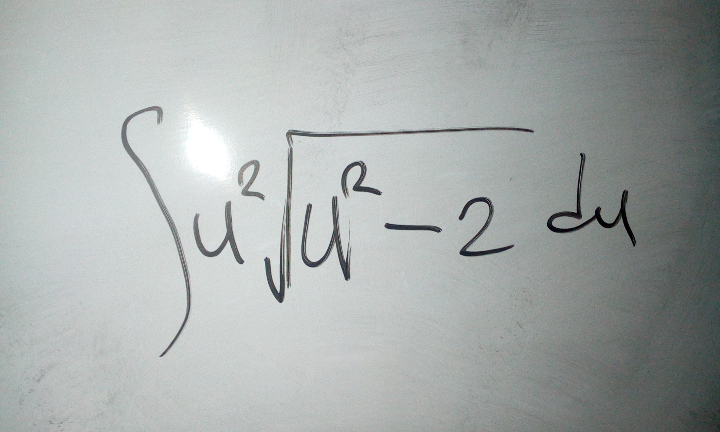
Question Number 70252 Answers: 1 Comments: 0
Question Number 70287 Answers: 0 Comments: 2
Question Number 70232 Answers: 0 Comments: 3
$$\mathrm{Solve} \\ $$$${xy}'\:−\:{y}\:\mathrm{sin}\:{x}\:+\:{y}^{\mathrm{5}} \:=\:\mathrm{0} \\ $$
Question Number 70230 Answers: 0 Comments: 2
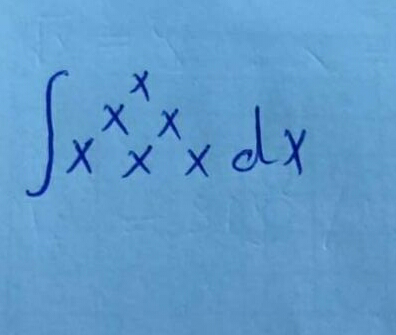
Question Number 70219 Answers: 0 Comments: 1

Question Number 70296 Answers: 1 Comments: 1

Question Number 70225 Answers: 1 Comments: 3

Question Number 70198 Answers: 1 Comments: 0

Question Number 70197 Answers: 0 Comments: 1

Question Number 70196 Answers: 1 Comments: 0

Question Number 70216 Answers: 0 Comments: 0
Question Number 70237 Answers: 0 Comments: 3
Question Number 70298 Answers: 1 Comments: 0
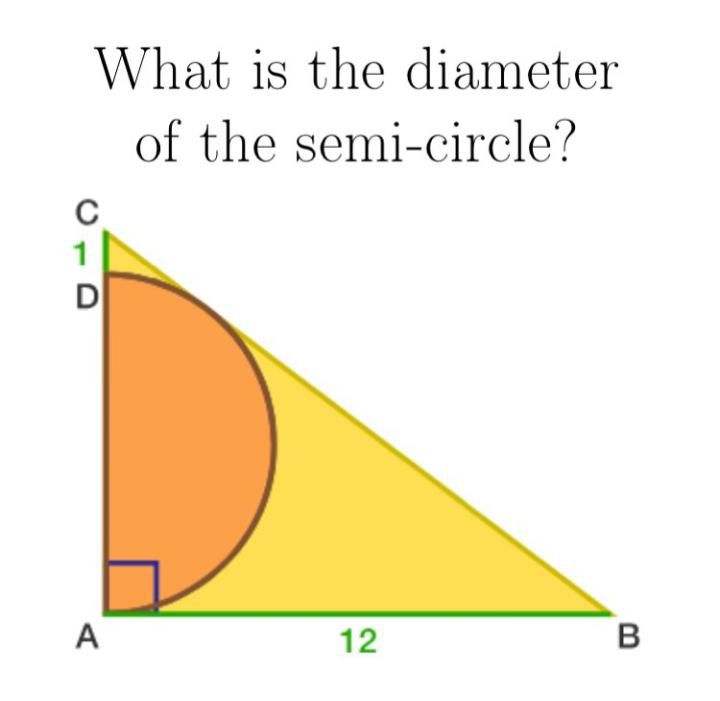
Question Number 70168 Answers: 1 Comments: 3
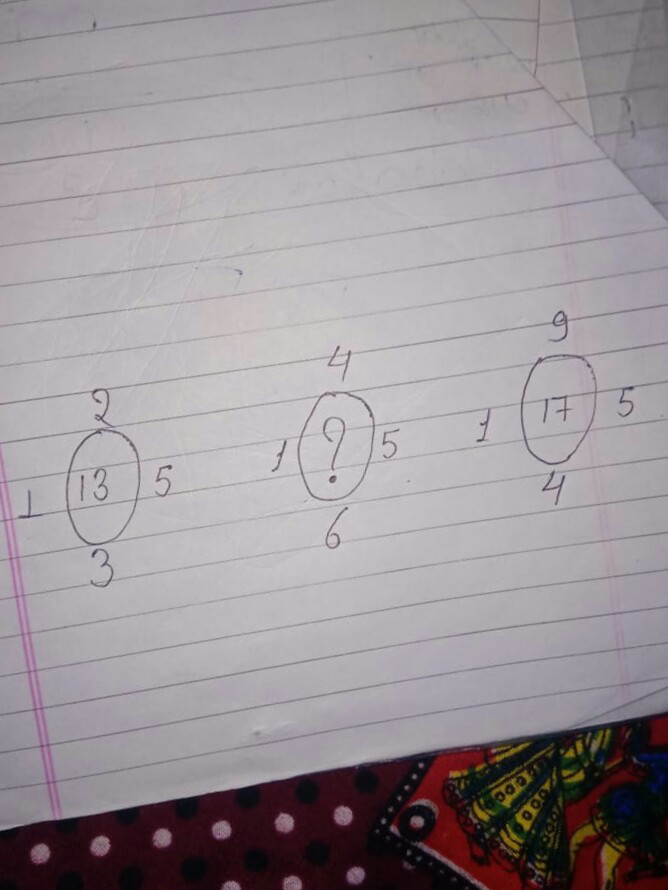
Question Number 70167 Answers: 0 Comments: 1
Question Number 70163 Answers: 1 Comments: 0
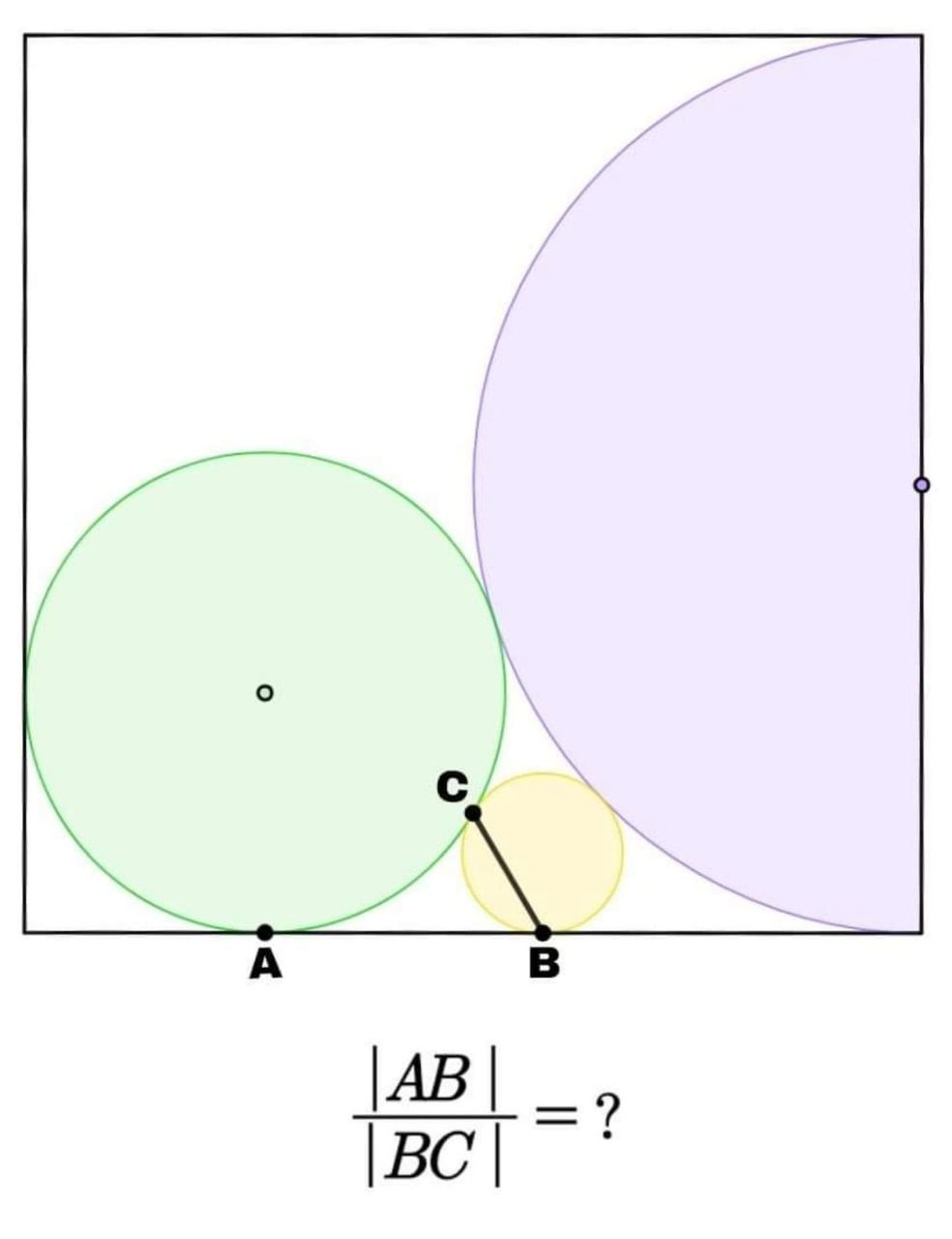
Question Number 70162 Answers: 1 Comments: 0

Pg 1401 Pg 1402 Pg 1403 Pg 1404 Pg 1405 Pg 1406 Pg 1407 Pg 1408 Pg 1409 Pg 1410
