
AllQuestion and Answers: Page 1405
Question Number 70429 Answers: 3 Comments: 6

Question Number 70425 Answers: 0 Comments: 0
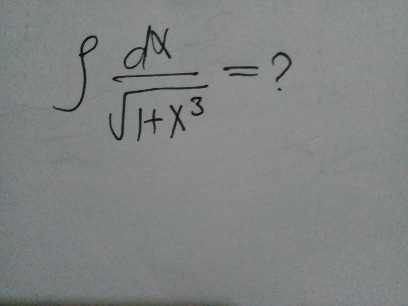
Question Number 70719 Answers: 1 Comments: 0
Question Number 70418 Answers: 0 Comments: 0

Question Number 70417 Answers: 1 Comments: 0

Question Number 70395 Answers: 1 Comments: 1

Question Number 70394 Answers: 1 Comments: 0
Question Number 70397 Answers: 0 Comments: 1

Question Number 70390 Answers: 0 Comments: 0

Question Number 70423 Answers: 2 Comments: 1

Question Number 70385 Answers: 1 Comments: 0
Question Number 70383 Answers: 0 Comments: 1
Question Number 70399 Answers: 1 Comments: 3
Question Number 70370 Answers: 1 Comments: 4
Question Number 70369 Answers: 0 Comments: 1
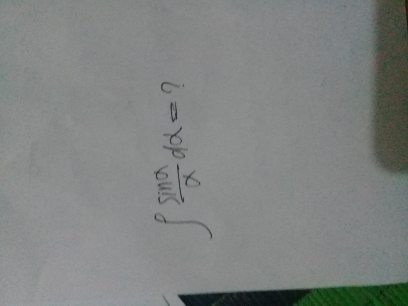
Question Number 70361 Answers: 0 Comments: 0
Question Number 70312 Answers: 1 Comments: 1
Question Number 70364 Answers: 0 Comments: 3
Question Number 70310 Answers: 3 Comments: 1
Question Number 70277 Answers: 2 Comments: 0

Question Number 70270 Answers: 1 Comments: 0
Question Number 70262 Answers: 0 Comments: 1
Question Number 70256 Answers: 0 Comments: 1
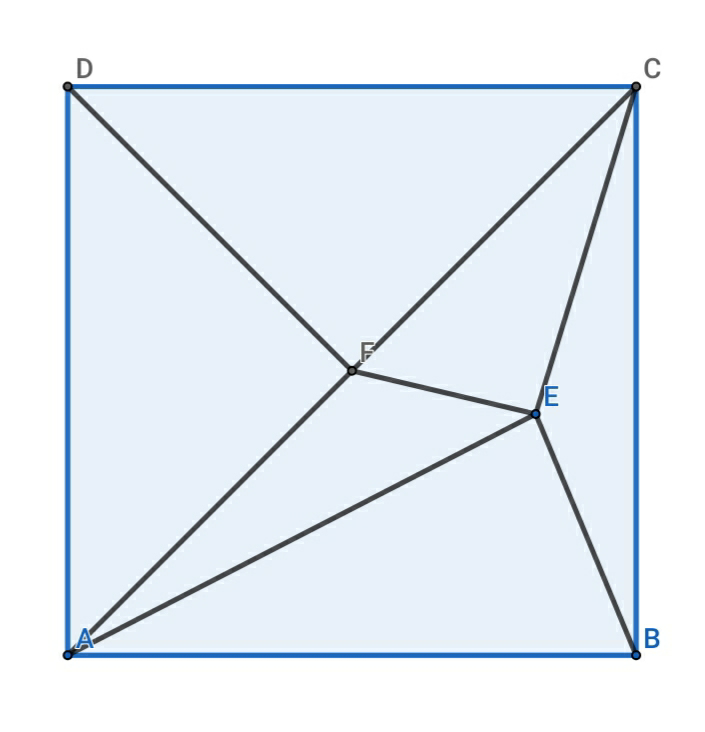
Question Number 70253 Answers: 0 Comments: 3
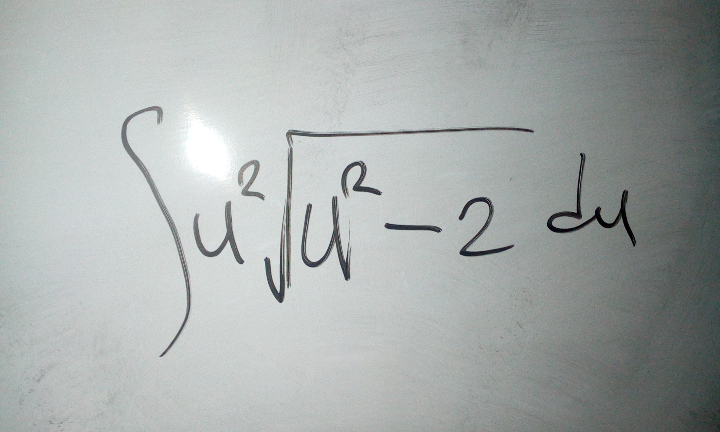
Question Number 70252 Answers: 1 Comments: 0
Question Number 70287 Answers: 0 Comments: 2
Pg 1400 Pg 1401 Pg 1402 Pg 1403 Pg 1404 Pg 1405 Pg 1406 Pg 1407 Pg 1408 Pg 1409
