Question and Answers Forum

Obain an equation for ⇒ the left Reimen Sum ⇒ the right Reimen sum ⇒ Trapeziodal rule ⇒ Newton Raphson′s Iteration Hence find and approximate value for ∫_0 ^3 (e^x + x^2 )dx

$${Obain}\:{an}\:{equation}\:{for}\: \\ $$$$\Rightarrow\:{the}\:{left}\:{Reimen}\:{Sum} \\ $$$$\Rightarrow\:{the}\:{right}\:{Reimen}\:{sum} \\ $$$$\Rightarrow\:{Trapeziodal}\:{rule} \\ $$$$\Rightarrow\:{Newton}\:{Raphson}'{s}\:{Iteration} \\ $$$$\:\:{Hence}\:{find}\:{and}\:{approximate}\:{value}\:{for}\:\int_{\mathrm{0}} ^{\mathrm{3}} \left({e}^{{x}} \:+\:{x}^{\mathrm{2}} \right){dx} \\ $$

Question Number 72332 Answers: 1 Comments: 0

let Q=((1+tan(((3π)/8)) . tan((π/(10))))/(1−tan((π/8)).tan((π/(10))))) prove that ((Q−1)/(Q+1))=(√(7−3(√5)−(√(85−38(√5)))))

$${let}\:{Q}=\frac{\mathrm{1}+{tan}\left(\frac{\mathrm{3}\pi}{\mathrm{8}}\right)\:.\:{tan}\left(\frac{\pi}{\mathrm{10}}\right)}{\mathrm{1}−{tan}\left(\frac{\pi}{\mathrm{8}}\right).{tan}\left(\frac{\pi}{\mathrm{10}}\right)} \\ $$$$ \\ $$$${prove}\:{that} \\ $$$$\: \\ $$$$\frac{{Q}−\mathrm{1}}{{Q}+\mathrm{1}}=\sqrt{\mathrm{7}−\mathrm{3}\sqrt{\mathrm{5}}−\sqrt{\mathrm{85}−\mathrm{38}\sqrt{\mathrm{5}}}} \\ $$$$ \\ $$

Question Number 72316 Answers: 0 Comments: 6

To whom it may concern! To solve some of your questions, I spent a lot of my time and gave much effort to prepare some diagrams. I did′t keep any backup for my answers and especially for the diagrams in my own device, because I thought they were saved in the forum. Since you, I don′t understand why, have deleted these answered questions, I also lose my answers and my diagrams forever. You don′t need to say a “thank you” for the help I give you, but you should be at least polite and respect me and my work. As far as I think you are such a person, regardless how you change your ID, I won′t answer any of your questions even when I know the answer. NOBODY HAS THE RIGHT TO DELETE MY ANSWERS. THEY ARE GIVEN TO ALL MEMBERS OF THE FORUM, NOT FOR YOU PERSONALLY!

$$\boldsymbol{\mathrm{T}{o}}\:\boldsymbol{{whom}}\:\boldsymbol{{it}}\:\boldsymbol{{may}}\:\boldsymbol{{concern}}! \\ $$$${To}\:{solve}\:{some}\:{of}\:{your}\:{questions},\:{I}\: \\ $$$${spent}\:{a}\:{lot}\:{of}\:{my}\:{time}\:{and}\:{gave}\:{much} \\ $$$${effort}\:{to}\:{prepare}\:{some}\:{diagrams}.\:{I} \\ $$$${did}'{t}\:{keep}\:{any}\:{backup}\:{for}\:{my}\:{answers} \\ $$$${and}\:{especially}\:{for}\:{the}\:{diagrams}\:{in}\:{my} \\ $$$${own}\:{device},\:{because}\:{I}\:{thought}\:{they}\: \\ $$$${were}\:{saved}\:{in}\:{the}\:{forum}.\:{Since}\:{you}, \\ $$$${I}\:{don}'{t}\:{understand}\:{why},\:{have}\:{deleted} \\ $$$${these}\:{answered}\:{questions},\:{I}\:{also}\:{lose} \\ $$$${my}\:{answers}\:{and}\:{my}\:{diagrams}\:{forever}. \\ $$$$ \\ $$$${You}\:{don}'{t}\:{need}\:{to}\:{say}\:{a}\:``{thank}\:{you}''\:{for} \\ $$$${the}\:{help}\:{I}\:{give}\:{you},\:{but}\:{you}\:{should}\:{be} \\ $$$${at}\:{least}\:{polite}\:{and}\:{respect}\:{me}\:{and}\:{my} \\ $$$${work}. \\ $$$$ \\ $$$${As}\:{far}\:{as}\:{I}\:{think}\:{you}\:{are}\:{such}\:{a}\:{person}, \\ $$$${regardless}\:{how}\:{you}\:{change}\:{your}\:{ID}, \\ $$$${I}\:{won}'{t}\:{answer}\:{any}\:{of}\:{your}\:{questions} \\ $$$${even}\:{when}\:{I}\:{know}\:{the}\:{answer}. \\ $$$$ \\ $$$${NOBODY}\:{HAS}\:{THE}\:{RIGHT}\:{TO} \\ $$$${DELETE}\:{MY}\:{ANSWERS}.\:{THEY} \\ $$$${ARE}\:{GIVEN}\:{TO}\:{ALL}\:{MEMBERS}\:{OF} \\ $$$${THE}\:{FORUM},\:{NOT}\:{FOR}\:{YOU} \\ $$$${PERSONALLY}! \\ $$

Question Number 72359 Answers: 1 Comments: 2

Question Number 72296 Answers: 1 Comments: 4

Let x = f(x) e^(f(x)) ∫_( 0) ^( e) f(x) dx = ?

$${Let}\:\:\:\:{x}\:\:=\:\:{f}\left({x}\right)\:{e}^{{f}\left({x}\right)} \\ $$$$\:\:\:\:\:\:\underset{\:\mathrm{0}} {\int}\overset{\:{e}} {\:}{f}\left({x}\right)\:{dx}\:\:=\:\:? \\ $$

Question Number 72294 Answers: 1 Comments: 4

Question Number 72291 Answers: 1 Comments: 0

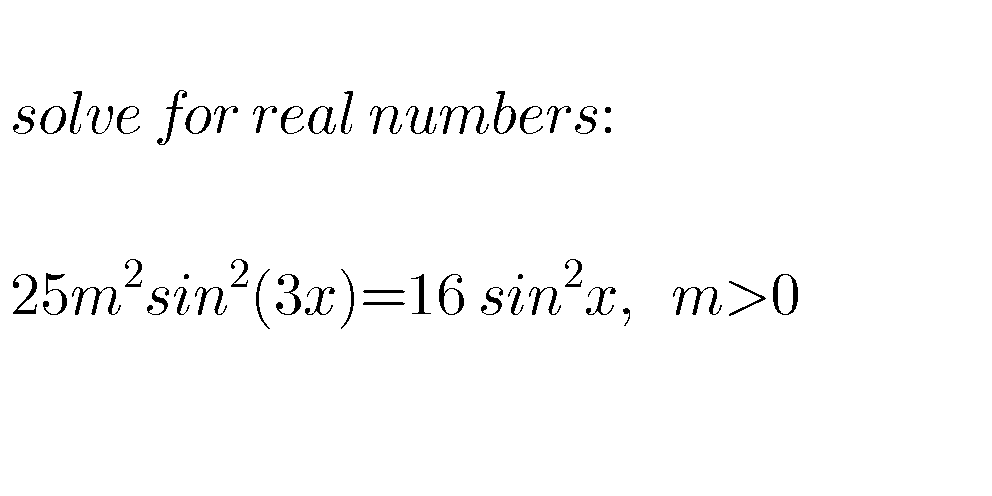
Question Number 72289 Answers: 2 Comments: 0

Bonjour. Aidez moi a resoudre le systeme suivant dans R^2 x−y=2 xy=20

$${Bonjour}. \\ $$$${Aidez}\:{moi}\:{a}\:{resoudre}\:{le}\:{systeme}\: \\ $$$${suivant}\:\:\:\:{dans}\:\mathbb{R}^{\mathrm{2}} \:\:\:\:\: \\ $$$${x}−{y}=\mathrm{2} \\ $$$${xy}=\mathrm{20} \\ $$

Question Number 72275 Answers: 0 Comments: 2

Σ_(i=1) ^6 X_i =42 Find X_1 and X_3

$$\underset{{i}=\mathrm{1}} {\overset{\mathrm{6}} {\sum}}{X}_{{i}} =\mathrm{42} \\ $$$${Find}\:{X}_{\mathrm{1}} \:{and}\:{X}_{\mathrm{3}} \\ $$

Question Number 72260 Answers: 1 Comments: 3

let f(x)=((2x+3)/(x^2 +1)) calculate f^((n)) (x) 2)find f^((10)) (x) and f^((15)) (x) 3)calculate f^((10)) (0) and f^((15)) (0) 4)developp f at integr serie 5)let g(x)=∫_0 ^x f(t)dt developp g at integr serie.

$${let}\:{f}\left({x}\right)=\frac{\mathrm{2}{x}+\mathrm{3}}{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$${calculate}\:\:{f}^{\left({n}\right)} \left({x}\right) \\ $$$$\left.\mathrm{2}\right){find}\:{f}^{\left(\mathrm{10}\right)} \left({x}\right)\:{and}\:{f}^{\left(\mathrm{15}\right)} \left({x}\right) \\ $$$$\left.\mathrm{3}\right){calculate}\:{f}^{\left(\mathrm{10}\right)} \left(\mathrm{0}\right)\:{and}\:{f}^{\left(\mathrm{15}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{4}\right){developp}\:{f}\:{at}\:{integr}\:{serie} \\ $$$$\left.\mathrm{5}\right){let}\:{g}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} {f}\left({t}\right){dt}\:\:{developp}\:{g}\:{at}\:{integr}\:{serie}. \\ $$

Question Number 72259 Answers: 1 Comments: 0

∫yz dx +∫xz dy +∫xy dz pleas sir help me ?

$$\int{yz}\:{dx}\:+\int{xz}\:{dy}\:+\int{xy}\:{dz}\:\:\:\:{pleas}\:{sir}\:{help}\:{me}\:? \\ $$

Question Number 72286 Answers: 0 Comments: 0

Question Number 72247 Answers: 1 Comments: 0

Question Number 72232 Answers: 0 Comments: 6

to all those who deleted their posts after they had been answered: I will not answer you anymore this forum had been great but lately it has been filling with unpolite people I′m not a freebie solver for anybody′s homework

$$\mathrm{to}\:\mathrm{all}\:\mathrm{those}\:\mathrm{who}\:\mathrm{deleted}\:\mathrm{their}\:\mathrm{posts}\:\mathrm{after}\:\mathrm{they} \\ $$$$\mathrm{had}\:\mathrm{been}\:\mathrm{answered}:\:\mathrm{I}\:\mathrm{will}\:\mathrm{not}\:\mathrm{answer}\:\mathrm{you} \\ $$$$\mathrm{anymore} \\ $$$$\mathrm{this}\:\mathrm{forum}\:\mathrm{had}\:\mathrm{been}\:\mathrm{great}\:\mathrm{but}\:\mathrm{lately}\:\mathrm{it}\:\mathrm{has} \\ $$$$\mathrm{been}\:\mathrm{filling}\:\mathrm{with}\:\mathrm{unpolite}\:\mathrm{people} \\ $$$$\mathrm{I}'\mathrm{m}\:\mathrm{not}\:\mathrm{a}\:\mathrm{freebie}\:\mathrm{solver}\:\mathrm{for}\:\mathrm{anybody}'\mathrm{s}\:\mathrm{homework} \\ $$

Question Number 72251 Answers: 0 Comments: 1

Question Number 72250 Answers: 0 Comments: 2

f(x)=(ax+b)sin(x)+(cx+d)cos(x) and (dy/dx)=xcos(x) find a , b , c , d

$${f}\left({x}\right)=\left({ax}+{b}\right){sin}\left({x}\right)+\left({cx}+{d}\right){cos}\left({x}\right) \\ $$$${and}\:\:\:\frac{{dy}}{{dx}}={xcos}\left({x}\right) \\ $$$${find}\:\:{a}\:,\:{b}\:,\:{c}\:,\:{d} \\ $$$$ \\ $$$$ \\ $$

Question Number 72248 Answers: 1 Comments: 1

Question Number 72287 Answers: 0 Comments: 0

Question Number 72218 Answers: 0 Comments: 3

Question Number 72198 Answers: 1 Comments: 8

Pg 1383 Pg 1384 Pg 1385 Pg 1386 Pg 1387 Pg 1388 Pg 1389 Pg 1390 Pg 1391 Pg 1392

Contact: info@tinkutara.com