
AllQuestion and Answers: Page 1387
Question Number 73048 Answers: 0 Comments: 0
Question Number 73047 Answers: 1 Comments: 0
Question Number 73046 Answers: 1 Comments: 0
Question Number 73045 Answers: 0 Comments: 0
Question Number 73044 Answers: 0 Comments: 0
Question Number 73043 Answers: 1 Comments: 2
Question Number 73042 Answers: 1 Comments: 0
Question Number 73041 Answers: 1 Comments: 1
Question Number 73040 Answers: 1 Comments: 0
Question Number 73039 Answers: 1 Comments: 0
Question Number 73037 Answers: 1 Comments: 1
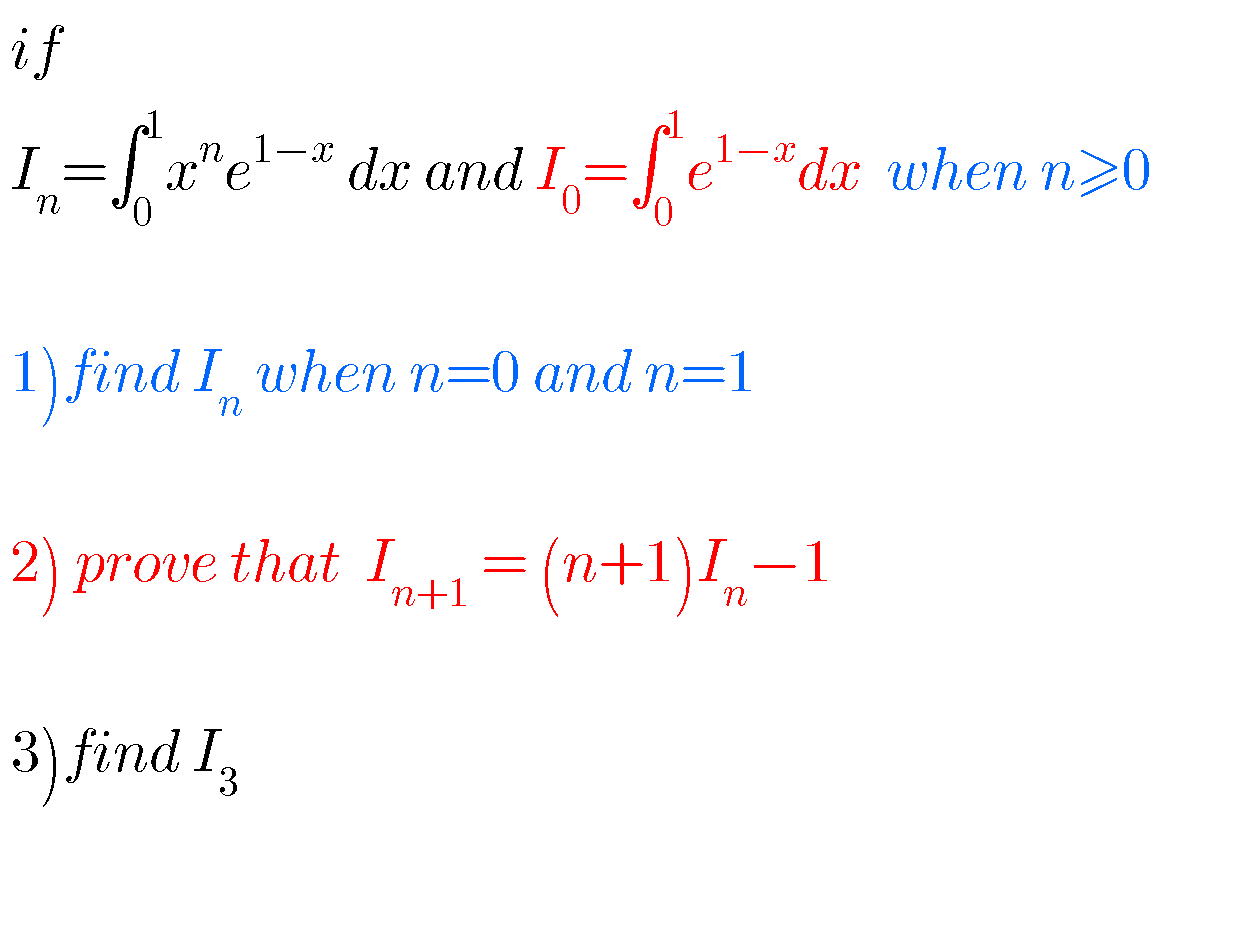
Question Number 73036 Answers: 1 Comments: 3
Question Number 73035 Answers: 0 Comments: 0
Question Number 73034 Answers: 1 Comments: 1
Question Number 73033 Answers: 1 Comments: 0
Question Number 73032 Answers: 1 Comments: 0
Question Number 73031 Answers: 0 Comments: 0
Question Number 73030 Answers: 2 Comments: 0

Question Number 73029 Answers: 1 Comments: 0
Question Number 73028 Answers: 2 Comments: 0
Question Number 73027 Answers: 1 Comments: 0
Question Number 73021 Answers: 0 Comments: 0
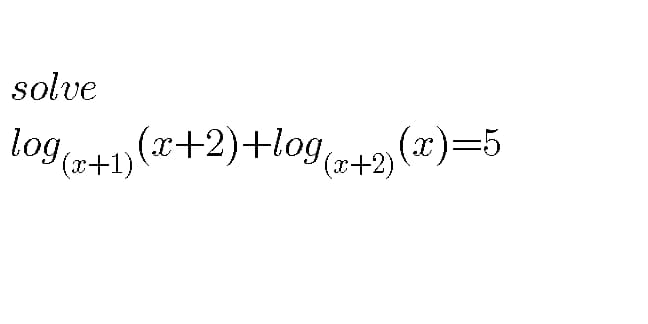
Question Number 73017 Answers: 0 Comments: 1
Question Number 73084 Answers: 1 Comments: 0

Question Number 72998 Answers: 0 Comments: 0
Question Number 72997 Answers: 1 Comments: 0
Pg 1382 Pg 1383 Pg 1384 Pg 1385 Pg 1386 Pg 1387 Pg 1388 Pg 1389 Pg 1390 Pg 1391
