
AllQuestion and Answers: Page 1385
Question Number 73273 Answers: 1 Comments: 0

Question Number 73269 Answers: 0 Comments: 0

Question Number 73260 Answers: 1 Comments: 0

Question Number 73255 Answers: 1 Comments: 0

Question Number 73247 Answers: 1 Comments: 0

Question Number 73243 Answers: 1 Comments: 0
Question Number 73238 Answers: 1 Comments: 1
Question Number 73235 Answers: 0 Comments: 0

Question Number 73261 Answers: 1 Comments: 1
Question Number 73231 Answers: 1 Comments: 1
Question Number 73230 Answers: 0 Comments: 0
Question Number 73227 Answers: 1 Comments: 0
Question Number 73225 Answers: 0 Comments: 0
Question Number 73224 Answers: 1 Comments: 0
Question Number 73223 Answers: 0 Comments: 5
Question Number 73222 Answers: 1 Comments: 0

Question Number 73221 Answers: 1 Comments: 0

Question Number 73210 Answers: 1 Comments: 2

Question Number 73203 Answers: 0 Comments: 1

Question Number 73202 Answers: 2 Comments: 3
Question Number 73200 Answers: 0 Comments: 4
Question Number 73258 Answers: 1 Comments: 0

Question Number 73191 Answers: 1 Comments: 0
Question Number 73251 Answers: 1 Comments: 0
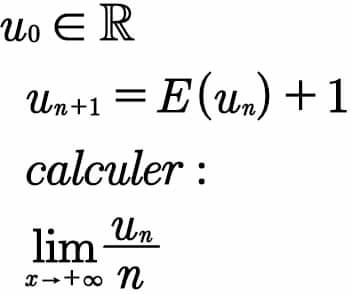
Question Number 73188 Answers: 0 Comments: 0

Question Number 73182 Answers: 0 Comments: 3
Pg 1380 Pg 1381 Pg 1382 Pg 1383 Pg 1384 Pg 1385 Pg 1386 Pg 1387 Pg 1388 Pg 1389
