Question and Answers Forum

AllQuestion and Answers: Page 1384

Question Number 72888 Answers: 1 Comments: 5

let f(x)=∫_(π/6) ^(π/4) ((tant)/(2+x cost))dt with x real 1)determine a explicit form for f(x) 2)determine also g(x)=∫_(π/6) ^(π/4) ((tant)/((2+xcost)^2 ))dx 3) find the value of ∫_(π/6) ^(π/4) ((tant)/((2+3cost)))dt and ∫_(π/6) ^(π/4) ((tant)/((2+3cost)^2 ))dt

Question Number 72886 Answers: 1 Comments: 0

let w=f(x, y) be a differentiable function where x=rcosθ and y=rsinθ show that (f_x )^2 +(f_y )^2 =(w_x )^2 +1/r^2 (w_y )^2 ? help me sir

$${let}\:{w}={f}\left({x},\:{y}\right)\:{be}\:{a}\:{differentiable}\:{function}\:{where}\:{x}={rcos}\theta\:{and}\:{y}={rsin}\theta\:{show}\:{that}\:\left({f}_{{x}} \right)^{\mathrm{2}} +\left({f}_{{y}} \right)^{\mathrm{2}} =\left({w}_{{x}} \right)^{\mathrm{2}} +\mathrm{1}/{r}^{\mathrm{2}} \left({w}_{{y}} \right)^{\mathrm{2}} ? \\ $$$${help}\:{me}\:{sir}\: \\ $$

Question Number 72884 Answers: 0 Comments: 1

find the area of the region bounded by the semicircle y=(√(a^2 −x^2 )) and the x=+−a and the line y=−a ? by using intigiral pleas sir help me

$${find}\:{the}\:{area}\:{of}\:{the}\:{region}\:{bounded}\:{by}\:{the}\:{semicircle}\:{y}=\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }\:{and}\:{the}\:{x}=+−{a}\:\:{and}\:{the}\:{line}\:{y}=−{a}\:?\:{by}\:{using}\:{intigiral} \\ $$$${pleas}\:{sir}\:{help}\:{me} \\ $$

Question Number 72883 Answers: 1 Comments: 0

if w=f(u and v) where f_(uu) +f_(vv) =0 and u=(x^2 −y^2 )/2 and v=xy show that w_(xx) +w_(yy) =0 ? pleas sir help me

$${if}\:{w}={f}\left({u}\:{and}\:{v}\right)\:{where}\:{f}_{{uu}} +{f}_{{vv}} =\mathrm{0}\:{and}\:{u}=\left({x}^{\mathrm{2}} −{y}^{\mathrm{2}} \right)/\mathrm{2}\:{and}\:{v}={xy}\:{show}\:{that}\:{w}_{{xx}} +{w}_{{yy}} =\mathrm{0}\:? \\ $$$${pleas}\:{sir}\:{help}\:{me} \\ $$

Question Number 72889 Answers: 1 Comments: 1

calculate Σ_(n=1) ^∞ (((−1)^n )/((2n+1)n^2 ))

$${calculate}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left(\mathrm{2}{n}+\mathrm{1}\right){n}^{\mathrm{2}} } \\ $$

Question Number 72880 Answers: 0 Comments: 0

Question Number 72877 Answers: 0 Comments: 1

by using theorem demwover find x^4 =1? pleas sir help me

$${by}\:{using}\:{theorem}\:{demwover}\:{find}\:\:{x}^{\mathrm{4}} =\mathrm{1}? \\ $$$${pleas}\:{sir}\:{help}\:{me} \\ $$

Question Number 72871 Answers: 1 Comments: 0

Question Number 72863 Answers: 0 Comments: 1

Question Number 72905 Answers: 2 Comments: 3

f(x)≥0, and lim_(x→a) f(x)=0,lim_(x→a) g(x)=∞ then lim_(x→a) f(x)^(g(x)) =?

$${f}\left({x}\right)\geqslant\mathrm{0},\:{and}\:\underset{{x}\rightarrow{a}} {\mathrm{lim}}{f}\left({x}\right)=\mathrm{0},\underset{{x}\rightarrow{a}} {\mathrm{lim}}{g}\left({x}\right)=\infty \\ $$$${then}\:\underset{{x}\rightarrow{a}} {\mathrm{lim}}{f}\left({x}\right)^{{g}\left({x}\right)} =? \\ $$

Question Number 72894 Answers: 1 Comments: 0

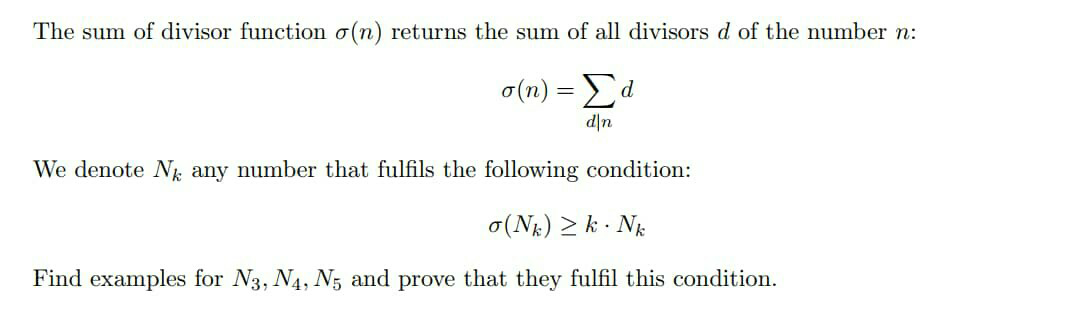
Question Number 72841 Answers: 1 Comments: 3

Could someone help me on this question? Knowing that the area of a circle segment is given by A=R^2 (θ−sinθ)/2. Where A=7m^2 ; R^2 =((28)/π). What is the best answer for the angle value (degree) a) 85°<θ<90° b) 95°<θ<100° c) 105°<θ<110° d) 115°<θ<120° e) 125°<θ<135°

$${Could}\:{someone}\:{help}\:{me}\:{on}\:{this}\:{question}? \\ $$$${Knowing}\:{that}\:{the}\:{area}\:{of}\:{a}\:{circle}\:{segment}\:{is}\:{given}\:{by}\:{A}={R}^{\mathrm{2}} \left(\theta−{sin}\theta\right)/\mathrm{2}.\:{Where}\:{A}=\mathrm{7}{m}^{\mathrm{2}} ;\:{R}^{\mathrm{2}} =\frac{\mathrm{28}}{\pi}. \\ $$$${What}\:{is}\:{the}\:{best}\:{answer}\:{for}\:{the}\:{angle}\:{value}\:\left({degree}\right) \\ $$$$\left.{a}\right)\:\mathrm{85}°<\theta<\mathrm{90}° \\ $$$$\left.{b}\right)\:\mathrm{95}°<\theta<\mathrm{100}° \\ $$$$\left.{c}\right)\:\mathrm{105}°<\theta<\mathrm{110}° \\ $$$$\left.{d}\right)\:\mathrm{115}°<\theta<\mathrm{120}° \\ $$$$\left.{e}\right)\:\mathrm{125}°<\theta<\mathrm{135}° \\ $$

Question Number 72838 Answers: 0 Comments: 1

given that f(x) = ((∣x −2∣)/(1−∣x∣)) check if f is continuous a x = 2 hence write f(x) as a pairwise function

$${given}\:{that}\:\:\:{f}\left({x}\right)\:=\:\frac{\mid{x}\:−\mathrm{2}\mid}{\mathrm{1}−\mid{x}\mid} \\ $$$${check}\:{if}\:{f}\:{is}\:{continuous}\:{a}\:{x}\:=\:\mathrm{2} \\ $$$${hence}\:\:{write}\:{f}\left({x}\right)\:{as}\:{a}\:{pairwise}\:{function}\: \\ $$

Question Number 72837 Answers: 0 Comments: 1

find lim_(x→0) x + [x]

$${find}\: \\ $$$$\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:{x}\:+\:\left[{x}\right] \\ $$

Question Number 72836 Answers: 1 Comments: 1

Question Number 72835 Answers: 1 Comments: 0

In an arithmetic progression the ninth term is greater than the second term and the sum of the first term with the fifth term is 20. What is the fifth term?

$${In}\:{an}\:{arithmetic}\:{progression}\:{the} \\ $$$${ninth}\:{term}\:{is}\:{greater}\:{than}\:{the}\:{second} \\ $$$${term}\:{and}\:{the}\:{sum}\:{of}\:{the}\:{first}\:{term} \\ $$$${with}\:{the}\:{fifth}\:{term}\:{is}\:\mathrm{20}.\:{What}\:{is} \\ $$$${the}\:{fifth}\:{term}? \\ $$

Question Number 72832 Answers: 1 Comments: 1

In a parallelogram OABC, OA^⇁ =a^(−⇁) , OC^→ =c^→ , D is a point such that AD^→ :DB^→ =1:2 Express the following in terms of a and c (i)CB^→ (ii)BC^→ (iii)AB^→ (iv) AD^→ (v)OD^→ (vi)DC^→

Question Number 72824 Answers: 1 Comments: 0

fnd all integers n for which 13∣ 4(n^2 + 1)

$${fnd}\:{all}\:{integers}\:{n}\:{for}\:{which}\: \\ $$$$\:\mathrm{13}\mid\:\mathrm{4}\left({n}^{\mathrm{2}} \:+\:\mathrm{1}\right) \\ $$

Question Number 72823 Answers: 1 Comments: 0

What is derivative for this function a×b^(x−1) ×c^((1/2)(x−1)(x−2)) ×d^((1/6)(x−1)(x−2)(x−3))

$$\mathrm{What}\:\mathrm{is}\:\mathrm{derivative}\:\mathrm{for}\:\mathrm{this}\:\mathrm{function} \\ $$$$\: \\ $$$${a}×{b}^{{x}−\mathrm{1}} ×{c}^{\frac{\mathrm{1}}{\mathrm{2}}\left({x}−\mathrm{1}\right)\left({x}−\mathrm{2}\right)} ×{d}^{\frac{\mathrm{1}}{\mathrm{6}}\left({x}−\mathrm{1}\right)\left({x}−\mathrm{2}\right)\left({x}−\mathrm{3}\right)} \\ $$

Question Number 72934 Answers: 1 Comments: 0

Question Number 72813 Answers: 0 Comments: 3

Find the area of the region enclosed by the line 5y=x+6 and the curve y=(√(∣x∣)) .

$${Find}\:{the}\:{area}\:{of}\:{the}\:{region}\:{enclosed} \\ $$$${by}\:{the}\:{line}\:\mathrm{5}{y}={x}+\mathrm{6}\:{and}\:{the}\:{curve} \\ $$$${y}=\sqrt{\mid{x}\mid}\:. \\ $$

Question Number 72806 Answers: 1 Comments: 2

show that lim_( x→0) [ x] does not exist. Hence define [x] and sketch a graph for y = 3x^2 + [x]

$$\underset{\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{x}\rightarrow\mathrm{0}} {\:{show}\:{that}\:\:\mathrm{lim}}\:\left[\:{x}\right]\:\:{does}\:{not}\:{exist}. \\ $$$${Hence}\:{define}\:\:\left[{x}\right]\:\:{and}\:{sketch}\:{a}\:{graph}\:{for}\: \\ $$$$\:{y}\:=\:\mathrm{3}{x}^{\mathrm{2}} \:+\:\left[{x}\right] \\ $$

Question Number 72805 Answers: 0 Comments: 0

PARTIAL VARIATION The success rate of government variws inversly as the number of corrupt mi nded individual and varies directly as the number of clean minded individal .if the goverment attain 95% success rate when there are two corrupt minded and 75% success rate when there are 5 corrupt minded and 20 clean minded individual. How many corrupr minded individual must be in administration with one clean minded individual to attain 99% success rate?

$${PARTIAL}\:{VARIATION} \\ $$$${The}\:{success}\:{rate}\:{of}\:{government}\:{variws}\: \\ $$$${inversly}\:{as}\:{the}\:{number}\:{of}\:{corrupt}\:{mi} \\ $$$${nded}\:{individual}\:{and}\:{varies}\:{directly} \\ $$$${as}\:{the}\:{number}\:{of}\:{clean}\:{minded}\:{individal} \\ $$$$.{if}\:\:{the}\:{goverment}\:{attain}\:\mathrm{95\%}\:{success} \\ $$$${rate}\:{when}\:{there}\:{are}\:{two}\:{corrupt}\:{minded} \\ $$$${and}\:\mathrm{75\%}\:{success}\:{rate}\:{when}\:{there}\:{are} \\ $$$$\mathrm{5}\:{corrupt}\:{minded}\:{and}\:\mathrm{20}\:{clean}\:{minded} \\ $$$${individual}.\:{How}\:{many}\:{corrupr}\:{minded} \\ $$$${individual}\:{must}\:{be}\:{in}\:{administration}\: \\ $$$${with}\:{one}\:{clean}\:{minded}\:{individual}\:{to}\: \\ $$$${attain}\:\mathrm{99\%}\:{success}\:{rate}? \\ $$

Question Number 72803 Answers: 2 Comments: 1

Question Number 72796 Answers: 1 Comments: 0

Integrate f(x,y)=(1/((1+x^2 +y^2 )^2 )) over the triangle with vertices (0,0) ,(1,0), (1,(√3)) after changing it to polar form.

$${Integrate}\:{f}\left({x},{y}\right)=\frac{\mathrm{1}}{\left(\mathrm{1}+{x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right)^{\mathrm{2}} }\:{over} \\ $$$${the}\:{triangle}\:{with}\:{vertices}\:\left(\mathrm{0},\mathrm{0}\right)\:,\left(\mathrm{1},\mathrm{0}\right), \\ $$$$\left(\mathrm{1},\sqrt{\mathrm{3}}\right)\:{after}\:{changing}\:{it}\:{to}\:{polar}\:{form}. \\ $$

Question Number 72789 Answers: 1 Comments: 4

Find the area of the surface generated by revolving the curve x=(y^4 /4)+(1/(8y^2 )) about the x−axis . (given:1≤y≤2)

$${Find}\:{the}\:{area}\:{of}\:{the}\:{surface}\:{generated} \\ $$$${by}\:{revolving}\:{the}\:{curve}\:{x}=\frac{{y}^{\mathrm{4}} }{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{8}{y}^{\mathrm{2}} }\: \\ $$$${about}\:{the}\:{x}−{axis}\:.\:\left({given}:\mathrm{1}\leqslant{y}\leqslant\mathrm{2}\right) \\ $$

Pg 1379 Pg 1380 Pg 1381 Pg 1382 Pg 1383 Pg 1384 Pg 1385 Pg 1386 Pg 1387 Pg 1388

Contact: info@tinkutara.com