Question and Answers Forum

AllQuestion and Answers: Page 1383

Question Number 63784 Answers: 0 Comments: 6

question 63639 again prove: ∀z∈C: ∣z+1∣+∣z^2 +z+1∣+∣z^3 +1∣≥1

$$\mathrm{question}\:\mathrm{63639}\:\mathrm{again} \\ $$$$\mathrm{prove}: \\ $$$$\forall{z}\in\mathbb{C}:\:\mid{z}+\mathrm{1}\mid+\mid{z}^{\mathrm{2}} +{z}+\mathrm{1}\mid+\mid{z}^{\mathrm{3}} +\mathrm{1}\mid\geqslant\mathrm{1} \\ $$

Question Number 63769 Answers: 1 Comments: 1

Question Number 63782 Answers: 1 Comments: 4

let f(a) =∫_(−∞) ^(+∞) (dx/((a^2 +x^2 )^3 )) with a>0 1) calculate f(a) 2)calculste also g(a) =∫_(−∞) ^(+∞) (dx/((a^2 +x^2 )^4 )) 3) find the values of integrals ∫_0 ^∞ (dx/((x^2 +1)^3 )) ∫_0 ^∞ (dx/((x^2 +2)^4 ))

$${let}\:{f}\left({a}\right)\:=\int_{−\infty} ^{+\infty} \:\:\:\frac{{dx}}{\left({a}^{\mathrm{2}} +{x}^{\mathrm{2}} \right)^{\mathrm{3}} }\:\:\:{with}\:{a}>\mathrm{0} \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}\left({a}\right) \\ $$$$\left.\mathrm{2}\right){calculste}\:{also}\:{g}\left({a}\right)\:=\int_{−\infty} ^{+\infty} \:\:\:\frac{{dx}}{\left({a}^{\mathrm{2}} \:+{x}^{\mathrm{2}} \right)^{\mathrm{4}} } \\ $$$$\left.\mathrm{3}\right)\:{find}\:{the}\:{values}\:{of}\:{integrals}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{2}\right)^{\mathrm{4}} } \\ $$

Question Number 63812 Answers: 0 Comments: 2

A can terminate a work 9 hour earlier than B. A and B terminate that work after 20 hour together. A can terminate that work after .... hour.

$$ \\ $$$${A}\:\:{can}\:{terminate}\:{a}\:{work}\:\:\mathrm{9}\:{hour}\:{earlier} \\ $$$${than}\:{B}. \\ $$$${A}\:\:{and}\:\:{B}\:\:{terminate}\:{that}\:{work}\:\:{after}\:\mathrm{20}\:{hour}\:{together}. \\ $$$${A}\:{can}\:{terminate}\:{that}\:{work}\:{after}\:....\:{hour}. \\ $$$$ \\ $$$$ \\ $$

Question Number 63823 Answers: 0 Comments: 0

calculate ∫_(−∞) ^(+∞) ((3x^2 −1)/(x^4 −2x^2 +3))dx

$${calculate}\:\:\int_{−\infty} ^{+\infty} \:\:\frac{\mathrm{3}{x}^{\mathrm{2}} −\mathrm{1}}{{x}^{\mathrm{4}} −\mathrm{2}{x}^{\mathrm{2}} \:+\mathrm{3}}{dx} \\ $$

Question Number 63763 Answers: 0 Comments: 1

Question Number 63758 Answers: 0 Comments: 6

Tanmay Sir. Are you ok ?

$$\mathrm{Tanmay}\:\mathrm{Sir}.\:\mathrm{Are}\:\mathrm{you}\:\mathrm{ok}\:? \\ $$

Question Number 63751 Answers: 0 Comments: 0

Where is sir tanmay

$$\mathrm{Where}\:\mathrm{is}\:\mathrm{sir}\:\mathrm{tanmay} \\ $$

Question Number 63790 Answers: 2 Comments: 0

Question Number 63789 Answers: 0 Comments: 0

Question Number 63788 Answers: 1 Comments: 0

Question Number 63750 Answers: 1 Comments: 2

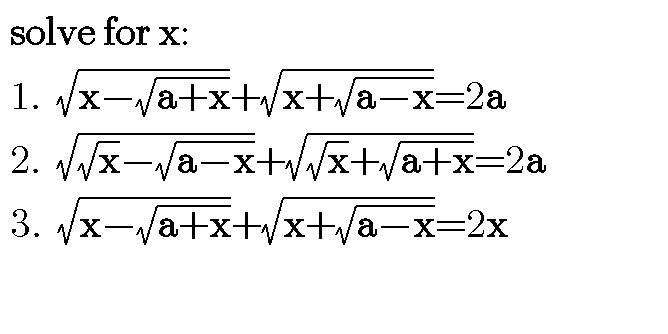
Question Number 63748 Answers: 0 Comments: 0

Question Number 63747 Answers: 0 Comments: 1

What does sin^(−2) x mean?

$${What}\:{does}\:{sin}^{−\mathrm{2}} {x}\:{mean}? \\ $$

Question Number 63792 Answers: 0 Comments: 0

Question Number 63738 Answers: 0 Comments: 0

useful formula ======== ∀a∈R^+ :∀b ∈R: a sin x +b cos x =(√(a^2 +b^2 ))sin (x+arctan (b/a)) ∫(dx/(a sin x +b cos x))= =(1/(√(a^2 +b^2 )))∫(dx/(sin (x+arctan (b/a))))= [t=x+arctan (b/a) → dx=dt] (1/(√(a^2 +b^2 )))∫(dt/(sin t))=−(1/(√(a^2 +b^2 )))ln ((1/(sin t))+(1/(tan t))) = =−(1/(√(a^2 +b^2 )))ln ∣(((√(a^2 +b^2 ))−b sin x +a cos x)/(a sin x +b cos x))∣ +C

$$\mathrm{useful}\:\mathrm{formula} \\ $$$$======== \\ $$$$ \\ $$$$\forall{a}\in\mathbb{R}^{+} :\forall{b}\:\in\mathbb{R}:\:{a}\:\mathrm{sin}\:{x}\:+{b}\:\mathrm{cos}\:{x}\:=\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }\mathrm{sin}\:\left({x}+\mathrm{arctan}\:\frac{{b}}{{a}}\right) \\ $$$$\int\frac{{dx}}{{a}\:\mathrm{sin}\:{x}\:+{b}\:\mathrm{cos}\:{x}}= \\ $$$$=\frac{\mathrm{1}}{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\int\frac{{dx}}{\mathrm{sin}\:\left({x}+\mathrm{arctan}\:\frac{{b}}{{a}}\right)}= \\ $$$$\:\:\:\:\:\left[{t}={x}+\mathrm{arctan}\:\frac{{b}}{{a}}\:\:\rightarrow\:{dx}={dt}\right] \\ $$$$\frac{\mathrm{1}}{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\int\frac{{dt}}{\mathrm{sin}\:{t}}=−\frac{\mathrm{1}}{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\mathrm{ln}\:\left(\frac{\mathrm{1}}{\mathrm{sin}\:{t}}+\frac{\mathrm{1}}{\mathrm{tan}\:{t}}\right)\:= \\ $$$$=−\frac{\mathrm{1}}{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }}\mathrm{ln}\:\mid\frac{\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }−{b}\:\mathrm{sin}\:{x}\:+{a}\:\mathrm{cos}\:{x}}{{a}\:\mathrm{sin}\:{x}\:+{b}\:\mathrm{cos}\:{x}}\mid\:+{C} \\ $$

Question Number 63722 Answers: 1 Comments: 6

1) calculate ∫ (x^2 −x+2)(√(x^2 −x+1))dx 2)find the value of ∫_0 ^1 (x^2 −x+2)(√(x^2 −x +1))dx .

$$\left.\mathrm{1}\right)\:{calculate}\:\int\:\left({x}^{\mathrm{2}} −{x}+\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}{dx} \\ $$$$\left.\mathrm{2}\right){find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\mathrm{1}} \left({x}^{\mathrm{2}} −{x}+\mathrm{2}\right)\sqrt{{x}^{\mathrm{2}} −{x}\:+\mathrm{1}}{dx}\:. \\ $$

Question Number 63721 Answers: 1 Comments: 2

calculate ∫ (dx/(√((x−1)(2−x))))

$${calculate}\:\int\:\:\frac{{dx}}{\sqrt{\left({x}−\mathrm{1}\right)\left(\mathrm{2}−{x}\right)}} \\ $$

Question Number 63720 Answers: 1 Comments: 2

calculate ∫(√((x−3)(2−x)))dx

$${calculate}\:\int\sqrt{\left({x}−\mathrm{3}\right)\left(\mathrm{2}−{x}\right)}{dx} \\ $$

Question Number 63711 Answers: 1 Comments: 1

calculate ∫_0 ^π (dx/((√3)cosx +(√2)sinx))