Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1372

Question Number 74649 Answers: 2 Comments: 1

Question Number 74647 Answers: 0 Comments: 2

Question Number 74639 Answers: 0 Comments: 4

$${If} \\ $$

Question Number 74634 Answers: 0 Comments: 6

Question Number 74632 Answers: 0 Comments: 1

$$. \\ $$

Question Number 74623 Answers: 1 Comments: 1

Question Number 74622 Answers: 0 Comments: 4

Expand 1+(2/3)∙(Σ_(k=1) ^(n−1) [cos(((2π)/3)x)]+2n−2)

$$\mathrm{Expand} \\ $$$$\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\centerdot\left(\underset{{k}=\mathrm{1}} {\overset{{n}−\mathrm{1}} {\sum}}\left[{cos}\left(\frac{\mathrm{2}\pi}{\mathrm{3}}{x}\right)\right]+\mathrm{2}{n}−\mathrm{2}\right) \\ $$

Question Number 74621 Answers: 1 Comments: 1

Question Number 74620 Answers: 1 Comments: 0

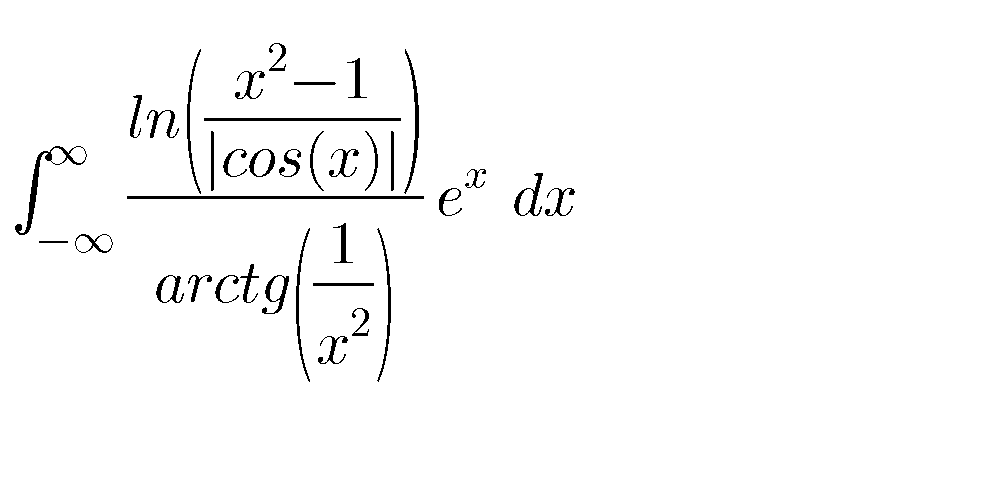
Question Number 74594 Answers: 1 Comments: 1

Question Number 74591 Answers: 0 Comments: 0

Question Number 74590 Answers: 1 Comments: 0

Question Number 74589 Answers: 1 Comments: 0

Question Number 74582 Answers: 1 Comments: 0

Find all values of x: (2^x )^(x^2 −8) =32

$${Find}\:{all}\:{values}\:{of}\:{x}: \\ $$$$\left(\mathrm{2}^{{x}} \right)^{{x}^{\mathrm{2}} −\mathrm{8}} =\mathrm{32} \\ $$

Question Number 74580 Answers: 1 Comments: 0

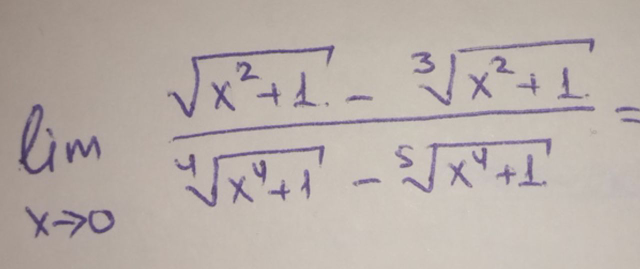
Question Number 74579 Answers: 0 Comments: 0

find the gradient of scalar point function being expressed in term of scalar triple product as u=(a^ ,b^ ,c^ )=a^ .b^ ×c^

$${find}\:{the}\:{gradient}\:{of}\:{scalar}\:{point}\:{function}\:{being}\:{expressed}\:{in}\:{term}\:{of}\:{scalar}\:{triple}\:{product}\:{as}\:{u}=\left(\bar {{a}},\bar {{b}},\bar {{c}}\right)=\bar {{a}}.\bar {{b}}×\bar {{c}} \\ $$

Question Number 74573 Answers: 1 Comments: 1

Find (turn it into non-segma expression) 1+Σ_(k=1) ^(n−1) (((−1)^k +3)/2)

$$\mathrm{Find}\:\left(\mathrm{turn}\:\mathrm{it}\:\mathrm{into}\:\mathrm{non}-\mathrm{segma}\:\mathrm{expression}\right) \\ $$$$\mathrm{1}+\underset{{k}=\mathrm{1}} {\overset{{n}−\mathrm{1}} {\sum}}\frac{\left(−\mathrm{1}\right)^{{k}} +\mathrm{3}}{\mathrm{2}} \\ $$

Question Number 74604 Answers: 0 Comments: 3

$$. \\ $$

Question Number 74601 Answers: 0 Comments: 0

solve y′′+ a(x)y=b(x) the general form of the solution if possible or juzt a solving metbod

$${solve}\:\:\:{y}''+\:{a}\left({x}\right){y}={b}\left({x}\right)\: \\ $$$${the}\:\:{general}\:\:{form}\:{of}\:\:{the}\:{solution}\:{if}\:\:{possible} \\ $$$${or}\:\:{juzt}\:{a}\:{solving}\:{metbod} \\ $$

Question Number 74600 Answers: 1 Comments: 0

Question Number 74599 Answers: 0 Comments: 0

Hello,verry Nice day let U_n =E((((3+(√(17)))/2))^n ),n∈N^∗ show that U_n ≡n(2)

$$\mathrm{Hello},\mathrm{verry}\:\mathrm{Nice}\:\mathrm{day}\: \\ $$$$\mathrm{let}\:\mathrm{U}_{\mathrm{n}} =\mathrm{E}\left(\left(\frac{\mathrm{3}+\sqrt{\mathrm{17}}}{\mathrm{2}}\right)^{\mathrm{n}} \right),\mathrm{n}\in\mathbb{N}^{\ast} \\ $$$$\mathrm{show}\:\mathrm{that}\:\mathrm{U}_{\mathrm{n}} \equiv\mathrm{n}\left(\mathrm{2}\right) \\ $$

Question Number 74570 Answers: 1 Comments: 0

lim_(n→∞) (1/((n)^(1/n) )) = ?

$${lim}_{{n}\rightarrow\infty} \frac{\mathrm{1}}{\left({n}\right)^{\frac{\mathrm{1}}{{n}}} }\:=\:? \\ $$

Question Number 74617 Answers: 0 Comments: 0

Expand 1+Σ_(k=1) ^(n−1) [∣((2(√3))/3)sin(120x)∣+2]

$$\mathrm{Expand} \\ $$$$\mathrm{1}+\underset{{k}=\mathrm{1}} {\overset{{n}−\mathrm{1}} {\sum}}\left[\mid\frac{\mathrm{2}\sqrt{\mathrm{3}}}{\mathrm{3}}{sin}\left(\mathrm{120}{x}\right)\mid+\mathrm{2}\right] \\ $$

Question Number 74615 Answers: 2 Comments: 0

Question Number 74614 Answers: 1 Comments: 0

Question Number 74611 Answers: 1 Comments: 0

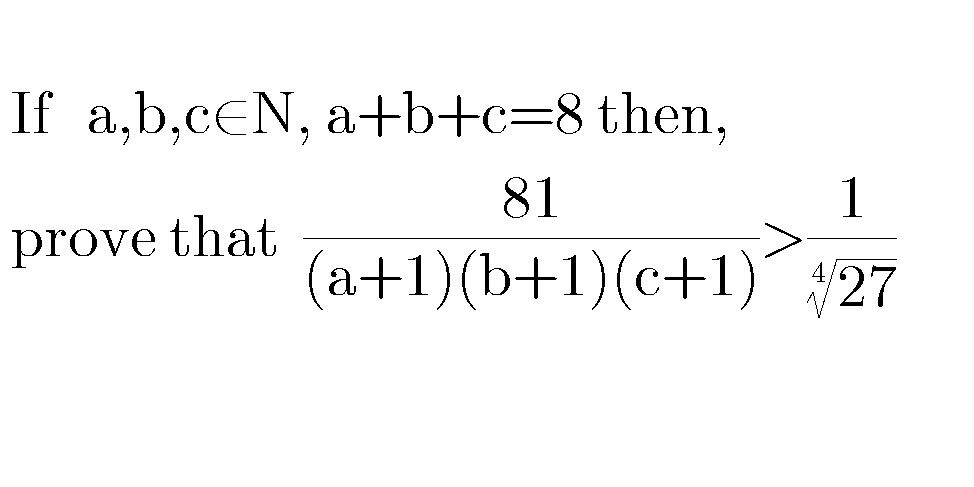
Pg 1367 Pg 1368 Pg 1369 Pg 1370 Pg 1371 Pg 1372 Pg 1373 Pg 1374 Pg 1375 Pg 1376

Contact: info@tinkutara.com