
AllQuestion and Answers: Page 1371
Question Number 72835 Answers: 1 Comments: 0
Question Number 72832 Answers: 1 Comments: 1
Question Number 72824 Answers: 1 Comments: 0
Question Number 72823 Answers: 1 Comments: 0
Question Number 72934 Answers: 1 Comments: 0

Question Number 72813 Answers: 0 Comments: 3
Question Number 72806 Answers: 1 Comments: 2
Question Number 72805 Answers: 0 Comments: 0
Question Number 72803 Answers: 2 Comments: 1

Question Number 72796 Answers: 1 Comments: 0
Question Number 72789 Answers: 1 Comments: 4
Question Number 72787 Answers: 1 Comments: 1
Question Number 72784 Answers: 1 Comments: 1
Question Number 72782 Answers: 1 Comments: 1

Question Number 72775 Answers: 0 Comments: 0

Question Number 72773 Answers: 1 Comments: 2

Question Number 72746 Answers: 1 Comments: 0
Question Number 72744 Answers: 0 Comments: 0

Question Number 72741 Answers: 0 Comments: 1

Question Number 72734 Answers: 3 Comments: 0
Question Number 72724 Answers: 0 Comments: 2

Question Number 72721 Answers: 1 Comments: 12

Question Number 72718 Answers: 0 Comments: 3
Question Number 72689 Answers: 1 Comments: 0
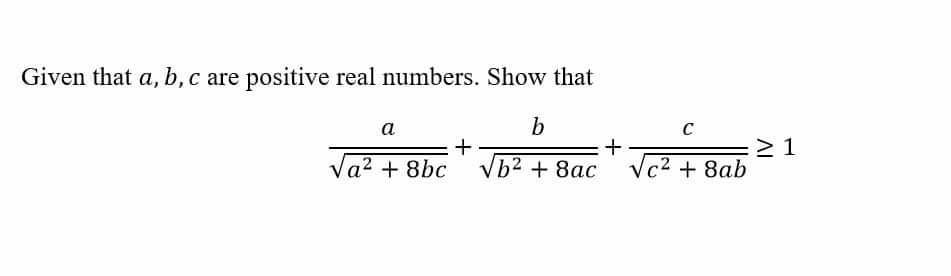
Question Number 72688 Answers: 3 Comments: 0
Question Number 72672 Answers: 2 Comments: 2

Pg 1366 Pg 1367 Pg 1368 Pg 1369 Pg 1370 Pg 1371 Pg 1372 Pg 1373 Pg 1374 Pg 1375
