Question and Answers Forum

AllQuestion and Answers: Page 1370

Question Number 74900 Answers: 0 Comments: 2

Question Number 74910 Answers: 1 Comments: 0

Explain a function with examples based on our daily life ?

$$\mathrm{Explain}\:\mathrm{a}\:\mathrm{function}\:\mathrm{with}\:\mathrm{examples}\:\mathrm{based} \\ $$$$\mathrm{on}\:\mathrm{our}\:\mathrm{daily}\:\mathrm{life}\:? \\ $$

Question Number 74891 Answers: 0 Comments: 4

Q. How will you define integrating constant C ? In how many ways can you define C ?

$$\mathrm{Q}.\:\mathrm{How}\:\mathrm{will}\:\mathrm{you}\:\mathrm{define}\:\mathrm{integrating}\: \\ $$$$\mathrm{constant}\:\mathrm{C}\:?\:\mathrm{In}\:\mathrm{how}\:\mathrm{many}\:\mathrm{ways}\:\mathrm{can}\:\mathrm{you} \\ $$$$\mathrm{define}\:\mathrm{C}\:? \\ $$$$ \\ $$

Question Number 74890 Answers: 1 Comments: 1

find ∫ (x+3)(√((x−1)(2−x)))dx

$${find}\:\int\:\:\:\left({x}+\mathrm{3}\right)\sqrt{\left({x}−\mathrm{1}\right)\left(\mathrm{2}−{x}\right)}{dx} \\ $$

Question Number 74889 Answers: 1 Comments: 1

find ∫_(−(1/2)) ^(+∞) e^(−x) (√(2x+1))dx

$${find}\:\int_{−\frac{\mathrm{1}}{\mathrm{2}}} ^{+\infty} \:\:{e}^{−{x}} \sqrt{\mathrm{2}{x}+\mathrm{1}}{dx} \\ $$

Question Number 74888 Answers: 1 Comments: 3

calculate f(α)=∫(√(x^2 −x+α))dx (α real)

$${calculate}\:{f}\left(\alpha\right)=\int\sqrt{{x}^{\mathrm{2}} −{x}+\alpha}{dx}\:\:\left(\alpha\:{real}\right) \\ $$

Question Number 74887 Answers: 0 Comments: 1

calculate Σ_(n=1) ^∞ (((−1)^n )/((n+1)n^3 ))

$${calculate}\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left({n}+\mathrm{1}\right){n}^{\mathrm{3}} } \\ $$

Question Number 74886 Answers: 0 Comments: 1

calculate ∫ ((x+1)/((x^3 +x−2)^2 ))dx

$${calculate}\:\int\:\:\frac{{x}+\mathrm{1}}{\left({x}^{\mathrm{3}} +{x}−\mathrm{2}\right)^{\mathrm{2}} }{dx} \\ $$

Question Number 74885 Answers: 1 Comments: 0

calcilate Σ_(n=1) ^(16) (1/n^3 )

$${calcilate}\:\sum_{{n}=\mathrm{1}} ^{\mathrm{16}} \:\frac{\mathrm{1}}{{n}^{\mathrm{3}} } \\ $$

Question Number 74884 Answers: 0 Comments: 2

calculate Σ_(n=1) ^(20) (1/n^2 )

$${calculate}\:\sum_{{n}=\mathrm{1}} ^{\mathrm{20}} \:\frac{\mathrm{1}}{{n}^{\mathrm{2}} } \\ $$$$ \\ $$

Question Number 74882 Answers: 1 Comments: 3

Question Number 74880 Answers: 1 Comments: 0

solve inR ((∣x+1∣))^(1/5) −((x^2 +4x−9))^(1/(10)) =(2x−10)(√(x^2 +1))

$${solve}\:{inR} \\ $$$$\sqrt[{\mathrm{5}}]{\mid{x}+\mathrm{1}\mid}−\sqrt[{\mathrm{10}}]{{x}^{\mathrm{2}} +\mathrm{4}{x}−\mathrm{9}}=\left(\mathrm{2}{x}−\mathrm{10}\right)\sqrt{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$

Question Number 74870 Answers: 1 Comments: 1

solve with explanation lim_(x→0^− ) [(x/(sinx))], where [ ] represents greatest integer

$$\mathrm{solve}\:\mathrm{with}\:\mathrm{explanation} \\ $$$$\mathrm{li}\underset{\mathrm{x}\rightarrow\mathrm{0}^{−} } {\mathrm{m}}\left[\frac{\mathrm{x}}{\mathrm{sinx}}\right],\:\mathrm{where}\:\left[\:\:\right]\:\mathrm{represents}\:\mathrm{greatest}\:\mathrm{integer} \\ $$

Question Number 74863 Answers: 2 Comments: 1

Question Number 76203 Answers: 0 Comments: 7

Question Number 74861 Answers: 1 Comments: 0

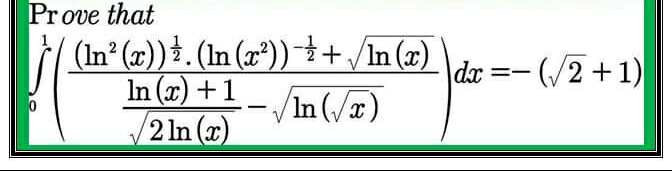
Question Number 74860 Answers: 1 Comments: 0

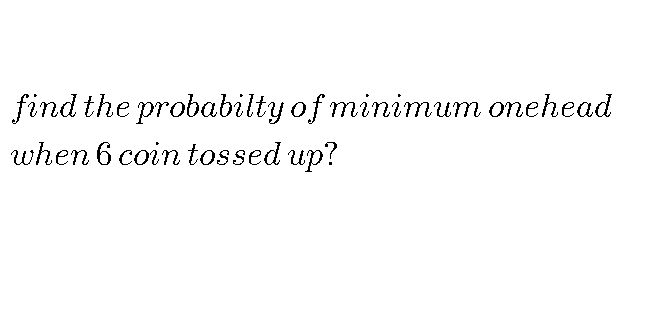
Question Number 74853 Answers: 0 Comments: 2

Is it possible to combine 2cos(90°x)+cos(180°x) into a form of a∙cos(b∙x+c) 2cos((π/2)x)+cos(πx)=^? a∙cos(bx+c)

$$\mathrm{Is}\:\mathrm{it}\:\mathrm{possible}\:\mathrm{to}\:\mathrm{combine}\:\mathrm{2}{cos}\left(\mathrm{90}°{x}\right)+{cos}\left(\mathrm{180}°{x}\right) \\ $$$$\mathrm{into}\:\mathrm{a}\:\mathrm{form}\:\mathrm{of}\:\:{a}\centerdot{cos}\left({b}\centerdot{x}+{c}\right) \\ $$$$\mathrm{2}{cos}\left(\frac{\pi}{\mathrm{2}}{x}\right)+{cos}\left(\pi{x}\right)\overset{?} {=}{a}\centerdot{cos}\left({bx}+{c}\right) \\ $$

Question Number 74840 Answers: 0 Comments: 3

Question Number 74825 Answers: 0 Comments: 5

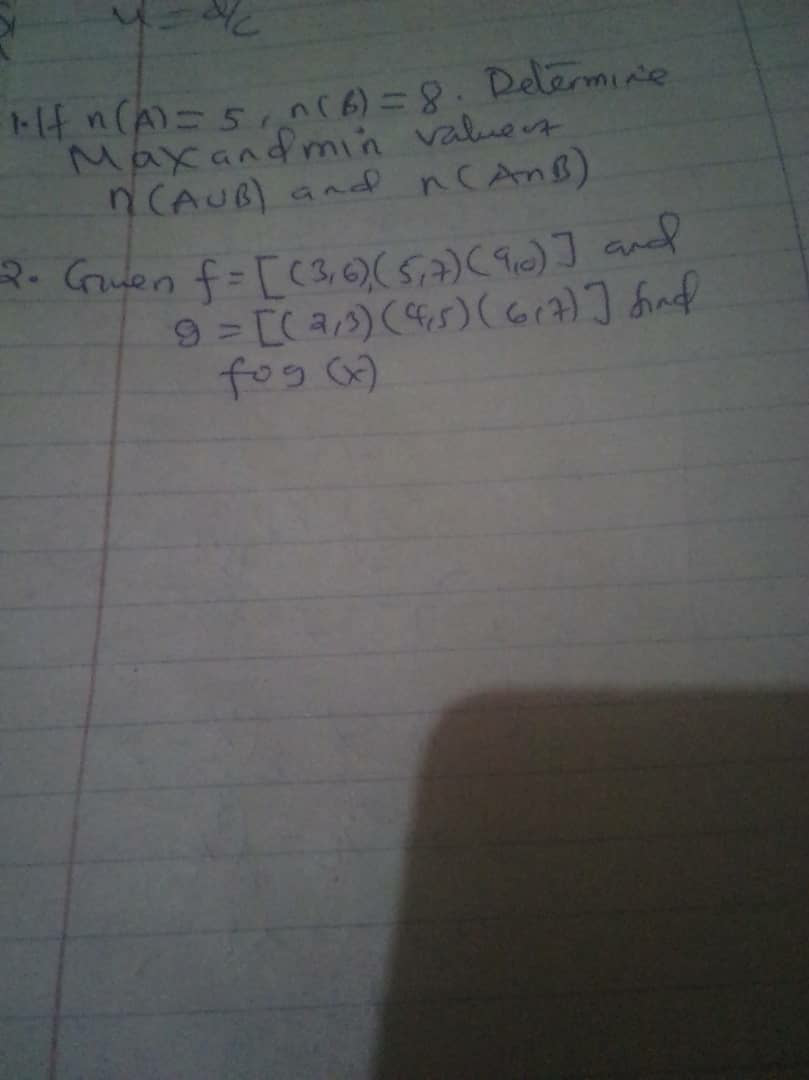
Question Number 74821 Answers: 1 Comments: 0

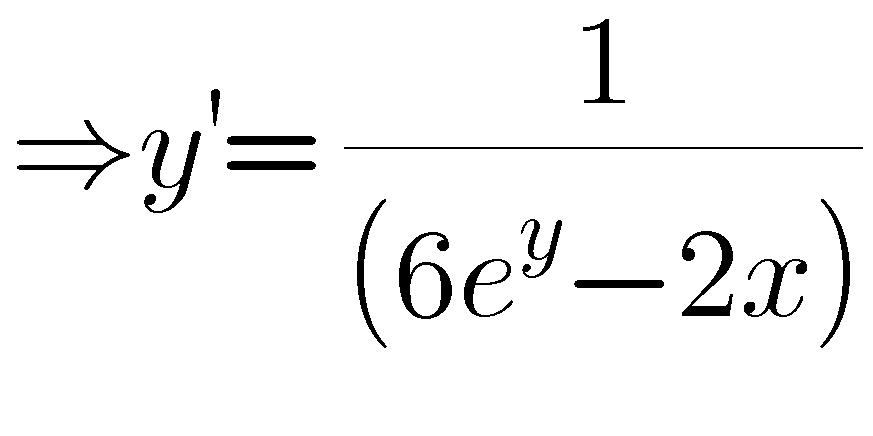
Question Number 74819 Answers: 1 Comments: 2

Expand Σ ((4n−1)/3)+(2/3)Σ_(k=1) ^(n−1) cos(120k)

$$\mathrm{Expand}\:\Sigma \\ $$$$\frac{\mathrm{4}{n}−\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{2}}{\mathrm{3}}\underset{{k}=\mathrm{1}} {\overset{{n}−\mathrm{1}} {\sum}}{cos}\left(\mathrm{120}{k}\right) \\ $$

Question Number 74817 Answers: 1 Comments: 0

Question Number 74802 Answers: 1 Comments: 4

Question Number 74801 Answers: 2 Comments: 2

{ ((x^2 +y^3 =23)),((x^3 +y^2 =32)) :} solve for x and y .

$$\begin{cases}{\boldsymbol{\mathrm{x}}^{\mathrm{2}} +\boldsymbol{\mathrm{y}}^{\mathrm{3}} =\mathrm{23}}\\{\boldsymbol{\mathrm{x}}^{\mathrm{3}} +\boldsymbol{\mathrm{y}}^{\mathrm{2}} =\mathrm{32}}\end{cases}\:\:\:\:\:\:\boldsymbol{\mathrm{solve}}\:\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{x}}\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{y}}\:. \\ $$

Question Number 74800 Answers: 1 Comments: 0

study the existence of f(x)=∫_0 ^∞ ((tcos(tx))/(1+t^2 ))dt

$${study}\:{the}\:{existence}\:{of}\:{f}\left({x}\right)=\int_{\mathrm{0}} ^{\infty} \:\:\frac{{tcos}\left({tx}\right)}{\mathrm{1}+{t}^{\mathrm{2}} }{dt} \\ $$

Pg 1365 Pg 1366 Pg 1367 Pg 1368 Pg 1369 Pg 1370 Pg 1371 Pg 1372 Pg 1373 Pg 1374

Contact: info@tinkutara.com