Question and Answers Forum

AllQuestion and Answers: Page 1369

Question Number 75439 Answers: 1 Comments: 0

A man left his office in a car at 10am to restaurant 30km away. He expected to arrive at 11:10am but had to stop 15km from the office for 15 minutes . He arrived at the restaurant 5 minutes late. a. Find the initial speed b. Draw a travel graph for the whole journey

$$\mathrm{A}\:\mathrm{man}\:\mathrm{left}\:\mathrm{his}\:\mathrm{office}\:\mathrm{in}\:\mathrm{a}\:\mathrm{car}\:\mathrm{at}\:\mathrm{10am} \\ $$$$\mathrm{to}\:\mathrm{restaurant}\:\mathrm{30km}\:\mathrm{away}.\:\mathrm{He}\:\mathrm{expected} \\ $$$$\mathrm{to}\:\mathrm{arrive}\:\mathrm{at}\:\mathrm{11}:\mathrm{10am}\:\mathrm{but}\:\mathrm{had}\:\mathrm{to}\:\mathrm{stop}\: \\ $$$$\mathrm{15km}\:\mathrm{from}\:\mathrm{the}\:\mathrm{office}\:\mathrm{for}\:\mathrm{15}\:\mathrm{minutes}\:. \\ $$$$\mathrm{He}\:\mathrm{arrived}\:\mathrm{at}\:\mathrm{the}\:\mathrm{restaurant}\:\mathrm{5}\:\mathrm{minutes}\: \\ $$$$\mathrm{late}. \\ $$$$\mathrm{a}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{initial}\:\mathrm{speed} \\ $$$$\mathrm{b}.\:\mathrm{Draw}\:\mathrm{a}\:\mathrm{travel}\:\mathrm{graph}\:\mathrm{for}\:\mathrm{the}\:\mathrm{whole}\:\mathrm{journey} \\ $$

Question Number 75437 Answers: 1 Comments: 6

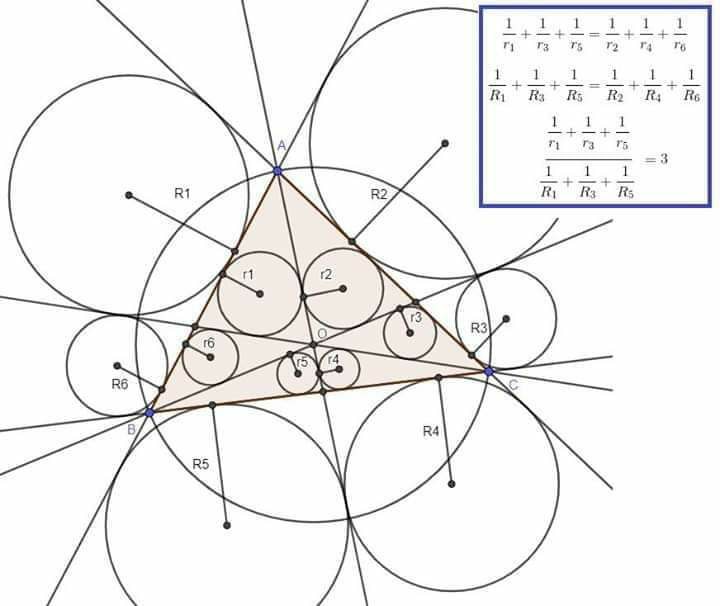
Question Number 75435 Answers: 0 Comments: 4

solve the integral with Residue theorem. ∫_0 ^(2π) ((3 dθ)/(9 +sin^2 θ))

$${solve}\:{the}\:{integral}\:{with}\:{Residue}\:{theorem}. \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{2}\pi} {\int}}\frac{\mathrm{3}\:{d}\theta}{\mathrm{9}\:+\mathrm{sin}^{\mathrm{2}} \theta} \\ $$

Question Number 75426 Answers: 1 Comments: 0

A boy has to cover 4km to catch a bus. He walks part of the distance as 3km/h and run the rest at 5km/h if he takes 1hour to complete the distance. For how many kilometres does he walk

$$\mathrm{A}\:\mathrm{boy}\:\mathrm{has}\:\mathrm{to}\:\mathrm{cover}\:\:\mathrm{4km}\:\:\mathrm{to}\: \\ $$$$\mathrm{catch}\:\mathrm{a}\:\mathrm{bus}.\:\mathrm{He}\:\mathrm{walks}\:\mathrm{part}\:\mathrm{of}\:\mathrm{the}\: \\ $$$$\mathrm{distance}\:\mathrm{as}\:\mathrm{3km}/\mathrm{h}\:\mathrm{and}\:\mathrm{run}\:\mathrm{the}\:\mathrm{rest}\:\mathrm{at} \\ $$$$\mathrm{5km}/\mathrm{h}\:\mathrm{if}\:\mathrm{he}\:\mathrm{takes}\:\mathrm{1hour}\:\mathrm{to}\:\mathrm{complete}\: \\ $$$$\mathrm{the}\:\mathrm{distance}.\:\mathrm{For}\:\mathrm{how}\:\mathrm{many}\:\mathrm{kilometres} \\ $$$$\mathrm{does}\:\mathrm{he}\:\mathrm{walk} \\ $$

Question Number 75421 Answers: 0 Comments: 3

If p is a point in the base AB of a triangle ABC such that AP :PB=P:Q prove that (p+q)cot θ=qcot A−pcot B

$${If}\:{p}\:{is}\:{a}\:{point}\:{in}\:{the}\:{base} \\ $$$${AB}\:{of}\:\:{a}\:\:{triangle}\:\:{ABC} \\ $$$${such}\:{that}\:{AP}\:\::{PB}={P}:{Q} \\ $$$${prove}\:{that} \\ $$$$\left({p}+{q}\right)\mathrm{cot}\:\theta={q}\mathrm{cot}\:{A}−{p}\mathrm{cot}\:{B} \\ $$

Question Number 75403 Answers: 1 Comments: 0

Question Number 75402 Answers: 0 Comments: 4

Explain the proof with appropriate diagram : Lim_(h→0) ((f(x)−f(x−h))/(−h)) = (dy/dx) , where y = f(x)

$$\mathrm{Explain}\:\mathrm{the}\:\mathrm{proof}\: \\ $$$$\mathrm{with}\:\mathrm{appropriate} \\ $$$$\mathrm{diagram}\::\: \\ $$$$\mathrm{Lim}_{{h}\rightarrow\mathrm{0}} \frac{{f}\left({x}\right)−{f}\left({x}−{h}\right)}{−{h}}\: \\ $$$$\:\:\:=\:\frac{{dy}}{{dx}}\:,\:\mathrm{where}\:{y}\:=\:{f}\left({x}\right) \\ $$

Question Number 75392 Answers: 1 Comments: 0

what is the general formular for the (d^n /dx^n )((x/(e^x −1)))

$${what}\:{is}\:{the}\:{general}\:{formular} \\ $$$${for}\:{the}\:\frac{{d}^{{n}} }{{dx}^{{n}} }\left(\frac{{x}}{{e}^{{x}} −\mathrm{1}}\right) \\ $$

Question Number 75391 Answers: 0 Comments: 8

Question Number 75606 Answers: 1 Comments: 1

Question Number 75607 Answers: 0 Comments: 0

Prove that ∫_0 ^∞ 3(((sinx)/x))^4 dx= π

$$\mathrm{Prove}\:\mathrm{that}\:\: \\ $$$$\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{3}\left(\frac{\mathrm{sinx}}{\mathrm{x}}\right)^{\mathrm{4}} \mathrm{dx}=\:\pi \\ $$

Question Number 75386 Answers: 1 Comments: 1

Question Number 75382 Answers: 1 Comments: 1

Question Number 75377 Answers: 0 Comments: 2

Question Number 75376 Answers: 1 Comments: 1

Question Number 75375 Answers: 0 Comments: 1

A rubber tube can be divided into 25 pieces each of length 56cm long. How many pieces each 35cm long can be out from the tube.

$$\mathrm{A}\:\mathrm{rubber}\:\mathrm{tube}\:\mathrm{can}\:\mathrm{be}\:\mathrm{divided}\:\mathrm{into} \\ $$$$\mathrm{25}\:\mathrm{pieces}\:\mathrm{each}\:\mathrm{of}\:\mathrm{length}\:\mathrm{56cm}\:\mathrm{long}.\: \\ $$$$\mathrm{How}\:\mathrm{many}\:\mathrm{pieces}\:\mathrm{each}\:\mathrm{35cm}\:\mathrm{long}\:\mathrm{can}\: \\ $$$$\mathrm{be}\:\mathrm{out}\:\mathrm{from}\:\mathrm{the}\:\mathrm{tube}. \\ $$

Question Number 75368 Answers: 2 Comments: 1

Find the interval for which the function f(x) = sinx + cosx, for x∈ [0, 2π] is strictly inceasing and srictly decreasing ?

$$ \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{interval}\:\mathrm{for}\:\mathrm{which}\:\mathrm{the}\:\mathrm{function} \\ $$$$\:{f}\left({x}\right)\:=\:{sinx}\:+\:{cosx},\:\mathrm{for}\:\:{x}\in\:\left[\mathrm{0},\:\mathrm{2}\pi\right] \\ $$$$\mathrm{is}\:\mathrm{strictly}\:\mathrm{inceasing}\:\mathrm{and}\:\mathrm{srictly}\:\mathrm{decreasing}\:? \\ $$

Question Number 75367 Answers: 1 Comments: 3

(6/(100))×10=?

$$\frac{\mathrm{6}}{\mathrm{100}}×\mathrm{10}=? \\ $$

Question Number 75366 Answers: 1 Comments: 0

i need the sol plz expansion the maclaurin series f(z)=(z/(z^4 + 9)) = (z/9) ×(1/(1+(z^4 /9)))

$${i}\:{need}\:{the}\:{sol}\:{plz} \\ $$$${expansion}\:{the}\:{maclaurin}\:{series} \\ $$$${f}\left({z}\right)=\frac{{z}}{{z}^{\mathrm{4}} \:+\:\mathrm{9}}\:=\:\frac{{z}}{\mathrm{9}}\:×\frac{\mathrm{1}}{\mathrm{1}+\frac{{z}^{\mathrm{4}} }{\mathrm{9}}}\: \\ $$

Question Number 75360 Answers: 0 Comments: 0

Question Number 75358 Answers: 0 Comments: 0

Question Number 75351 Answers: 1 Comments: 0

Question Number 75339 Answers: 0 Comments: 2

A water flows from a tap into an empty cylindrical jar at rate of 23211cm^3 per secons. At what time will the tap fill the cylinder with volume of 6011cm^3 .

$$\mathrm{A}\:\mathrm{water}\:\mathrm{flows}\:\mathrm{from}\:\mathrm{a}\:\mathrm{tap}\:\mathrm{into}\:\mathrm{an}\:\mathrm{empty} \\ $$$$\mathrm{cylindrical}\:\mathrm{jar}\:\mathrm{at}\:\mathrm{rate}\:\mathrm{of}\:\:\mathrm{23211cm}^{\mathrm{3}} \:\mathrm{per} \\ $$$$\mathrm{secons}.\:\mathrm{At}\:\mathrm{what}\:\mathrm{time}\:\mathrm{will}\:\mathrm{the}\:\mathrm{tap}\:\mathrm{fill}\: \\ $$$$\mathrm{the}\:\mathrm{cylinder}\:\mathrm{with}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{6011cm}^{\mathrm{3}} . \\ $$

Question Number 75330 Answers: 0 Comments: 3

Question Number 75329 Answers: 1 Comments: 0

Question Number 75327 Answers: 1 Comments: 3

If T_(n + 1) = 1 + (1/2)T_n Find a formular for T_n in terms of n and find the sum of first n terms

$$\mathrm{If}\:\:\:\:\:\mathrm{T}_{\mathrm{n}\:+\:\mathrm{1}} \:\:\:=\:\:\:\mathrm{1}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{T}_{\mathrm{n}} \\ $$$$\mathrm{Find}\:\mathrm{a}\:\mathrm{formular}\:\mathrm{for}\:\:\mathrm{T}_{\mathrm{n}} \:\:\mathrm{in}\:\mathrm{terms}\:\mathrm{of}\:\:\mathrm{n} \\ $$$$\mathrm{and}\:\mathrm{find}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{first}\:\mathrm{n}\:\mathrm{terms} \\ $$

Pg 1364 Pg 1365 Pg 1366 Pg 1367 Pg 1368 Pg 1369 Pg 1370 Pg 1371 Pg 1372 Pg 1373

Contact: info@tinkutara.com