Question and Answers Forum

AllQuestion and Answers: Page 1352

Question Number 75607 Answers: 0 Comments: 0

Prove that ∫_0 ^∞ 3(((sinx)/x))^4 dx= π

$$\mathrm{Prove}\:\mathrm{that}\:\: \\ $$$$\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{3}\left(\frac{\mathrm{sinx}}{\mathrm{x}}\right)^{\mathrm{4}} \mathrm{dx}=\:\pi \\ $$

Question Number 75386 Answers: 1 Comments: 1

Question Number 75382 Answers: 1 Comments: 1

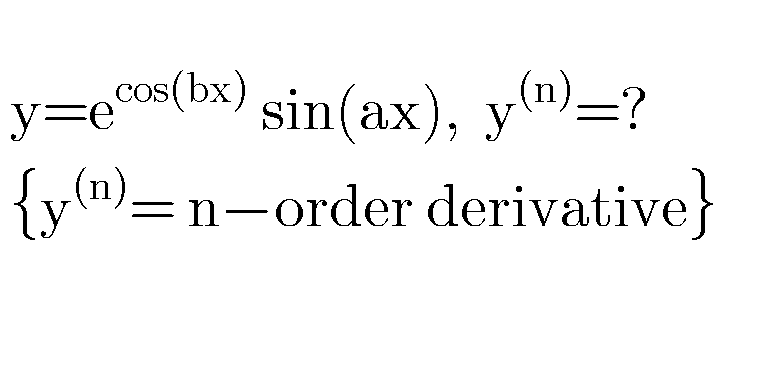
Question Number 75377 Answers: 0 Comments: 2

Question Number 75376 Answers: 1 Comments: 1

Question Number 75375 Answers: 0 Comments: 1

A rubber tube can be divided into 25 pieces each of length 56cm long. How many pieces each 35cm long can be out from the tube.

$$\mathrm{A}\:\mathrm{rubber}\:\mathrm{tube}\:\mathrm{can}\:\mathrm{be}\:\mathrm{divided}\:\mathrm{into} \\ $$$$\mathrm{25}\:\mathrm{pieces}\:\mathrm{each}\:\mathrm{of}\:\mathrm{length}\:\mathrm{56cm}\:\mathrm{long}.\: \\ $$$$\mathrm{How}\:\mathrm{many}\:\mathrm{pieces}\:\mathrm{each}\:\mathrm{35cm}\:\mathrm{long}\:\mathrm{can}\: \\ $$$$\mathrm{be}\:\mathrm{out}\:\mathrm{from}\:\mathrm{the}\:\mathrm{tube}. \\ $$

Question Number 75368 Answers: 2 Comments: 1

Find the interval for which the function f(x) = sinx + cosx, for x∈ [0, 2π] is strictly inceasing and srictly decreasing ?

$$ \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{interval}\:\mathrm{for}\:\mathrm{which}\:\mathrm{the}\:\mathrm{function} \\ $$$$\:{f}\left({x}\right)\:=\:{sinx}\:+\:{cosx},\:\mathrm{for}\:\:{x}\in\:\left[\mathrm{0},\:\mathrm{2}\pi\right] \\ $$$$\mathrm{is}\:\mathrm{strictly}\:\mathrm{inceasing}\:\mathrm{and}\:\mathrm{srictly}\:\mathrm{decreasing}\:? \\ $$

Question Number 75367 Answers: 1 Comments: 3

(6/(100))×10=?

$$\frac{\mathrm{6}}{\mathrm{100}}×\mathrm{10}=? \\ $$

Question Number 75366 Answers: 1 Comments: 0

i need the sol plz expansion the maclaurin series f(z)=(z/(z^4 + 9)) = (z/9) ×(1/(1+(z^4 /9)))

$${i}\:{need}\:{the}\:{sol}\:{plz} \\ $$$${expansion}\:{the}\:{maclaurin}\:{series} \\ $$$${f}\left({z}\right)=\frac{{z}}{{z}^{\mathrm{4}} \:+\:\mathrm{9}}\:=\:\frac{{z}}{\mathrm{9}}\:×\frac{\mathrm{1}}{\mathrm{1}+\frac{{z}^{\mathrm{4}} }{\mathrm{9}}}\: \\ $$

Question Number 75360 Answers: 0 Comments: 0

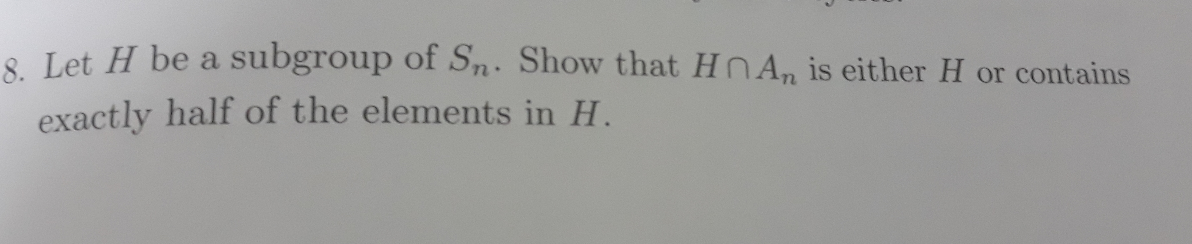
Question Number 75358 Answers: 0 Comments: 0

Question Number 75351 Answers: 1 Comments: 0

Question Number 75339 Answers: 0 Comments: 2

A water flows from a tap into an empty cylindrical jar at rate of 23211cm^3 per secons. At what time will the tap fill the cylinder with volume of 6011cm^3 .

$$\mathrm{A}\:\mathrm{water}\:\mathrm{flows}\:\mathrm{from}\:\mathrm{a}\:\mathrm{tap}\:\mathrm{into}\:\mathrm{an}\:\mathrm{empty} \\ $$$$\mathrm{cylindrical}\:\mathrm{jar}\:\mathrm{at}\:\mathrm{rate}\:\mathrm{of}\:\:\mathrm{23211cm}^{\mathrm{3}} \:\mathrm{per} \\ $$$$\mathrm{secons}.\:\mathrm{At}\:\mathrm{what}\:\mathrm{time}\:\mathrm{will}\:\mathrm{the}\:\mathrm{tap}\:\mathrm{fill}\: \\ $$$$\mathrm{the}\:\mathrm{cylinder}\:\mathrm{with}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{6011cm}^{\mathrm{3}} . \\ $$

Question Number 75330 Answers: 0 Comments: 3

Question Number 75329 Answers: 1 Comments: 0

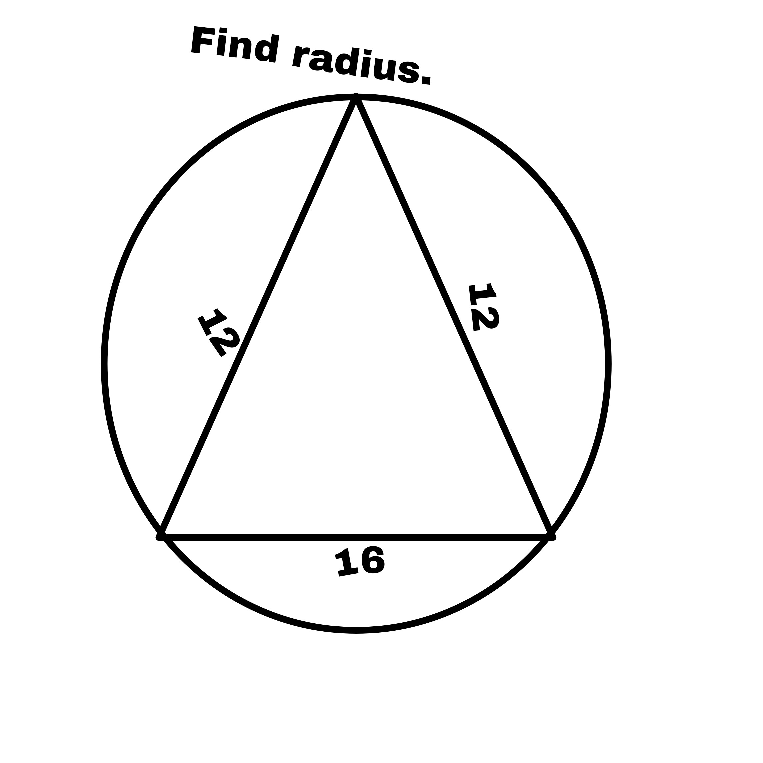
Question Number 75327 Answers: 1 Comments: 3

If T_(n + 1) = 1 + (1/2)T_n Find a formular for T_n in terms of n and find the sum of first n terms

$$\mathrm{If}\:\:\:\:\:\mathrm{T}_{\mathrm{n}\:+\:\mathrm{1}} \:\:\:=\:\:\:\mathrm{1}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{T}_{\mathrm{n}} \\ $$$$\mathrm{Find}\:\mathrm{a}\:\mathrm{formular}\:\mathrm{for}\:\:\mathrm{T}_{\mathrm{n}} \:\:\mathrm{in}\:\mathrm{terms}\:\mathrm{of}\:\:\mathrm{n} \\ $$$$\mathrm{and}\:\mathrm{find}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{first}\:\mathrm{n}\:\mathrm{terms} \\ $$

Question Number 75325 Answers: 1 Comments: 0

Question Number 77131 Answers: 1 Comments: 0

Question Number 75338 Answers: 0 Comments: 3

The wall of mr nelsons bathroom is covered with 1200 square tiles eah of length 24cm. How many square tiles of sides length 24cm would be needed to cover the same wall.

$$\mathrm{The}\:\mathrm{wall}\:\mathrm{of}\:\mathrm{mr}\:\mathrm{nelsons}\:\mathrm{bathroom}\:\mathrm{is} \\ $$$$\mathrm{covered}\:\mathrm{with}\:\mathrm{1200}\:\mathrm{square}\:\mathrm{tiles}\:\mathrm{eah}\:\mathrm{of}\: \\ $$$$\mathrm{length}\:\mathrm{24cm}.\:\mathrm{How}\:\mathrm{many}\:\mathrm{square}\:\mathrm{tiles}\: \\ $$$$\mathrm{of}\:\mathrm{sides}\:\mathrm{length}\:\mathrm{24cm}\:\mathrm{would}\:\mathrm{be}\:\mathrm{needed}\:\mathrm{to}\: \\ $$$$\mathrm{cover}\:\mathrm{the}\:\mathrm{same}\:\mathrm{wall}. \\ $$

Question Number 75321 Answers: 0 Comments: 0

lim_(x→∞) ((𝚷_(k=0) ^n ((n),(k) )))^(1/(n(n+1)))

$$ \\ $$$$\: \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{l}\boldsymbol{\mathrm{im}}}\:\sqrt[{\boldsymbol{{n}}\left(\boldsymbol{{n}}+\mathrm{1}\right)}]{\underset{\boldsymbol{{k}}=\mathrm{0}} {\overset{\boldsymbol{{n}}} {\boldsymbol{\prod}}}\begin{pmatrix}{\boldsymbol{{n}}}\\{\boldsymbol{{k}}}\end{pmatrix}} \\ $$

Question Number 75319 Answers: 0 Comments: 0

Question Number 75318 Answers: 0 Comments: 6

Question Number 75317 Answers: 2 Comments: 1

Find the image of the line ((x−2)/(−1)) = ((y−3)/2) = ((z−4)/3) in the plane 2x+3y−4z +3=0.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{image}\:\mathrm{of}\:\mathrm{the}\:\mathrm{line}\: \\ $$$$\frac{{x}−\mathrm{2}}{−\mathrm{1}}\:=\:\frac{\mathrm{y}−\mathrm{3}}{\mathrm{2}}\:=\:\frac{\mathrm{z}−\mathrm{4}}{\mathrm{3}}\:\:\:\mathrm{in}\:\mathrm{the}\:\mathrm{plane}\: \\ $$$$\mathrm{2}{x}+\mathrm{3}{y}−\mathrm{4}{z}\:+\mathrm{3}=\mathrm{0}. \\ $$

Question Number 75306 Answers: 0 Comments: 3

Use Venn diagram to represent the set of natural numbers, integers, rational numbers, irrational numbers, real numbers, complex numbers

$${Use}\:{Venn}\:{diagram}\:{to} \\ $$$${represent}\:{the}\:{set}\:{of}\:{natural} \\ $$$${numbers},\:{integers},\:{rational} \\ $$$${numbers},\:{irrational} \\ $$$${numbers},\:{real}\:{numbers}, \\ $$$${complex}\:{numbers} \\ $$

Question Number 75303 Answers: 1 Comments: 0

If cosθ = ((cosα−cosβ)/(1−cosα cosβ)) , prove that one of the value of tan(θ/2) is tan(α/2) . tan(β/2) ??

$$\mathrm{If}\:{cos}\theta\:=\:\frac{{cos}\alpha−\mathrm{cos}\beta}{\mathrm{1}−{cos}\alpha\:{cos}\beta}\:,\:\mathrm{prove}\:\mathrm{that}\:\mathrm{one} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{tan}\frac{\theta}{\mathrm{2}}\:\mathrm{is}\:{tan}\frac{\alpha}{\mathrm{2}}\:.\:{tan}\frac{\beta}{\mathrm{2}}\:?? \\ $$

Question Number 75302 Answers: 1 Comments: 1

If θ is eleminated from the equation x = a cos(θ−α) and y = b cos(θ−β) then prove that (x^2 /a^2 ) + (y^2 /b^2 ) − ((2xy)/(ab)) cos(α−β) = sin^2 (α−β) ?

$$\mathrm{If}\:\theta\:\mathrm{is}\:\mathrm{eleminated}\:\mathrm{from}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{x}\:=\: \\ $$$${a}\:{cos}\left(\theta−\alpha\right)\:\mathrm{and}\:{y}\:=\:{b}\:{cos}\left(\theta−\beta\right)\:\mathrm{then} \\ $$$$\mathrm{prove}\:\mathrm{that}\:\frac{{x}^{\mathrm{2}} }{{a}^{\mathrm{2}} }\:+\:\frac{{y}^{\mathrm{2}} }{{b}^{\mathrm{2}} }\:−\:\frac{\mathrm{2}{xy}}{{ab}}\:{cos}\left(\alpha−\beta\right)\:=\:{sin}^{\mathrm{2}} \left(\alpha−\beta\right)\:? \\ $$

Pg 1347 Pg 1348 Pg 1349 Pg 1350 Pg 1351 Pg 1352 Pg 1353 Pg 1354 Pg 1355 Pg 1356

Contact: info@tinkutara.com