
AllQuestion and Answers: Page 1349
Question Number 77158 Answers: 0 Comments: 4
Question Number 77154 Answers: 0 Comments: 6

Question Number 77149 Answers: 0 Comments: 2
Question Number 77147 Answers: 0 Comments: 1
Question Number 77144 Answers: 1 Comments: 2

Question Number 77143 Answers: 1 Comments: 2

Question Number 77132 Answers: 0 Comments: 1

Question Number 77129 Answers: 1 Comments: 0
Question Number 77128 Answers: 2 Comments: 0
Question Number 77127 Answers: 2 Comments: 0
Question Number 77126 Answers: 1 Comments: 0
Question Number 77123 Answers: 1 Comments: 0
Question Number 77119 Answers: 2 Comments: 0
Question Number 77117 Answers: 0 Comments: 0
Question Number 77103 Answers: 1 Comments: 1
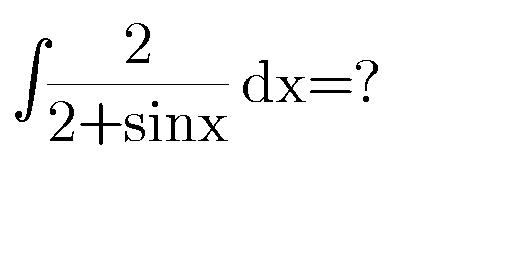
Question Number 77091 Answers: 0 Comments: 3

Question Number 77089 Answers: 0 Comments: 2
Question Number 77087 Answers: 1 Comments: 1
Question Number 77086 Answers: 1 Comments: 2
$$\int_{\mathrm{0}} ^{\mathrm{1}} {xtan}^{−\mathrm{1}} {xdx} \\ $$$$ \\ $$
Question Number 77067 Answers: 1 Comments: 2

Question Number 77066 Answers: 0 Comments: 5

Question Number 77057 Answers: 1 Comments: 2
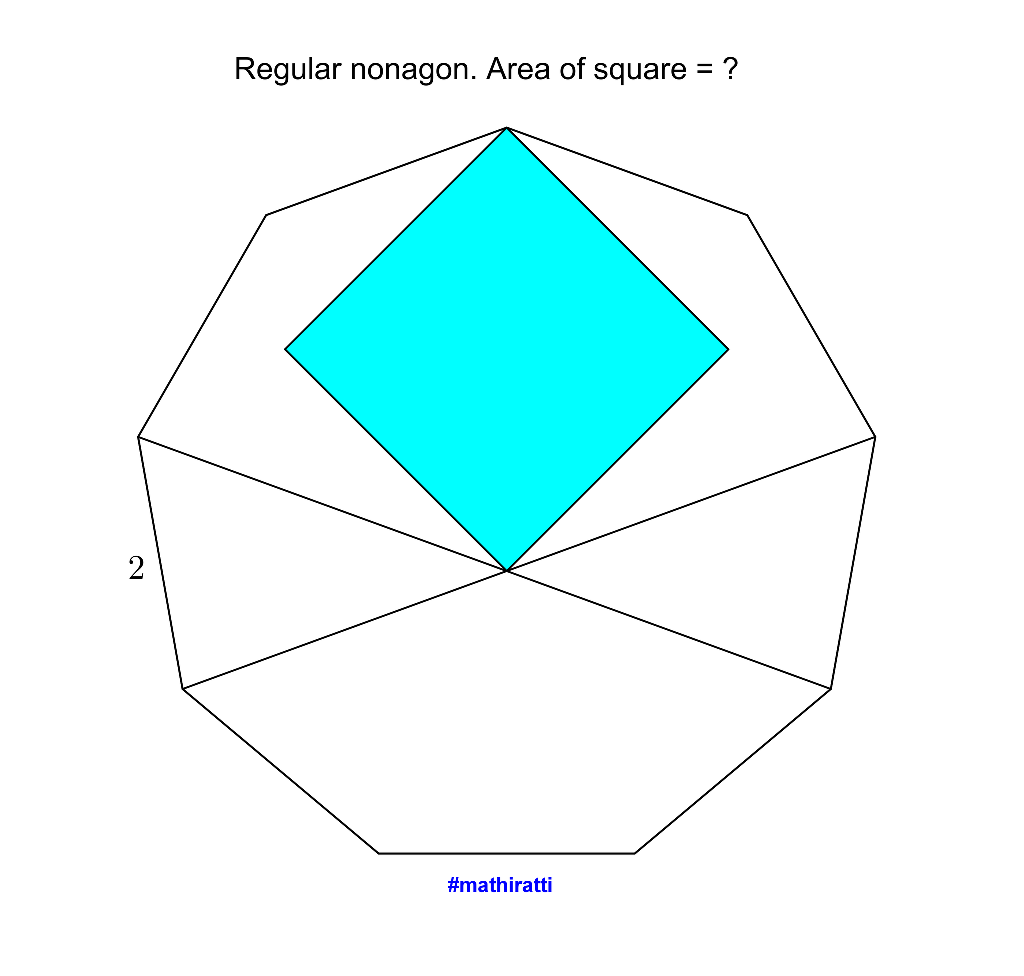
Question Number 77046 Answers: 1 Comments: 0
$${x}={R}^{\mathrm{2}} \sqrt{\mathrm{1}−\frac{{t}^{\mathrm{2}} }{{R}^{\mathrm{2}} }}\: \\ $$
Question Number 77041 Answers: 2 Comments: 0
Question Number 77036 Answers: 0 Comments: 12

Question Number 77035 Answers: 1 Comments: 0
Pg 1344 Pg 1345 Pg 1346 Pg 1347 Pg 1348 Pg 1349 Pg 1350 Pg 1351 Pg 1352 Pg 1353
