Question and Answers Forum

AllQuestion and Answers: Page 1326

Question Number 79565 Answers: 0 Comments: 2

lim_(x→∞) (x−(√(x^2 −x+1)) )×(((ln(e^x +x))/x))

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(\mathrm{x}−\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}\:\right)×\left(\frac{\mathrm{ln}\left(\mathrm{e}^{\mathrm{x}} +\mathrm{x}\right)}{\mathrm{x}}\right) \\ $$

Question Number 79560 Answers: 0 Comments: 1

(√(1+x)) ≤ ((5−x))^(1/(4 ))

$$\sqrt{\mathrm{1}+\mathrm{x}}\:\leqslant\:\sqrt[{\mathrm{4}\:}]{\mathrm{5}−\mathrm{x}} \\ $$

Question Number 79538 Answers: 1 Comments: 13

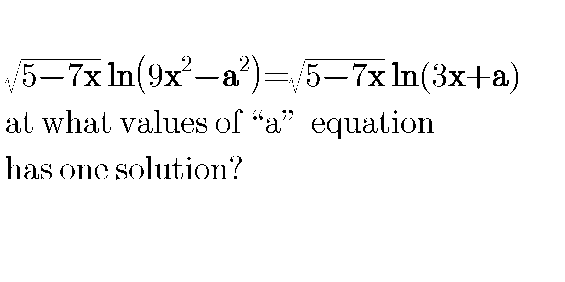
Question Number 79536 Answers: 0 Comments: 4

Question Number 79532 Answers: 1 Comments: 2

Given function f : R ⇒ R x^2 f(x) + f(1 − x) = 2x − x^4 f(2019) = ?

$${Given}\:\:{function}\:\:{f}\::\:\mathbb{R}\:\:\Rightarrow\:\:\mathbb{R} \\ $$$$\:\:\:\:\:\:\:\:{x}^{\mathrm{2}} \:{f}\left({x}\right)\:+\:{f}\left(\mathrm{1}\:−\:{x}\right)\:\:=\:\:\mathrm{2}{x}\:−\:{x}^{\mathrm{4}} \\ $$$${f}\left(\mathrm{2019}\right)\:\:=\:\:? \\ $$

Question Number 79531 Answers: 0 Comments: 1

∫^1 _0 ((ln((1/x)+x))/(x^2 +1))dx ?

$$\underset{\mathrm{0}} {\int}^{\mathrm{1}} \:\frac{\mathrm{ln}\left(\frac{\mathrm{1}}{\mathrm{x}}+\mathrm{x}\right)}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\mathrm{dx}\:? \\ $$

Question Number 79528 Answers: 0 Comments: 0

find ∫_0 ^∞ e^(−x^3 ) cos(x^2 )dx

$${find}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{x}^{\mathrm{3}} } {cos}\left({x}^{\mathrm{2}} \right){dx} \\ $$

Question Number 79527 Answers: 0 Comments: 1

find ∫_0 ^∞ e^(−x^3 ) dx

$${find}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{x}^{\mathrm{3}} } {dx} \\ $$

Question Number 79520 Answers: 1 Comments: 2

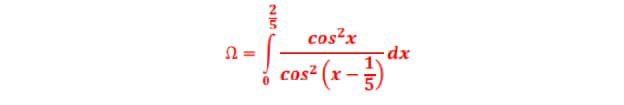
Question Number 79516 Answers: 0 Comments: 3

Question Number 79515 Answers: 0 Comments: 0

Question Number 79513 Answers: 0 Comments: 1

Q.solve if t^2 =n^2 cos^2 (x)+m^2 sin^2 (x) then show that: t+(d^2 t/dx^2 )=(((nm)^2 )/t^3 )

$${Q}.{solve} \\ $$$${if}\:{t}^{\mathrm{2}} ={n}^{\mathrm{2}} {cos}^{\mathrm{2}} \left({x}\right)+{m}^{\mathrm{2}} {sin}^{\mathrm{2}} \left({x}\right) \\ $$$$ \\ $$$${then}\:{show}\:{that}: \\ $$$${t}+\frac{{d}^{\mathrm{2}} {t}}{{dx}^{\mathrm{2}} }=\frac{\left({nm}\right)^{\mathrm{2}} }{{t}^{\mathrm{3}} } \\ $$$$ \\ $$

Question Number 79512 Answers: 0 Comments: 0

Q.solve if t^2 =n^2 cos^2 (x)+m^2 sin^2 (x) then show that: t+(d^2 t/dx^2 )=(((nm)^2 )/t^3 )

Question Number 79852 Answers: 2 Comments: 1

Question Number 79500 Answers: 1 Comments: 0

∫(cot^2 x+cot^4 x)dx

$$\int\left(\mathrm{cot}\:^{\mathrm{2}} {x}+\mathrm{cot}\:^{\mathrm{4}} {x}\right){dx} \\ $$

Question Number 79499 Answers: 0 Comments: 2

∫_0 ^(30π) ∣sin x∣ dx=

$$\underset{\mathrm{0}} {\overset{\mathrm{30}\pi} {\int}}\mid\mathrm{sin}\:\mathrm{x}\mid\:\mathrm{dx}=\: \\ $$

Question Number 79491 Answers: 0 Comments: 4

Find the number of used place

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{used}\:\mathrm{place} \\ $$

Question Number 79485 Answers: 1 Comments: 0

∫(tan^2 x+tan^4 x)dx

$$\int\left(\mathrm{tan}\:^{\mathrm{2}} {x}+\mathrm{tan}\:^{\mathrm{4}} {x}\right){dx} \\ $$

Question Number 79480 Answers: 0 Comments: 3

Question Number 79479 Answers: 0 Comments: 1

how do 16 people play 3 matches in teams of 4 but must only be in the same team once ?

$$\mathrm{how}\:\mathrm{do}\:\mathrm{16}\:\mathrm{people}\:\mathrm{play}\:\mathrm{3}\:\mathrm{matches} \\ $$$$\mathrm{in}\:\mathrm{teams}\:\mathrm{of}\:\mathrm{4}\:\mathrm{but}\:\mathrm{must}\:\mathrm{only}\:\mathrm{be} \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{same}\:\mathrm{team}\:\mathrm{once}\:?\: \\ $$

Question Number 79497 Answers: 1 Comments: 0

(((4x−∣x−6∣)(log_(1/3) (x+4)+1))/2^(x^2 −2^(∣x∣) ) )≥0

$$\frac{\left(\mathrm{4}{x}−\mid{x}−\mathrm{6}\mid\right)\left(\mathrm{log}_{\frac{\mathrm{1}}{\mathrm{3}}} \left({x}+\mathrm{4}\right)+\mathrm{1}\right)}{\mathrm{2}^{{x}^{\mathrm{2}} −\mathrm{2}^{\mid{x}\mid} } }\geqslant\mathrm{0} \\ $$

Question Number 79472 Answers: 0 Comments: 5

what the minimum value of f(x)=(√(x^2 +2x+5)) +(√(x^2 −14x+65))

$$\mathrm{what}\:\mathrm{the}\:\mathrm{minimum}\:\mathrm{value}\:\mathrm{of} \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{2x}+\mathrm{5}}\:+\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{14x}+\mathrm{65}} \\ $$

Question Number 79469 Answers: 0 Comments: 0

Q.solve −(d^2 y/dt^2 )−coth(t)(dy/dt)+(20+(4/(sinh^2 (t))))y=0

$${Q}.{solve} \\ $$$$−\frac{{d}^{\mathrm{2}} {y}}{{dt}^{\mathrm{2}} }−{coth}\left({t}\right)\frac{{dy}}{{dt}}+\left(\mathrm{20}+\frac{\mathrm{4}}{{sinh}^{\mathrm{2}} \left({t}\right)}\right){y}=\mathrm{0} \\ $$

Question Number 79462 Answers: 1 Comments: 0

prove p⇒q and ∼q⇒∼p are logicaly equivalent with out truth table

$${prove}\:{p}\Rightarrow{q}\:{and}\:\sim{q}\Rightarrow\sim{p}\:{are}\:{logicaly}\: \\ $$$${equivalent}\:{with}\:{out}\:{truth}\:{table} \\ $$$$ \\ $$

Question Number 79456 Answers: 0 Comments: 2

If A and B are acute positive angles satisfying the equations 3 sin^2 A+2 sin^2 B=1 and 3 sin 2A−2 sin 2B=0, then A+2B=

$$\mathrm{If}\:{A}\:\mathrm{and}\:{B}\:\mathrm{are}\:\mathrm{acute}\:\mathrm{positive}\:\mathrm{angles} \\ $$$$\mathrm{satisfying}\:\mathrm{the}\:\mathrm{equations}\: \\ $$$$\mathrm{3}\:\mathrm{sin}^{\mathrm{2}} {A}+\mathrm{2}\:\mathrm{sin}^{\mathrm{2}} {B}=\mathrm{1}\:\mathrm{and}\: \\ $$$$\mathrm{3}\:\mathrm{sin}\:\mathrm{2}{A}−\mathrm{2}\:\mathrm{sin}\:\mathrm{2}{B}=\mathrm{0},\:\mathrm{then}\:{A}+\mathrm{2}{B}= \\ $$

Question Number 79455 Answers: 1 Comments: 0

If sin θ_1 +sin θ_2 +sin θ_3 = 3, then cos θ_1 +cos θ_2 +cos θ_3 =

$$\mathrm{If}\:\:\mathrm{sin}\:\theta_{\mathrm{1}} +\mathrm{sin}\:\theta_{\mathrm{2}} +\mathrm{sin}\:\theta_{\mathrm{3}} \:=\:\mathrm{3},\:\mathrm{then} \\ $$$$\mathrm{cos}\:\theta_{\mathrm{1}} +\mathrm{cos}\:\theta_{\mathrm{2}} +\mathrm{cos}\:\theta_{\mathrm{3}} \:= \\ $$

Pg 1321 Pg 1322 Pg 1323 Pg 1324 Pg 1325 Pg 1326 Pg 1327 Pg 1328 Pg 1329 Pg 1330

Contact: info@tinkutara.com