Question and Answers Forum

AllQuestion and Answers: Page 1325

Question Number 80237 Answers: 1 Comments: 5

Question Number 80230 Answers: 1 Comments: 0

Question Number 80227 Answers: 0 Comments: 5

how to prove ∫_0 ^1 x^n (1−x)^(m ) dx = ((m! ×n!)/((m+n)!)) via Gamma function

$${how}\:{to}\:{prove} \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:{x}^{{n}} \:\left(\mathrm{1}−{x}\right)^{{m}\:} \:{dx}\:=\:\frac{{m}!\:×{n}!}{\left({m}+{n}\right)!} \\ $$$${via}\:{Gamma}\:{function} \\ $$

Question Number 80222 Answers: 1 Comments: 2

Question Number 80220 Answers: 0 Comments: 3

Question Number 80219 Answers: 1 Comments: 0

Question Number 80209 Answers: 2 Comments: 1

Question Number 80208 Answers: 0 Comments: 0

Question Number 80207 Answers: 0 Comments: 6

Question Number 80206 Answers: 1 Comments: 2

Question Number 80204 Answers: 0 Comments: 2

Question Number 80199 Answers: 2 Comments: 1

Question Number 80180 Answers: 1 Comments: 0

h(x)=((x−x^2 )/(x+1)) we defined this function on R−{−1}→R 1) Study the variations of h then draw up its table of variation. please sirs i need your kind help

$$\mathrm{h}\left({x}\right)=\frac{{x}−{x}^{\mathrm{2}} }{{x}+\mathrm{1}} \\ $$$${we}\:{defined}\:{this}\:{function}\:{on} \\ $$$$\mathbb{R}−\left\{−\mathrm{1}\right\}\rightarrow\mathbb{R} \\ $$$$ \\ $$$$\left.\mathrm{1}\right)\:\mathrm{Study}\:\mathrm{the}\:\mathrm{variations}\:\mathrm{of}\:\mathrm{h}\:\mathrm{then} \\ $$$$\mathrm{draw}\:\mathrm{up}\:\mathrm{its}\:\mathrm{table}\:\mathrm{of}\:\mathrm{variation}. \\ $$$$ \\ $$$$\mathrm{please}\:\mathrm{sirs}\:\mathrm{i}\:\mathrm{need}\:\mathrm{your}\:\mathrm{kind}\:\mathrm{help} \\ $$

Question Number 80178 Answers: 0 Comments: 4

Question Number 80175 Answers: 1 Comments: 1

∫_0 ^1 (dx/(√(x^2 +x+1))) = ?

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{dx}}{\sqrt{{x}^{\mathrm{2}} +{x}+\mathrm{1}}}\:=\:? \\ $$

Question Number 80172 Answers: 0 Comments: 2

Given that lim_(x→0) ((√(f(x)+ x))/h) = L then lim_(x→0) ((√(f(x) + 2x))/h) = ?

$${Given}\:{that}\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{{f}\left({x}\right)+\:{x}}}{{h}}\:=\:{L}\:{then} \\ $$$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\sqrt{{f}\left({x}\right)\:+\:\mathrm{2}{x}}}{{h}}\:=\:? \\ $$

Question Number 80164 Answers: 0 Comments: 0

Question Number 80161 Answers: 0 Comments: 10

Find the relation between q and r so that x^3 +3px^2 +qx+r is a perfect cube for all value of x

$${Find}\:{the}\:{relation}\:{between} \\ $$$${q}\:{and}\:{r}\:\:{so}\:\:{that} \\ $$$${x}^{\mathrm{3}} +\mathrm{3}{px}^{\mathrm{2}} +{qx}+{r}\:{is}\:{a}\:{perfect} \\ $$$${cube}\:{for}\:{all}\:\:{value}\:{of}\:{x} \\ $$

Question Number 80160 Answers: 0 Comments: 1

∫_0 ^∞ (x^3 /(e^(2x) −e^x ))

$$\int_{\mathrm{0}} ^{\infty} \frac{{x}^{\mathrm{3}} }{{e}^{\mathrm{2}{x}} −{e}^{{x}} } \\ $$

Question Number 80159 Answers: 1 Comments: 4

IF THE SUM OF p TERMS OF AN A.P. IS EQUAL TO SUM OF ITS q TERMS. PROVE THAT THE SUM OF (p+q) TERMS OF IT IS EQUAL TO 0(ZERO).

$$\boldsymbol{{IF}}\:\:\boldsymbol{{THE}}\:\:\:\boldsymbol{{SUM}}\:\:\:\boldsymbol{{OF}}\:\:\:\boldsymbol{{p}}\:\:\boldsymbol{{TERMS}} \\ $$$$\boldsymbol{{OF}}\:\:\boldsymbol{{AN}}\:\:\:\:\boldsymbol{{A}}.\boldsymbol{{P}}.\:\:\:\boldsymbol{{IS}}\:\:\:\boldsymbol{{EQUAL}}\:\:\boldsymbol{{TO}} \\ $$$$\boldsymbol{{SUM}}\:\:\boldsymbol{{OF}}\:\:\:\boldsymbol{{ITS}}\:\:\:\boldsymbol{{q}}\:\:\:\boldsymbol{{TERMS}}.\:\: \\ $$$$\boldsymbol{{PROVE}}\:\:\boldsymbol{{THAT}}\:\:\boldsymbol{{THE}}\:\:\boldsymbol{{SUM}}\:\:\boldsymbol{{OF}} \\ $$$$\left(\boldsymbol{{p}}+\boldsymbol{{q}}\right)\:\:\boldsymbol{{TERMS}}\:\:\boldsymbol{{OF}}\:\:\:\boldsymbol{{IT}}\:\:\:\boldsymbol{{IS}}\:\:\: \\ $$$$\boldsymbol{{EQUAL}}\:\:\boldsymbol{{TO}}\:\:\mathrm{0}\left(\boldsymbol{{ZERO}}\right). \\ $$

Question Number 80146 Answers: 1 Comments: 0

Two system of rectangular axes have the same origin. If a plane cuts them at distance a, b, c and p, q, r respectively, then prove with the help of an appropriate diagram that : (1/a^2 ) + (1/b^2 ) + (1/c^2 ) = (1/p^2 ) + (1/q^2 ) + (1/r^2 )

$${Two}\:{system}\:{of}\:{rectangular}\:{axes}\:{have} \\ $$$${the}\:{same}\:{origin}.\:{If}\:{a}\:{plane}\:{cuts}\:{them} \\ $$$${at}\:{distance}\:{a},\:{b},\:{c}\:{and}\:{p},\:{q},\:{r} \\ $$$${respectively},\:{then}\:{prove}\:{with}\:{the}\:{help} \\ $$$${of}\:{an}\:{appropriate}\:{diagram}\:{that}\:: \\ $$$$\frac{\mathrm{1}}{{a}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{b}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\:=\:\frac{\mathrm{1}}{{p}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{q}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{r}^{\mathrm{2}} } \\ $$

Question Number 80139 Answers: 1 Comments: 6

Question Number 80142 Answers: 2 Comments: 0

a. Σ_(k=1) ^∞ ((k^3 /2^k ))=? b. Σ_(k=1) ^∞ (((k^3 +k^2 +k+1)/7^k ))=?

$$\mathrm{a}.\:\:\:\underset{\boldsymbol{\mathrm{k}}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\left(\frac{\boldsymbol{\mathrm{k}}^{\mathrm{3}} }{\mathrm{2}^{\boldsymbol{\mathrm{k}}} }\right)=? \\ $$$$\boldsymbol{\mathrm{b}}.\:\:\:\:\underset{\boldsymbol{\mathrm{k}}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\left(\frac{\boldsymbol{\mathrm{k}}^{\mathrm{3}} +\boldsymbol{\mathrm{k}}^{\mathrm{2}} +\boldsymbol{\mathrm{k}}+\mathrm{1}}{\mathrm{7}^{\boldsymbol{\mathrm{k}}} }\right)=? \\ $$

Question Number 80131 Answers: 0 Comments: 2

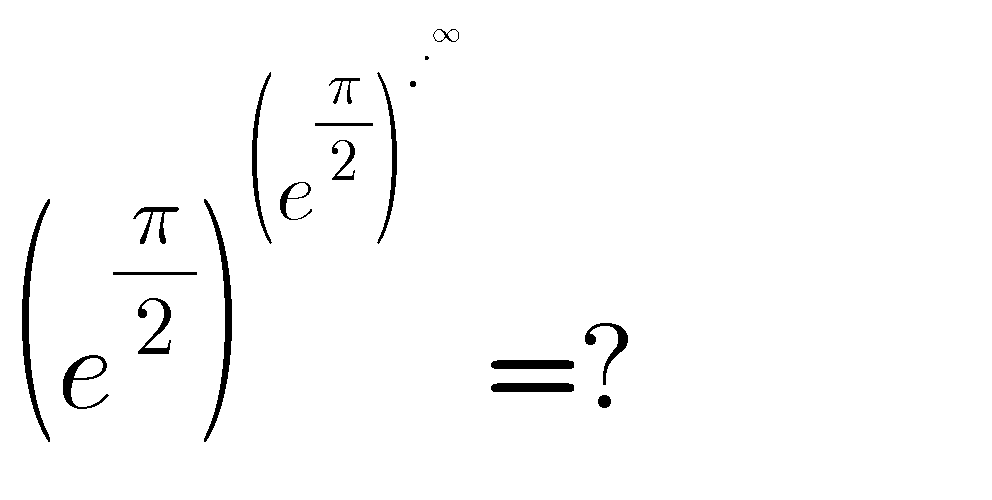
Question Number 80145 Answers: 1 Comments: 5

{ (((x/a)+(y/b)=a^2 +b^2 )),(( [a,b∈R])),((ab(x^2 −y^2 )=xy(a^2 −b^2 ))) :}

$$\begin{cases}{\frac{\boldsymbol{\mathrm{x}}}{\boldsymbol{\mathrm{a}}}+\frac{\boldsymbol{\mathrm{y}}}{\boldsymbol{\mathrm{b}}}=\boldsymbol{\mathrm{a}}^{\mathrm{2}} +\boldsymbol{\mathrm{b}}^{\mathrm{2}} }\\{\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left[\boldsymbol{\mathrm{a}},\boldsymbol{\mathrm{b}}\in\boldsymbol{\mathrm{R}}\right]}\\{\boldsymbol{\mathrm{ab}}\left(\boldsymbol{\mathrm{x}}^{\mathrm{2}} −\boldsymbol{\mathrm{y}}^{\mathrm{2}} \right)=\boldsymbol{\mathrm{xy}}\left(\boldsymbol{\mathrm{a}}^{\mathrm{2}} −\boldsymbol{\mathrm{b}}^{\mathrm{2}} \right)}\end{cases} \\ $$

Question Number 80119 Answers: 2 Comments: 0

Pg 1320 Pg 1321 Pg 1322 Pg 1323 Pg 1324 Pg 1325 Pg 1326 Pg 1327 Pg 1328 Pg 1329

Contact: info@tinkutara.com