
AllQuestion and Answers: Page 1323
Question Number 80503 Answers: 1 Comments: 0
Question Number 80504 Answers: 0 Comments: 2
Question Number 80501 Answers: 0 Comments: 0
Question Number 80455 Answers: 1 Comments: 2
Question Number 80452 Answers: 0 Comments: 1
Question Number 80451 Answers: 0 Comments: 1
Question Number 80448 Answers: 1 Comments: 3
Question Number 80442 Answers: 1 Comments: 4

Question Number 80433 Answers: 1 Comments: 6
Question Number 80432 Answers: 0 Comments: 7
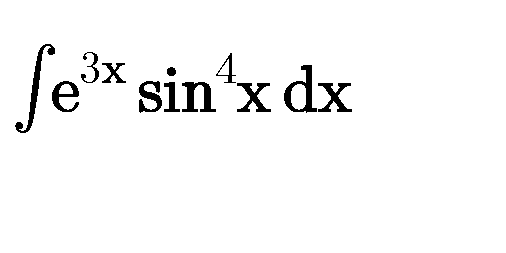
Question Number 80417 Answers: 0 Comments: 5
Question Number 80416 Answers: 1 Comments: 0
Question Number 80405 Answers: 1 Comments: 11
Question Number 80404 Answers: 1 Comments: 0

Question Number 80402 Answers: 0 Comments: 0
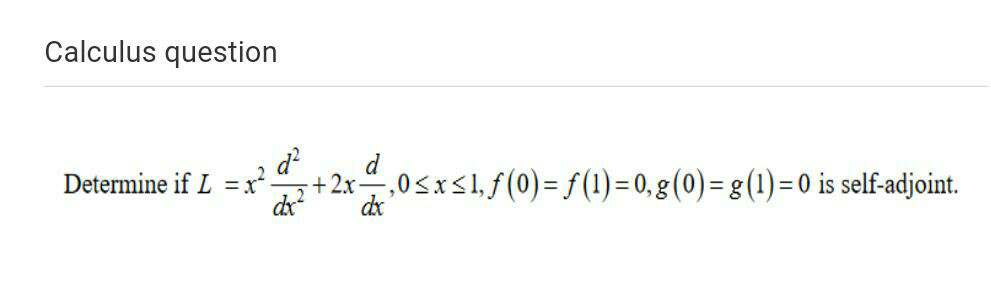
Question Number 80397 Answers: 0 Comments: 3
Question Number 80386 Answers: 1 Comments: 2
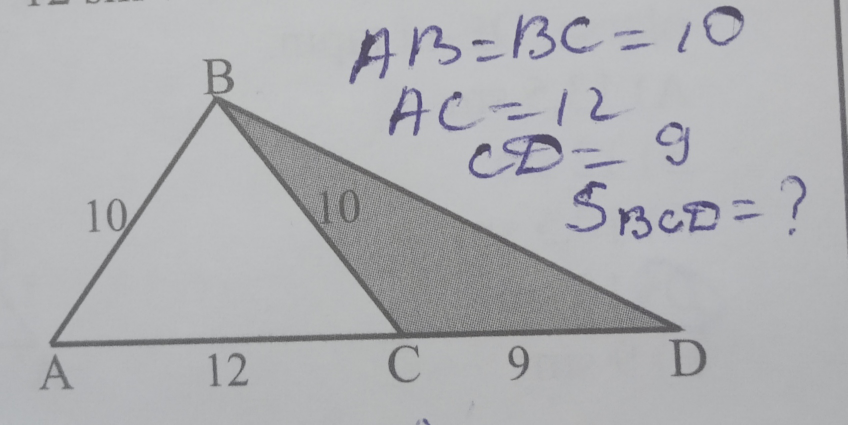
Question Number 80376 Answers: 1 Comments: 2

Question Number 80374 Answers: 0 Comments: 5

Question Number 80369 Answers: 0 Comments: 1

Question Number 80365 Answers: 1 Comments: 6

Question Number 80362 Answers: 1 Comments: 0
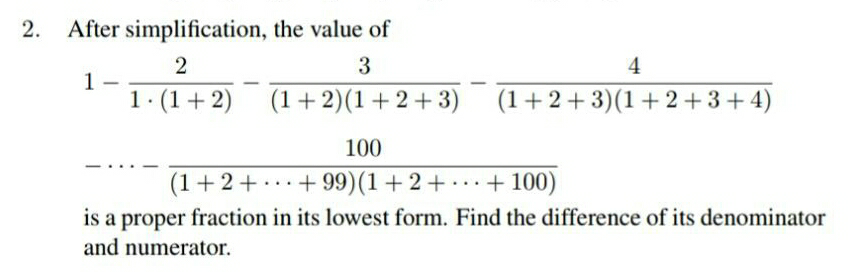
Question Number 80341 Answers: 1 Comments: 0
Question Number 80340 Answers: 1 Comments: 0
Question Number 80334 Answers: 0 Comments: 1
Question Number 80332 Answers: 0 Comments: 1
Pg 1318 Pg 1319 Pg 1320 Pg 1321 Pg 1322 Pg 1323 Pg 1324 Pg 1325 Pg 1326 Pg 1327
